I was wondering about $\mathcal{C}^{\infty}$ immersions $S^1 \longrightarrow \mathbb{R}^2$ which are the restriction to $\partial \mathbb{D}$ of an immersion $\overline{\mathbb{D}} \longrightarrow \mathbb{R}^2$ ? Especially, is a computable invariant for such immersed closed curves ?
$\begingroup$
$\endgroup$
2
-
3$\begingroup$ The paper "Extensions of codimension one immersions" by C. Pappas (1996) TAMS covers your question, although I suspect your question was answered earlier than that. The Pappas paper may give the appropriate reference. $\endgroup$– Ryan BudneyCommented Sep 18, 2014 at 14:13
-
3$\begingroup$ See this answer: mathoverflow.net/a/68021/1345 $\endgroup$– Ian AgolCommented Sep 18, 2014 at 23:14
Add a comment
|
1 Answer
$\begingroup$
$\endgroup$
1
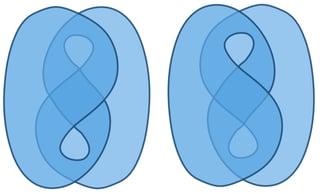
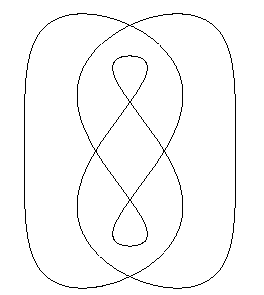
Here is a cool example (due I believe independently to Eliashberg, Milnor, and
Blank) related to your question. There is an immersion of $S^1$ into $\mathbb{R}^2$ that extends to two different immersions of $D^2$ into $\mathbb{R}^2$. Best done with a picture but I
don't know how to up load one. A reference is page 150 of "Topology of Spaces of S-Immersions" by Eliashberg and Mishachev. Glueing these two together gives rise to a map from $S^2$ to $\mathbb{R}^2$ with only fold singularities which is not homotopic through such maps to the standard quish the 2-sphere onto the plane, which is the topic of the E and M paper.
answered Sep 18, 2014 at 16:35
-
$\begingroup$ Thanks Ian! Now can you prove your true powers by making the two different immersions evident ;-). $\endgroup$ Commented Sep 23, 2014 at 19:40