In general, one extracts a manifold invariant from a TQFT by interpreting the closed manifold as a bordism from the empty set to the empty set. The TQFT sends this bordism to a homomorphism of the ground field, which is a number. Such invariants are always multiplicative under disjoint union, this is a consequence of the TQFT being a monoidal functor: $$\mathcal{Z}(M_1 \sqcup M_2) = \mathcal{Z}(M_1) \otimes \mathcal{Z}(M_2) = \mathcal{Z}(M_1) \cdot \mathcal{Z}(M_2)$$
Some TQFTs, like the Crane-Yetter invariant (but not, say, the Turaev-Viro model) give manifold invariants that are multiplicative under connected sum $\#$.
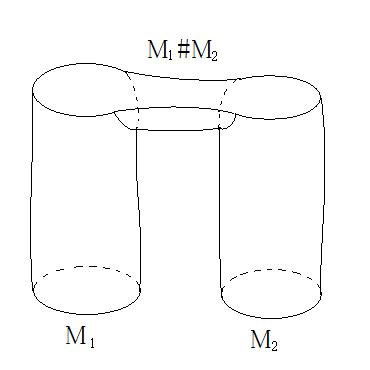
One way to see this is to notice that they can be defined (for connected manifolds) with Kirby calculus: Given a manifold, choose a handle decomposition and consider its link diagram. The diagram is then labelled with morphisms from a ribbon fusion category and the whole diagram is evaluated as a morphism from the monoidal identity to itself, again a number. Now the evaluation of the disjoint union of two link diagrams must then give the product of the evaluations of the respective diagrams, since a ribbon fusion category is monoidal. But the disjoint union of two link diagrams of manifolds $M_1$ and $M_2$ is the link diagram of the connected sum $M_1 \# M_2$!
Which leads me to believe that this multiplicativity secretly comes from monoidality of some functor again. Is there a category of bordisms where the monoidal product of morphisms (=bordisms) is the connected sum, and not the disjoint union? Are such TQFTs actually monoidal functors from this bordism category to $\mathrm{Vect}$?
A related, noncategorical question was asked here: Monoid structure of oriented manifolds with connect sum