Cubic graphs whose 2-factors all have the same cycle type

Question

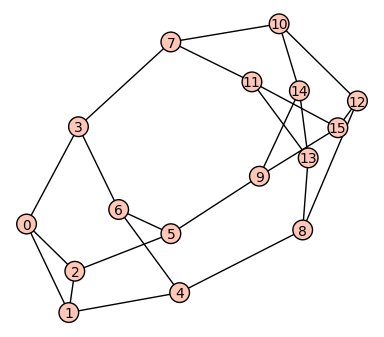
Let $G$ be a bridgeless cubic graph. I am interested in such graphs where all 2-factors are isomorphic (as graphs), i.e. have the same partition as cycle type. We'll say that this partition is realized by the graph.
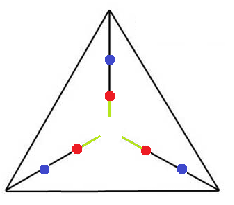
Apart from the trivial $K_4$ and $K_{3,3}$, an example is the Petersen graph realizing $(5,5)$, as all of its 2-factors are of type $(5,5)$. We can easily derive from it a 2-connected graph with a unique 2-factor, of type $(12, 5,5)$, by taking two copies and adding the blue vertices and edges.

It is in fact straightforward to see here that both "inner pentagrams" must belong to any 2-factor.

In view of this kind of construction, it doesn't seem of high interest to ask which partitions can be realized by 2-connected graphs. But it is much more interesting to look at 3-connected graphs.
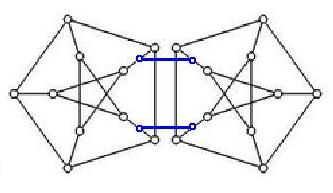
It is not at all obvious for instance that there are 3-connected graphs which have Hamilton cycles as their only 2-factors. The following construction gives one for $n=26$:

In the Petersen graph $P$, there are triples of edges with pairwise distance $3$. If we add vertices $a,b,c$ on each of the edges of such a triple (see picture), now take a second instance of this graph and add three edges $aa',bb',cc'$, we'll come up with a 3-connected graph $G$ whose only 2-factors are H-cycles, i.e. it realizes the partition $(26)$. This is because $a,b,c$ cannot be all contained in a 2-factor of $P$, so there must be two of $aa',bb',cc'$ contained in any 2-factor of $G$, and from there it is easy to check that such a 2-factor cannot contain a $C_5$ of $P$, i.e. it must pass through all vertices.
EDIT: We can realize other types $(n)$ by replacing $P'$ by certain other graphs:

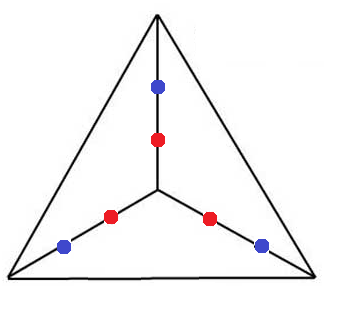
• $n=14$ by just adding one vertex at which $a',b',c'$ concur.
• $n=16$ by using instead a $K_3$ with vertices $a',b',c'$
• $n=20$ by using a $K_4$ with $a',b',c'$ added on the edges of one of its triangles
• ${n=22}$ by using a $K_{3,3}$ with $a',b',c'$ added on the edges of one of its 1-factors [edited again - this one isn't hamiltonian, but has all its 2-factors of type $(17,5)$]
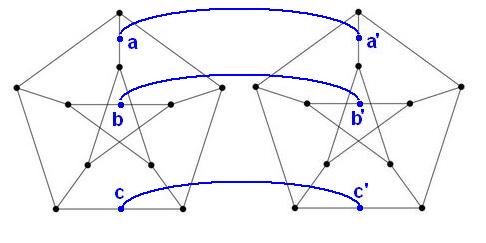
• for $n=36$ we can realize type $(n)$ as follows: start with two copies $P$ and $P'$ as above, join the three outgoing edges of $P$ (call them "blue edges") to the blue vertices and the three outgoing edges of $P'$ (call them "red edges") to the red vertices of this graph:
  
  As above, any 2-factor of $G$ must contain exactly two of the red edges, and it is clear that there cannot be a smaller cycle in it, by checking all paths between two red vertices. On the other hand, several H-cycles obviously exist.
• Replacing the central vertex by a small triangle doesn't change anything in terms of 2-factors, so the new graph realizes the partition (38). In fact, it looks like many (or, to be cautious, let's say several) graphs can be inscribed here instead, as long as they have a H-path between some two of the three 'corners', and as no other path exists between any two 'corners' such that the (graph induced on the) remaining vertices have a 2-factor. Believe it or not... inserting another Petersen graph here (with the three outgoing edges as above) would do $\implies$ a graph for $ n=48$ !

The general question:

• Which partitions (= types) can be realized by 3-connected graphs?
• Are there constructions that yield infinite families of such graphs (e.g. Hamiltonian graphs like the above one) ?

Answer 1

If you replace a vertex of the Petersen graph with a $K_{2,3}$ in the obvious fashion, then this gives a graph on 14 vertices with 12 two-factors all of type $(5,9)$.

Also there's one on 16 vertices obtained from Petersen by a different vertex-replacement.

I'm sure you can find more similar ones.

Answer 2

The examples given above already contain all the ingredients for a construction that yields indeed infinitely many graphs whose only 2-factors are H-cycles.

Start with an arbitrary rooted binary tree. We'll think from the leaves to the root.

• Replace each leaf by the above graph $P$, leaving three "outgoing" edges.

• Replace each node by the graph $T$ shown below, which accommodates a red and a blue triple of "incoming" edges, one for each branch/leaf, and has three "outgoing" edges (in green).
  

• Finally, replace the root by one of the four small graphs as above ($K_1,K_3,K_4$ or $P$, maybe some others are possible, too), accommodating the three "incoming" edges.

At each stage, two of the incoming red edges must belong to a 2-factor, and the same argument as for $n=36$ applies to show that the cycle (which must be, a forteriori, the same cycle as the one containing two of the blue incoming edges) must "continue beyond $T$". If the tree has $k$ leaves, this will result in a graph realizing $(n)$, where $n=13+(13+9)(k-1)+r=22k-9+r$ with $r\in\{1,3,7,13\}$ depending on the small graph chosen for the root.