4
$\begingroup$

Let $C$ be a right circular cone, the convex hull of a unit-radius disk and a point directly above the disk center at height $h$.

               

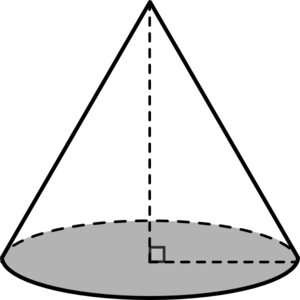
Is the most dense packing of $\mathbb{R}^3$ achieved by translates of $C$ and its negative,* $-C$, i.e., upside-down $C$? Or is it known that rotations of the cones permit achieving a denser packing?

* Barany, Imre, and Jiri Matousek. "Packing cones and their negatives in space." Discrete & Computational Geometry 38.2 (2007): 177-187. (Springer link)

Here is TMA's idea:
            PackingCones
(27Jun14). Conjecture by Wlodek Kuperberg (see the comments), the expert on the topic:

"For a generic cone, the densest packing by translates of the cone and its point-reflection is perhaps the best one."

Now marked as an open problem.

Improve this question
$\endgroup$
8
  • 1
    $\begingroup$ I see it as dependent on the ratio radius/height. For example, pack 6 cones point to point inside a cube. $\endgroup$
    – The Masked Avenger
    Commented Jun 14, 2014 at 2:10
  • 1
    $\begingroup$ Direct link to the article on the website of one of the authors: renyi.hu/~barany/cikkek/kuper.pdf $\endgroup$
    – K. Henriksen
    Commented Jun 14, 2014 at 3:26
  • 1
    $\begingroup$ If one does a "penny packing" of cones (bases in a hexagonal lattice), with a negative on top to fill space, I get a density of (2/3)(pi/2sqrt(3)) or pi/sqrt(27) density, regardless of h. So there is an open interval around 1 of values of r/h in which cube-packing gives a higher density. There may also be a good packing based on a tesellation by octahedra and tetrahedra, but I am not seeing it yet. $\endgroup$
    – The Masked Avenger
    Commented Jun 14, 2014 at 15:36
  • 1
    $\begingroup$ @TheMaskedAvenger The "penny packing" has substantial room for local improvement, since the opposing layers don't touch. $\endgroup$
    – S. Carnahan ♦
    Commented Jun 15, 2014 at 0:12
  • 2
    $\begingroup$ The best packing density of a cone most likely depends on its shape, that is, the ratio between its height and the diameter of its base. For a generic cone, the densest packing by translates of the cone and its point-reflection is perhaps the best one - this is a conjecture. Here is a relevant reference: Bezdek, A., Kuperberg, W.: Dense packing of space with various convex solids, L. Fejes Toth Special Volume, Renyi Inst., Hungary, 2010; arxiv.org/pdf/1008.2398.pdf $\endgroup$
    – Wlodek Kuperberg
    Commented Jun 15, 2014 at 1:52

0

Reset to default

You must log in to answer this question.