Knight's tours in higher dimensions

Question

I wonder if Knight's Tours have been explored in higher dimensions, using the following definition of a knight move.

• In dimension $d=2$, the knight moves left/right and forward/back one step and two steps in either order. So $\{ \pm 1, \pm 2 \}$ steps. This leads to $8$ moves.

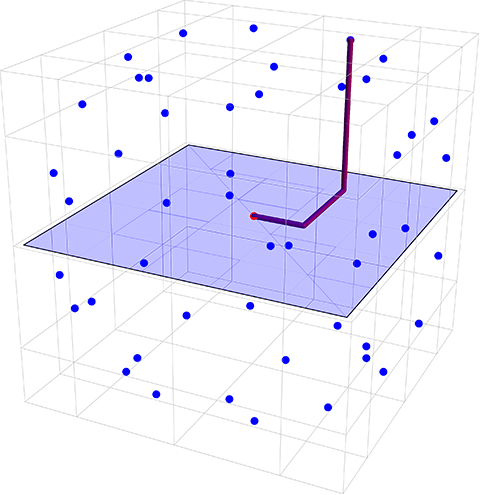
• In dimension $d=3$, the knight moves $\{ \pm 1, \pm 2, \pm 3 \}$ steps in any order along $x,y,z$ axes. This leads to $48$ moves; see below.

• In dimension $d$, the knight moves $\{ \pm 1, \pm 2, \ldots \pm d \}$ steps in any order along the axes. This leads to $d!\, 2^d$ moves.

I have found literature on knight's tours in 3D but under a different (planar) definition of move.¹

It would be interesting to learn which (if any!) $n \times n \times n$ cube chess boards allow a knight's tour.

¹ Awani Kumar. "Magic Knight's Tours in Higher Dimensions." (arXiv link)

---

**Addendum**. *BenBarber* found much more relevant references for planar knight moves in higher dimensions: "with $n$ even, there is always a knight's tour provided that $n$ is sufficiently large." *TMA* made an incisive observation that answered my explicit question: There is no knight's tour for $d=3,4$, due to parity considerations. So, what remains is: (a) $d \ge 5$ and other odd triangular numbers, or (b) knight's tours of the even-coordinate-sum ("black") cells in $d=3,4$.

Answer 1

In my recent preprint, available on arXiv, I show that a more natural $d$-dimensional generalization of the planar knight move rule can be deduced from Article 3.6 of Section E of the well-known FIDE hanbook (see FIDE Handbook).
Basically, since the aforementioned Article 3.6 refers to the Euclidean distance between the starting cell and the ending cell of a knight jump, we can take as a rule that this Euclidean distance has to be fixed at any jump and it is clearly equal to $\sqrt{5}$ chessboard units. Then, the arising diophantine equation admits only two kinds of solutions for any $d \geq 5$, one describing the well-known L-shaped move (whose taxicab length is $3$) and one changing by one $5$ out of the $d$ Cartesian coordinates of the starting cell.

As a result, by Theorem 4.1, we have that closed (Euclidean) knight's tours esist also on any $2 \times 2 \times \cdots \times 2 \subseteq \mathbb{Z}^d$ chessboard, as long as $d > 6$ is given.

Theorem 2.1 constructively proves the existence of open Euclidean knight's tours on a $3 \times 3 \times 3 \times 3 \times 3 \subseteq \mathbb{Z}^5$ chessboard, while there cannot be any closed Euclidean knight's tour on $3 \times 3 \times \cdots \times 3$ chessboards due to the parity argument.

Now, if we still wish to extend the knight move rule by following the pattern $\{\pm 1, \pm 2, \pm 3, \dots, \pm d\}$ instead of the more reasonable $\{\pm 2^0, \pm 2^1, \pm 2^2, \dots, \pm 2^d\}$, we should consider that this violates the light-cell/dark-cell rule so that such a knight would jump from a light-cell to another light-cell even on three dimensional chessboard (in 2006, Qing&Watkins clearly explained this fact in their paper entitled Knigh's Tours for Cubes and Boxes, p. 46). Figure 2 of my preprint shows how to properly color a $k$-dimensional chessboard according to the (widely accepted) parity argument and consequently the $1,2,3,\dots,d$ pattern is not coeherent with the light-square/dark-square jumping principle.

I honestly do not thing that this is a minor issue for the given problem and my proposal of considering the $\sqrt{5}$ constraint in order to extend the knight move rule from $d=2$ to $d=3$ and above could open a new perspective on this topic, avoiding to guess the continuation of a short integer sequence as $1, 2, \dots, ?$.