i have this theorem 

in the paper they gives an example:
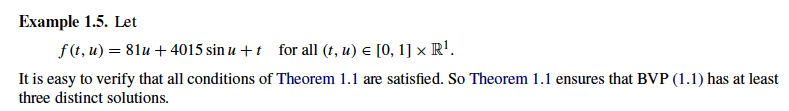
but here $H_1$ is not satisfied !
How to correct it please?
i have this theorem 

in the paper they gives an example:
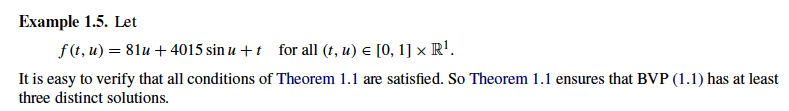
but here $H_1$ is not satisfied !
How to correct it please?
http://www.academia.edu/4591584/Multiple_solutions_of_some_nonlinear_fourth-order_beam_equations
I think you can change the example to $f(t,u)=81u+4015\sin u+tu$. Then H1 holds, while for H2, the integral is $$F(t,u)=({81+t\over 2})u^2+4015(1-\cos u)$$ and so is bounded as indicated with $\alpha={81+1\over 2}=41<\pi^4/2$ and $\beta=2\cdot 4015$. Finally, $$f'_u(t,u)=81+4015\cos u+t$$ so $f'_u(t,0)=4096+t$, for which $m=2$ gives the desired inequality in H3. At least if I got all the details right.
Whether or not this is what was intended, or if the modified example is of any relevance, is a different matter.