A colleague asked me a topology question which comes down to this: Suppose that $M$ is a smooth $n$-manifold, and $C\subset M$ is a closed set such that $H_{n-p}(M-C)\to H_{n-p}(M)$ is not surjective. What can you say about $C$? Clearly in some sense it is at least $p$-dimensional, but in what sense? For example, must it have a subset homeomorphic to $\mathbb R^p$?
-
$\begingroup$ That's what the topological duality theorems are about. $\endgroup$– Włodzimierz HolsztyńskiCommented Apr 18, 2014 at 0:55
-
$\begingroup$ What are the topological duality theorems? $\endgroup$– Tom GoodwillieCommented Apr 18, 2014 at 1:48
-
$\begingroup$ First there was the absolute (just for the spaces, not for subsets) Poincare duality theorem. Then there was Alexander-Pontryagin theorem for subsets of a manifold. On the later occasion Pontryagin introduced his duality theorem for topological groups--initially it was about compact abelian groups versus discrete abelian groups. (This was generalized to locallyt compact abelian groups Egbert van Kampen in 1935 and André Weil in 1940--see wikipedia). An early result about dissecting $\mathbb R^n$ by a compact subset was obtained by Karol Borsuk. Etc. (you need to ask not me but a specialist). $\endgroup$– Włodzimierz HolsztyńskiCommented Apr 18, 2014 at 4:26
-
$\begingroup$ Certainly some kind of $p$th Cech cohomology of $C$ is nontrivial. My question is, what does this imply geometrically about $C$? $\endgroup$– Tom GoodwillieCommented Apr 18, 2014 at 12:23
1 Answer
For sure you can not expect a subset homeomorphic to $\mathbb R^p$.
Say, take $p=1$ and $M=\mathbb T^2$. Note that there is an open embedding of cylinder $f\colon (0,1)\times \mathbb S^1\to\mathbb T^2$ such that the complement $\Sigma=\mathbb T^2 \backslash \mathrm{Im}f$ is a pseudo-circle. In particular $\Sigma$ does not contain a subset homeomorphic to $\mathbb R$. Clearly $$\mathbb Z=H_{1}(\mathbb T^2-\Sigma)\to H_{1}(\mathbb T^2)=\mathbb Z^2$$ is not surjective.
P.S. The pseudo-circle can be constructed as an intersection of nested crooked chains of dics. Here is the image of a chain crooked in a circular chain with 6 links from here; it gives the second iteration in the construction.
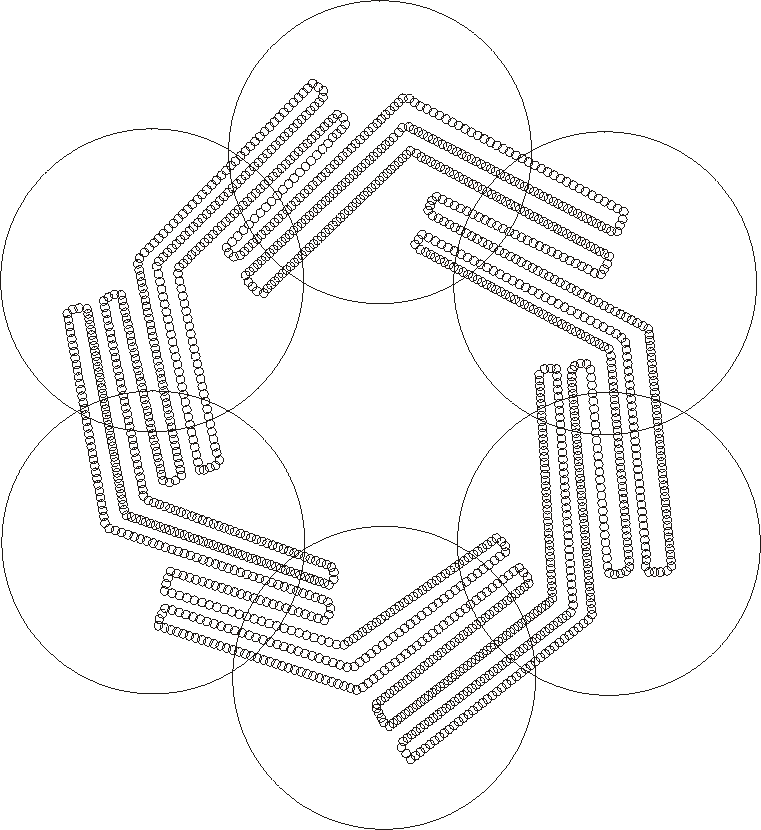
-
$\begingroup$ Why is the complement homeomorphic to a cylinder? $\endgroup$ Commented Apr 18, 2014 at 14:54
-
1$\begingroup$ Thank you, Anton. I did not know that there were such strange examples in such low dimensions. $\endgroup$ Commented Apr 18, 2014 at 16:25
-
$\begingroup$ Anton, what do you mean by statement: For sure you can not expect a subset homeomorphic to $\mathbb R^p$ ? $\endgroup$ Commented Apr 18, 2014 at 18:18
-
1$\begingroup$ @WlodzimierzHolsztynski: search the web on "pseudo-circle" and "continuum". $\endgroup$ Commented Apr 18, 2014 at 19:43
-
1$\begingroup$ I gather, Cech cohomology of the pseudo-circle are the same as for the circle, so by Alexander duality its complement in the plane has two components and has first homology isomorphic to $\mathbb Z$. By the classification of surfaces this means that the complement's components are open disk and open annulus. Joining them by a handle gives the desired embedding into the $2$-torus. The only thing I am not sure about is whether the first Cech cohomology is $\mathbb Z$, but if this weren't true, why would it be called pseudo-circle? $\endgroup$ Commented Apr 18, 2014 at 21:02
