Genus of Tutte-Coxeter Graph

Question

What is the genus of the Tutte-Coxeter graph -- the incidence graph of the GQ of order 2? Seems like it should be well known, since nearly every other parameter for that graph is known, but I can find no reference to cite.

Answer 1

According to sage, the genus is 4

sage: T = graphs.TutteCoxeterGraph()
sage: T.genus()
4

Answer 2

Really just a long comment with some pictures. An embedding onto a genus 4 surface can also be seen directly although in the end we probably need sage to establish the bound is sharp.

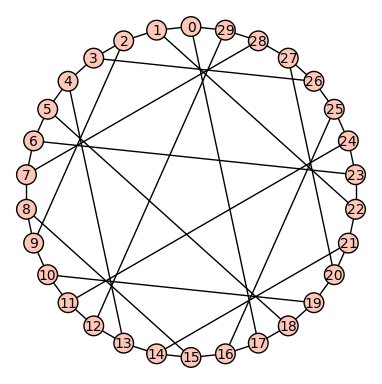
Here is a description of the graph followed by a picture (produced by sage),

{0: [1, 17, 29],1: [0, 2, 22],2: [1, 3, 9],3: [2, 4, 26], 4: [3, 5, 13], 5: [4, 6, 18], 6: [5, 7, 23], 7: [6, 8, 28], 8: [7, 9, 15], 9: [2, 8, 10], 10: [9, 11, 19], 11: [10, 12, 24], 12: [11, 29, 13], 13: [4, 14, 12], 14: [13, 15, 21], 15: [8, 14, 16], 16: [15, 17, 25], 17: [0, 16, 18], 18: [5, 19, 17], 19: [10, 20, 18], 20: [19, 21, 27], 21: [14, 20, 22], 22: [1, 23, 21], 23: [6, 24, 22], 24: [11, 23, 25], 25: [16, 24, 26], 26: [3, 25, 27], 27: [20, 26, 28], 28: [7, 29, 27], 29: [0, 28, 12]}

The edges [j,j+1 mod 30] form a $C_{30}$ subgraph this $C_{30}$ together with the edges $[0,17],[4,13],[20,27]$ and can be embedded in a disk $D_0$ such that the $C_{30}$ is embedded in the boundary of $D_0$. Attach a second disk $D_1$ to the boundary of $D_0$ in this disk embed the edges $[1,22],[5,18],[8,15]$. Likewise, attach disks $D_2$, $D_3$, $D_4$ with edge sets $[2,9],[12,29],[16,25]$; $[3,26],[6,23],[10,19]$; $[7,28],[11,24],[14,21]$. (The groups of 3 represent 3 non-intersecting edges in the graph that are not part of the specified $C_{30}$.)

Next embed this quotient space of the five disks into $\mathbb{R}^3$ and take a regular neighborhood N of this space. (Note we can choose an embedding such that the space has the cross section below.)

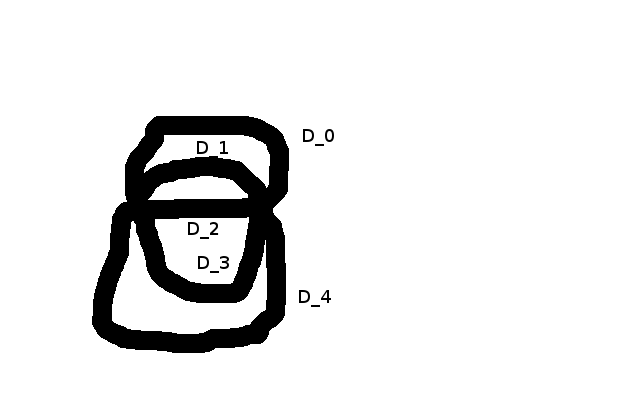
Actually, the graph embeds in the boundary of the neighborhood of this cross section, which is a genus 4 surface.

Furthermore, since the girth of the graph is 8, the Euler characteristic of any surface that this graph embeds into is less than $-2.75$, so by hand we can see the genus is either 3 or 4.