Is there an analog of Sperner's lemma for the Hopf invariant?

Question

Recall that Sperner's lemma is essentially a combinatorial version of the topological statement "A map from $S^n$ to $S^n$ with degree one cannot be nullhomotopic."

My question is, does there exist an analogous combinatorial lemma corresponding to the statement "A map from $S^3$ to $S^2$ with Hopf invariant one cannot be nullhomotopic"?

Answer 1

Sperner's Lemma. Let me start by talking through the implications for topology of Sperner's Lemma. The point is a map $S^1 \rightarrow S^1$ is null-homotopic exactly if it extends to a map $D^1 \rightarrow S^1$. Then the 3 coloring of Sperner's Lemma gives a map from the boundary of the triangulated triangle, to the boundary of a triangle (with the vertices corresponding to the three colors). The condition on the coloring amounts to requiring the map to be degree 1.

Since a map from a simplicial disc to a triangle maps only to its boundary if and only if no 2-simplex maps to the entire triangle, the conclusion follows.

Null-homotopic maps. Now, as Vidit Nanda has noted, a map from $S^3 \rightarrow S^2$ is similarly null-homotopic if and only if it extends to a map $D^4 \rightarrow S^2$. Thus, to show a simplicial map $f$ from the boundary of a triangulated 4-simplex $\Delta$ to the boundary of a tetrahedron is not null-homotopic, it suffices to show that some 3-simplex $\sigma$ in $\Delta$ is "rainbow" -- that is, that the 4 vertices of $\sigma$ map to the 4 vertices of the tetrahedron. (Rainbow being a good word if you think of the 4 vertices as being named Red, Blue, Green, and Yellow.)

The Hopf invariant. At this point, I can answer Jim's question: Yes, there exists a combinatorial analogue of Sperner's Lemma showing that a map with Hopf invariant 1 is not null-homotopic. The definition of simplicial cohomology is purely combinatorial, and the above allows us to translate "null-homotopic" into combinatorics. There surely exists a (possibly complicated) encoding of "Hopf invariant 1" into statements about colors.

Towards a concrete answer According to P Hilton's "An introduction to homotopy theory" Chapter 6, we can define the Hopf invariant for $f:S^3 -> S^2$ as follows: take a point $x$ of $S^2$. The inverse image of $x$ under $f$ is a (homology 1-) cycle $C$, and so there is a homology 2-chain $D$ which has $C$ as its boundary. Since $f$ collapses the boundary of $D$ (and leaves the rest alone), we get that $f(D)$ is a homology 2-cycle in $S^2$. If $f(D)$ is the fundamental cycle, then we say $f$ has Hopf invariant 1.

Thus, a map has Hopf invariant 1 if we can find $D$ and $C$ as above such that the induced map from $D/C \rightarrow S^2$ has degree 1.

Hilton's formulation of the Hopf invariant however does seem to be somewhat more tractable to turning into a combinatorial condition than the standard one from e.g. Wikipedia -- Hilton's is easier to work with simplicially! Incidentally, Hilton seems to say that his formulation is closer than the standard to Hopf's original statements.

Let me try to translate Hopf-invariant-one into a coloring condition:

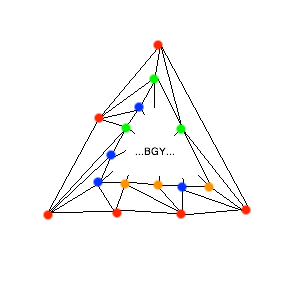
A theorem: Let $f$ be a 4-coloring of the vertices of a triangulated 5-simplex $\Delta$, such that there is a subcomplex $D \subseteq \partial \Delta$ which is a triangulated polygon (i.e., 2-disc) satisfying the following coloring properties:
i) The boundary of $D$ is monochromatic (say, Red),
ii) No interior vertices of $D$ are colored Red, and
iii) The subcomplex of $D$ induced by the interior vertices of $D$ satisfies the Sperner condition on Green, Blue, and Yellow -- that is, there are boundary vertices $v_1, v_2, v_3$ colored Green, Blue, and Yellow, such that the outside path from $v_1$ to $v_2$ has all vertices Green or Blue, etc.
Then $\Delta$ has a rainbow (Red-Green-Blue-Yellow) simplex.

An illustration of the conditions on $D$ follows:

Proof: If there is a rainbow simplex on $\partial \Delta$, then the result is trivial. Otherwise, $f$ induces a map $\partial \Delta \rightarrow S^2$, and the conditions above directly imply that $D/\partial D$ (with the induced coloring) satisfies the conditions of Sperner's Lemma for the boundary of the 2-simplex. We get that the map $f \vert_D$ induces a degree 1 map from $D / \partial D \cong S^2 \rightarrow S^2$, and thus that $f \vert_{\partial \Delta}$ has Hopf invariant 1. Thus, $f \vert_{\partial \Delta}$ is not null-homotopic, and the theorem follows from the discussion above of null-homotopic maps. $\square$

Loose ends The above theorem is a start towards what @JimBelk asked for, but there are several things that are unsatisfactory about it:

1. It is not clear to me that the homology chain $D$ in the definition of the Hopf invariant need be a disc. This would be the main obstacle to proving from the above theorem that a map with Hopf invariant 1 is not null-homotopic.
   (If I understand Hopf's map correctly though, one can indeed take the homology chain to be a disc here. Since this is the only example in these dimensions, requiring $D$ to be a disc might be within the boundaries of good taste.)
2. It would be nice to have a direct combinatorial proof of the above theorem. The main obstacle is seeing how the interior of the simplicial $D^4$ yields an interior of $D / \partial D$. (If you can understand some 3-dimensional interior of $D / \partial D$, then I guess it'll follow from Sperner.)

Answer 2

It seems really hard to impose combinatorial Sperner-like conditions which would guarantee the nontriviality of the Hopf invariant. But if you allow things to get slightly more algebraic by constructing a local system (or a cellular sheaf), then the following statement is a reasonable candidate.

---

Let $K$ be a triangulated $D^4$, i.e., any polyhedral subdivision of the $4$-simplex (with the triangulated $S^3$ in its boundary being denoted $\partial K$), let $L$ be the $3$-simplex on four vertices (which we might label R, G, B, Y). Finally, let $\rho:K \to L$ be any simplicial map so that the following conditions are satisfied:

1. For $\sigma \in L$ of dimension $2$, (i.e., RGB, RGY, RBY, GBY) the corresponding fiber $\rho^{-1}(\sigma)$ restricted to $\partial K$ has the rational homology of a circle.
2. For any face relation $\tau < \sigma$ in $L$ where $\dim \tau = 1$ and $\dim \sigma = 2$, the inclusion $\rho^{-1}(\sigma) \hookrightarrow \rho^{-1}(\tau)$ (again restricted to $\partial K$) induces an isomorphism on rational homology,

Then, there exists a simplex of $K$ which is sent by $\rho$ to the unique $3$-simplex of $L$.

---

From your point of view, the question of central interest might be "what combinatorial restrictions on $\rho$ guarantee 1. and 2. above?". I have no ideas about this yet.