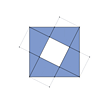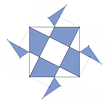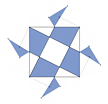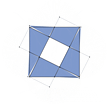
Can you divide one square paper into five equal squares? You have a scissor and glue. You can measure and cut and then attach as well. Only condition is You can't waste any paper.
-
4$\begingroup$ This question is hardly appropriate for MO, despite that AnonymousQQJ's answer is actually informative. I'm voting to close. $\endgroup$– José Figueroa-O'FarrillCommented Feb 13, 2010 at 14:36
-
7$\begingroup$ Jose: it's not immediately obvious that this is possible with finitely many cuts is it? One has to think a little, which, to me, means the question is one of those "here's the question here's the answer now everyone moves on" questions rather than one of those "here's the question but any mathematician who looks at it instantly recognises that it's trivial so it gets closed" questions. $\endgroup$– Kevin BuzzardCommented Feb 13, 2010 at 17:31
-
3$\begingroup$ @Kevin: I agree with your assessment, perhaps I should have written not that the question was inappropriate, just inappropriately posed. I think we ought to discourage questions which are unmotivated or not properly contextualised. This one has attracted some interesting answers, but in general such questions do not. $\endgroup$– José Figueroa-O'FarrillCommented Feb 13, 2010 at 18:31
-
$\begingroup$ Someone on math.stackexchange asked the converse. My answer there (read backwards) gives a concrete answer to this question: math.stackexchange.com/questions/96776/… $\endgroup$– Bill CookCommented Jan 11, 2012 at 0:52
5 Answers
The Wallace-Bolyai-Gerwien Theorem theorem says:
Any two simple polygons of equal area are equidecomposable
(where simple means no self intersections and equidecomposable means finitely cut and glued).
For your problem you can take the first polygon to be a unit square and the second to be a sqrt(5) by 1/sqrt(5) rectangle and apply this theorem. Then perform the remaining four cuts.
Also, the generalisation of your question is the 2d analogue of Hilbert's 3rd Problem which asks whether given any two polyhedra with equal volume can one be finitely cut and glued into the other. The answer here, unlike in the 2d case, is "no" which was proved by Dehn using Dehn invariants in 1900.
-
3$\begingroup$ More information about geometric dissections and equidecomposability can be found in: Boltyanskii, V., Hilbert's Third Problem, Wiley, New York, 1978. Boltyanskii, V., Equivalent and Equidecomposable Figures, D. C. Heath, Boston, 1963. Frederickson, G., Dissections: Plane and Fancy, Cambridge U. Press, New York, 1997. $\endgroup$ Commented Feb 13, 2010 at 14:56
Cut from (0,0) to (1,1/2), and from (0,1/2) to (1,1). We can glue these three pieces together to get a ring with circumference $\sqrt 5$ and height $\sqrt 5 / 5$. Now it's easy!
-
2
-
1$\begingroup$ That is a very simple and elegant solution. $\endgroup$ Commented Feb 13, 2010 at 17:47
Since $1+2i$ has length $\sqrt5$, you can lift a square fundamental domain of $\mathbb C/\mathbb Z[i]$ to $\mathbb C/(1+2i)\mathbb Z[i]$. Overlay a square fundamental domain for the larger torus to get a way to divide a square into 5 smaller squares.
It's pretty easy to decompose any rectangle into a square geometrically, but the general decomposition is not as nice.
-
1$\begingroup$ I think this is the best answer yet. It is basically computation-free! $\endgroup$ Commented Feb 14, 2010 at 6:10
-
2$\begingroup$ With one choice of fundamental domain, it can be the same as TonyK's construction. This shows why that type of construction works for divisions into 2, 4, 5, 8, 9, 10, or 61 squares, since these are norms of Gaussian integers, but doesn't adapt easily to divisions into 3, 6, 7, 11, or 91 squares, although other constructions work then. $\endgroup$ Commented Feb 14, 2010 at 17:15
-
$\begingroup$ Very nice answer! Will have to remember this next time I teach number theory. $\endgroup$ Commented Aug 24, 2010 at 12:49
-
$\begingroup$ This is the same answer as Douglas Zare's. $\endgroup$– Gro-TsenCommented Aug 20 at 21:11
-
1$\begingroup$ It shows a little more, namely a hinged dissection between the two shapes. $\endgroup$ Commented Aug 21 at 11:41
-
$\begingroup$ Beautiful! This would also be a fine answer for proofs without words, mathoverflow.net/questions/8846/proofs-without-words $\endgroup$ Commented Aug 21 at 12:11
The same as other answers, but rendered in terms of origami to define the cut lines.
Label the corners $A,B,C,D$ in rotational order. Then fold and unfold $AB$ onto $DC$ and from this fold identify $A'$ as the midpoint of $BC$, $C'$ as the midpoint of $AD$. Next fold and unfold $BC$ onto $AD$ and from this fold identify $B'$ as the midpoint of $DC$, $D'$ as the midpoint of $AB$.
Now cut along $AA',BB',CC',DD'$ to generate a complete smaller square (originally in the center of the larger square) and eight pieces that are easily paired to get the four remaining smaller squares.
Note that the rotational order may be either clockwise or counterclockwise with equally good validity. Thereby both mirror-image solutions are covered.