Periodic orbits of a billiard ball bouncing in a square have been well-studied.
I am seeking similar analysis of what is sometimes called a rough ball, one
whose high friction causes it to pick up spin when it hits a wall.
Assuming no slip at the point of contact, that kinetic energy is conserved (a "superball"), and
that gravity is not relevant, there is a definite dynamics, dependent upon the
moment of inertia of the ball. For example, a solid ball (e.g., a lacrosse ball) has
moment $I= \alpha m r^2$ where $\alpha=\frac{2}{5}$ (and $m$ and $r$ are mass and radius).
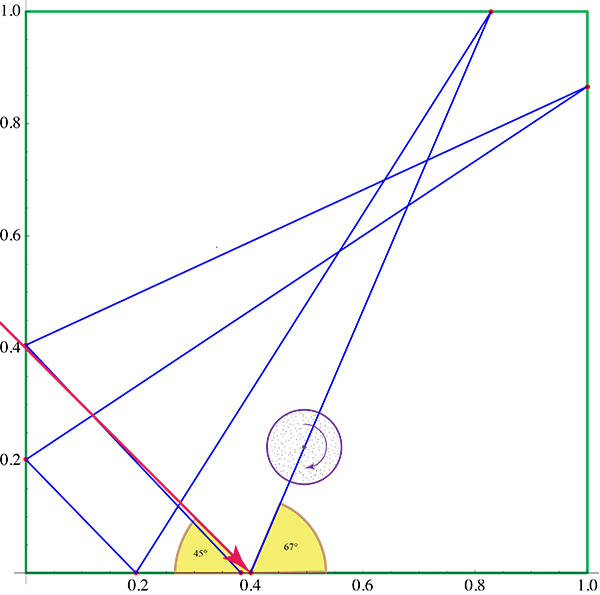
As an example, if such a ball is thrown against the bottom side of a square, entering
(along the red vector) with zero spin at $45^\circ$, horizontal velocity $1$,
it exits at about $68^\circ$, with a clockwise spin
resulting in a horizontal ball-rim velocity of $\frac{-10}{7}$.
I've tried to track above the collision equations, without at all being certain
that I am exactly correct. In my calculation, six bounces almost completes a cycle,
but not quite.
Regardless of the accuracy of these calculations, my question is whether or not periodic orbits of such rough, elastic balls have been explored. Thanks!
(Added 10 Dec 2013.) Just found this paper by Aston & Shail: "The dynamics of a bouncing superball with spin." Dynamical Systems: An International Journal, Volume 22, Issue 3, 2007. Journal link.
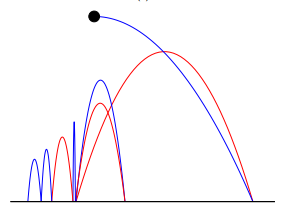
Blue trajectories have back-spin, red forward-spin. Obviously including dissipation. One cool aspect of this paper is that they analyze a ball bouncing up and down a staircase:
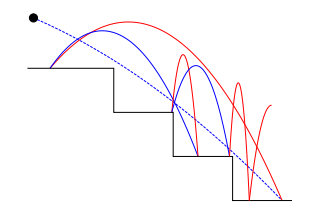
The dashed line is the initial "throw" that initiates the illustrated sequence of bounces.
