How to extract the divergent part from the singular integral

Question

How to extract the divergent part of the following integral simply as $u \rightarrow \infty$

$$g(u) = \frac{\sqrt{2u}}{\pi} \int^1_{\frac{1}{u}} dz \frac{\sqrt{z-1}}{\sqrt{z^2-u^{-2}}} $$

Answer 1

it's an elliptic integral; a series expansion gives

$$g(u)=\frac{\sqrt{2u}}{\pi} \int^1_{1/u} dz \frac{\sqrt{z-1}}{\sqrt{z^2-u^{-2}}}=i\frac{1}{\pi}(2u)^{1/2}\;[\ln (8u)-2]+{\cal O}(u^{-1/2})$$

so the integral diverges as $\sqrt{u}\ln u$

here is a plot of $-i(\pi/\sqrt{2u})g(u)$, evaluated numerically, and $\ln(8u)-2$ versus $u$, just as a check:

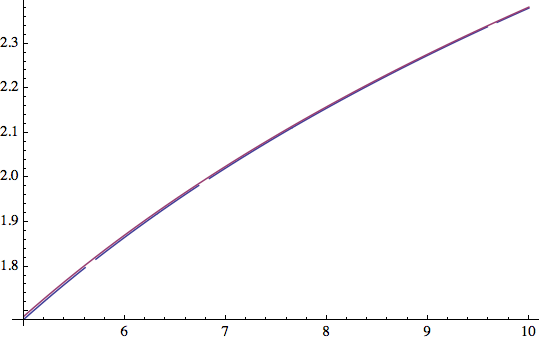
quite a fast convergence

Answer 2

$\newcommand{\+}{^{\dagger}}% \newcommand{\angles}[1]{\left\langle #1 \right\rangle}% \newcommand{\braces}[1]{\left\lbrace #1 \right\rbrace}% \newcommand{\bracks}[1]{\left\lbrack #1 \right\rbrack}% \newcommand{\ceil}[1]{\,\left\lceil #1 \right\rceil\,}% \newcommand{\dd}{{\rm d}}% \newcommand{\down}{\downarrow}% \newcommand{\ds}[1]{\displaystyle{#1}}% \newcommand{\equalby}[1]{{#1 \atop {= \atop \vphantom{\huge A}}}}% \newcommand{\expo}[1]{\,{\rm e}^{#1}\,}% \newcommand{\fermi}{\,{\rm f}}% \newcommand{\floor}[1]{\,\left\lfloor #1 \right\rfloor\,}% \newcommand{\half}{{1 \over 2}}% \newcommand{\ic}{{\rm i}}% \newcommand{\iff}{\Longleftrightarrow} \newcommand{\imp}{\Longrightarrow}% \newcommand{\isdiv}{\,\left.\right\vert\,}% \newcommand{\ket}[1]{\left\vert #1\right\rangle}% \newcommand{\ol}[1]{\overline{#1}}% \newcommand{\pars}[1]{\left( #1 \right)}% \newcommand{\partiald}[3][]{\frac{\partial^{#1} #2}{\partial #3^{#1}}} \newcommand{\pp}{{\cal P}}% \newcommand{\root}[2][]{\,\sqrt[#1]{\,#2\,}\,}% \newcommand{\sech}{\,{\rm sech}}% \newcommand{\sgn}{\,{\rm sgn}}% \newcommand{\totald}[3][]{\frac{{\rm d}^{#1} #2}{{\rm d} #3^{#1}}} \newcommand{\ul}[1]{\underline{#1}}% \newcommand{\verts}[1]{\left\vert\, #1 \,\right\vert}$ $$ {\rm g}\pars{u} = {\root{2u} \over \pi}\int_{1/u}^{1}{\root{z - 1} \over \sqrt{z^{2} - u^{-2}}}\,\dd z = {\root{2u} \over \pi}\int_{1/u}^{1}\root{z - 1}\varphi'\pars{z}\,\dd z $$ where $\ds{\varphi\pars{z} \equiv \int_{1/u}^{z}{\dd t \over \root{t^{2} - u^{-2}}}}$

Then, \begin{align} {\rm g}\pars{u} &= -\,{\root{2} \over 2\pi}\,u^{1/2}\int_{1/u}^{1}\, {\varphi\pars{z} \over \root{z - 1}}\,\dd z \end{align} With the change of variables $\ds{t \equiv u^{-1}\sec\pars{x}}$: \begin{align} \varphi\pars{z} &=\int_{0}^{\arccos\pars{u^{-1}z^{-1}}}\sec\pars{x}\,\dd x =\left.\ln\pars{\sec\pars{x} + \tan\pars{x}}\right\vert_{0}^{\arccos\pars{u^{-1}z^{-1}}} \\[3mm]&=\left.\ln\pars{ut + \root{u^{2}t^{2} - 1}}\right\vert_{u^{-1}}^{z} \\[3mm]&=\ln\pars{uz + \root{u^{2}z^{2} - 1}} =\ln\pars{u} + \ln\pars{z + \root{z^{2} - u^{-2}}} \end{align}

The 'leading term' when $u \gg 1$ becomes: $$ {\rm g}\pars{u}\sim -\,{\root{2} \over 2\pi}\,u^{1/2}\ln\pars{u} \int_{u^{-1}}^{1}{\dd z \over \root{z - 1}} = {\root{2} \over \pi}\,u^{1/2}\ln\pars{u}\root{u^{-1} - 1} $$

Answer 3

The Maple command $$series(sqrt(z-1)/sqrt(z^2-1/u^2), z = 1/u, 2) assuming u>0$$ produces $$ 1/2\,\sqrt {-{\frac {-1+u}{u}}}\sqrt {2}\sqrt {u}{\frac {1}{\sqrt {z-{ u}^{-1}}}}+ \left( -1/8\,\sqrt {-{\frac {-1+u}{u}}}\sqrt {2}{u}^{3/2}- 1/4\,\sqrt {-{\frac {-1+u}{u}}}\sqrt {2}{u}^{3/2} \left( -1+u \right) ^{-1} \right) \sqrt {z-{u}^{-1}}+O \left( \left( z-{u}^{-1} \right) ^ {3/2} \right) .$$