Convex deltahedra in higher dimensions

Question

There are eight convex polyhedra whose faces are equilateral triangles, so-called deltahedra:
   
   _{(Image from here)}

Q. Have the equivalent higher-dimensional analogs been enumerated?

These would be convex polytopes in $\mathbb{R}^d$ all of whose facets are regular $(d{-}1)$-simplices, with no two adjacent simplices coplanar, i.e., lying in the same $d{-}1$ flat.

Answer 1

Yes, but similar to the classification of regular solids there are few such polytopes as the dimension gets high enough. There are 5 four dimensional deltatopes and only 3 for each higher dimension (the simplex, the cross-polytope, and the bipyramid over the lower dimensional simplex). This is proved in Sullivan's (unpublished) preprint "Convex Deltatopes in all Dimensions and Polyhedral Soap Films" (available here).

---

Abstract added by J.O'Rourke:
 

Answer 2

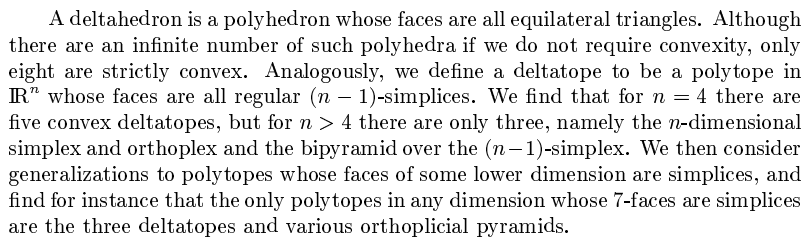
Well, the paper of Sullivan is still unpuplished. Moreover it is from Oct. 1994, as he states on the website mentioned in the other reply.

But there is a German couple, Gerd and Roswitha Blind, who published in the span of 2 decades before already a much more encompassing result: the class of polytopes, all facets of which are regular polytopes. They provide an explicite list of all those, except of the enumeration of the very numerous such diminishings of the 600-cell.

Here are their published references (most of those in German):

• "Die konvexen Polytope im R^4, bei denen alle Facetten reguläre Tetraeder sind", G. Blind and R. Blind, Mh. Math 89 (1980) 87-93, Springer-Verlag (already submitted July 1979)
• "Konvexe Polytope mit kongruenten regulären (n-1)-Seiten im R^n (n>=4)", R. Blind, Comment. Math. Helvetici 54 (1979) 304-308, Birkhäuser Verlag Basel
• "Über die Symmetriegruppen von regulärseitigen Polytopen", G. Blind and R. Blind, Mh. Math. 108 (1989) 103-114, Springer-Verlag
• "The semiregular polytopes", G. Blind and R. Blind, Comment. Math. Helvetici 66 (1991) 150-154, Birkhäuser Verlag Basel

--- rk