It follows from a general theorem of Honda that the formal group with the logarithm $$ x+x^{2^s}/2+x^{3^s}/3+x^{4^s}/4+\cdots $$ has integer coefficients. I became interested in it because its $p$-typizations give the formal groups of the $s$th Morava K-theories (after reducing modulo $p$).
In particular I wonder whether the series $$ \sum_{n\geqslant1}\frac{x^{n^2}}n $$ which one obtains for $s=2$ is related in any way to modular forms and elliptic curves.
Does anybody know where to find information about this?
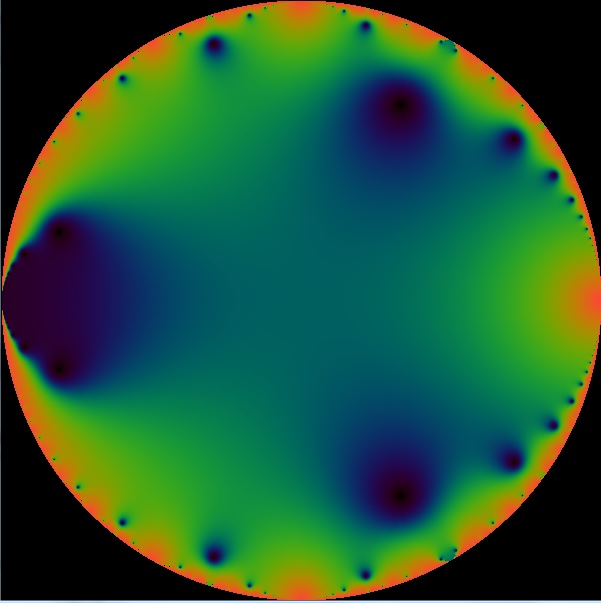
P.S. - Decided to add a picture: here is the color-coded modulus of the derivative of the above series as a function of a complex variable $x$ in the unit disk, where its ``modular-like'' behavior is especially apparent.
P.P.S. - ...and for some further suspense, here are the first few terms of the formal group itself. Notation: $s$ is the sum of the two variables and $p$ is their product. Note the reappearing factors. \begin{align*} s\\ -p&(2s^2-p)\\ +2s^3p&(2s^2-p)\\ -sp&(3s^6-9s^4p+10s^2p^2-3p^3)\\ -s^2p&(2s^2-p)(4s^4+6s^2p-3p^2)\\ +s^4p&(12s^6-21s^4p+20s^2p^2-6p^3)\\ +2sp&(2s^2-p)(4s^8+18s^6p-5s^4p^2-4s^2p^3+p^4)\\ -2s^3p&(18s^{10}+18s^8p-67s^6p^2+87s^4p^3-48s^2p^4+9p^5)\\ -s^2p&(36s^{12}+246s^{10}p+72s^8p^2-493s^6p^3+356s^4p^4-106s^2p^5+12p^6)\\ +3s^9p&(3s^6-9s^4p+10s^2p^2-3p^3)\\ +... \end{align*}
