Width of a random convex polygon

Question

1. Consider a planar (2D) random walk comprised of N steps.

2. Consider the minimum convex polygon enclosing the N points visited by the random walker.

3. Assume the definition of the width of a convex polygon given in http://cgm.cs.mcgill.ca/~orm/width.html

Is it possible to determine the probability density of the width of such a random convex polygon?

Answer 1

(Not an answer; rather an example.)

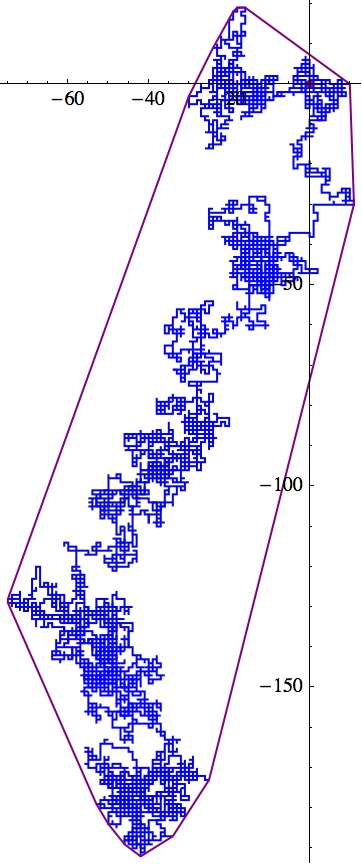
Here is a random walk with random $\pm 1$ $xy$-steps with equal probability (you didn't specify details), for $n=10^4$ steps, and its convex hull.
   
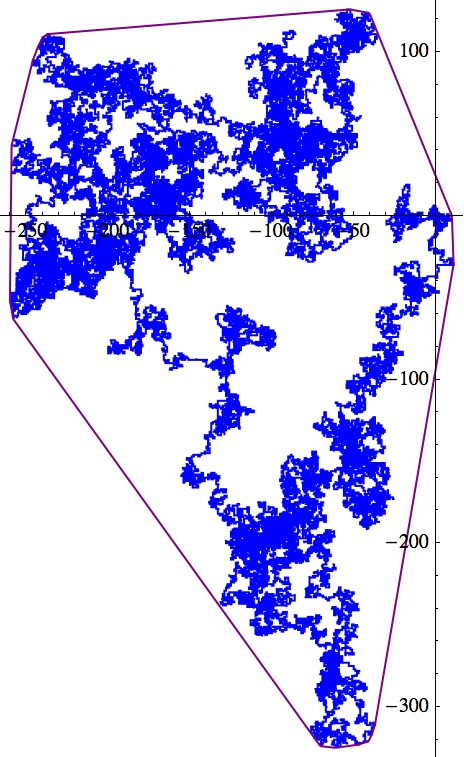
And here is the very same random walk extended to $n=10^5$ steps:
   

---

Added 15Oct15. There is a new paper relevant to this question:

Zakhar Kabluchko, Vladislav Vysotsky, and Dmitry Zaporozhets. "Convex hulls of random walks, hyperplane arrangements, and Weyl chambers." (arXiv abstract.)

"We give an explicit formula for the probability that the convex hull of an $n$-step random walk in $\mathbb{R}^d$ with centrally symmetric density of increments does not contain the origin."

By "contain" here they mean "strictly contain in the interior of the hull."

---

Update 1Apr2017. The same authors have revised their paper, establishing a formula for the expected number of $k$-dimensional faces of the convex hull of a random walk:

Kabluchko, Zakhar, Vladislav Vysotsky, and Dmitry Zaporozhets. "Convex hulls of random walks: Expected number of faces and face probabilities." Revised 21 Aug 2017. (arXiv:1612.00249 Abs.)