Given two convex polytopes $P,Q$ such that $P\subset Q$. We are given that all the vertices of $P$ are also vertices of $Q$ and all the facets defining planes of $Q$ are also facets defining planes of $P$. If $V_P$ is the set of vertices of $P$ and $V_Q$ is the set of vertices of $Q$, we want to estimate $\lvert V_Q\setminus V_P\rvert$ possibly in terms of the dimension of $P$ (or $Q$, since both have the same dimension).
An example when $P\neq Q$:
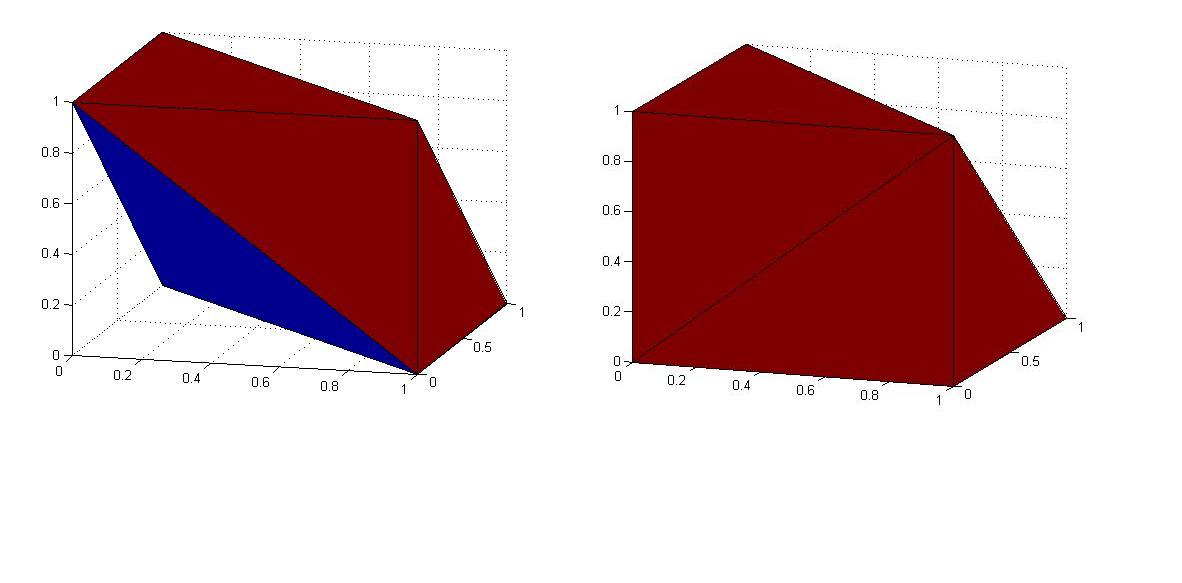
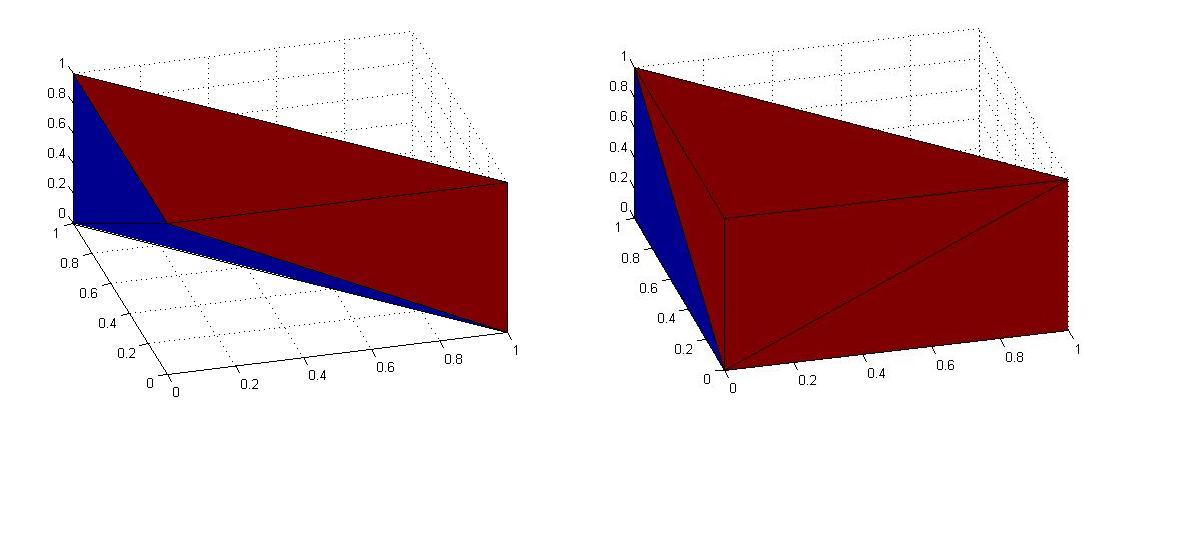
$P=conv([0,0,1],[0,1,0],[1,0,0],[1,1,0],[1,0,1],[0,1,1])$ $Q=conv([0,0,0],[0,0,1],[0,1,0],[1,0,0],[1,1,0],[1,0,1],[0,1,1])$
(Credit to Prof. Shashank K Mehta for constructing the above example)
See the figures below: