I am seeking some assistance on a Mathematics/logic problem which I am having trouble with.
My problem is understanding how a number is calculated in a book called Gödel's Proof (about Kurt Gödel's Incompleteness Theorems)...page 97. Basically, the problem is regards to the (G) formula, whose meta-mathematical statement refers to itself as being not 'demonstrable'.
My problem is that I can't work out how Gödel number (g) could be sub (n, 17, n):
(1) ~ (∃x) Dem (x, Sub (y, 17, y)) Gödel number = n
(G) ~ (∃x) Dem (x, Sub (n, 17, n)) Gödel number (g) = sub (n, 17, n)
(G) is derived from (1), due to specialization of the 'y' variable - replacing y with the Gödel number for (1), which is n.
The sub (n, 17, n) function is really a shorthand for a formula of a formal system (which has the Gödel number 'n'), where any instance of the variable which has the Gödel number '17' (say 'y') is replaced by the Gödel number for 'n' itself.....kind of self-swallowing. I assume that a fellow reader of the book could put it all in context, someone with stronger mathematical/logic ability than my own.
The way I interpret the formula (G) is that it states that (1) is not a theorem (although it is as there world be a number x that fits the criteria), and since (G) is a specialization of (1), I can see how it would be a reference to 'itself', however, I cannot workout how (G)'s Gödel number would be sub (n, 17, n).
I would like to understand more clearly how the number sub (n, 17, n) could be the Gödel number for the formula (G). This is the only difficulty I have with the book..just want to understand the nuts and bolts of (G).
I would be immensely grateful for any help with this and thank you for reading my post.
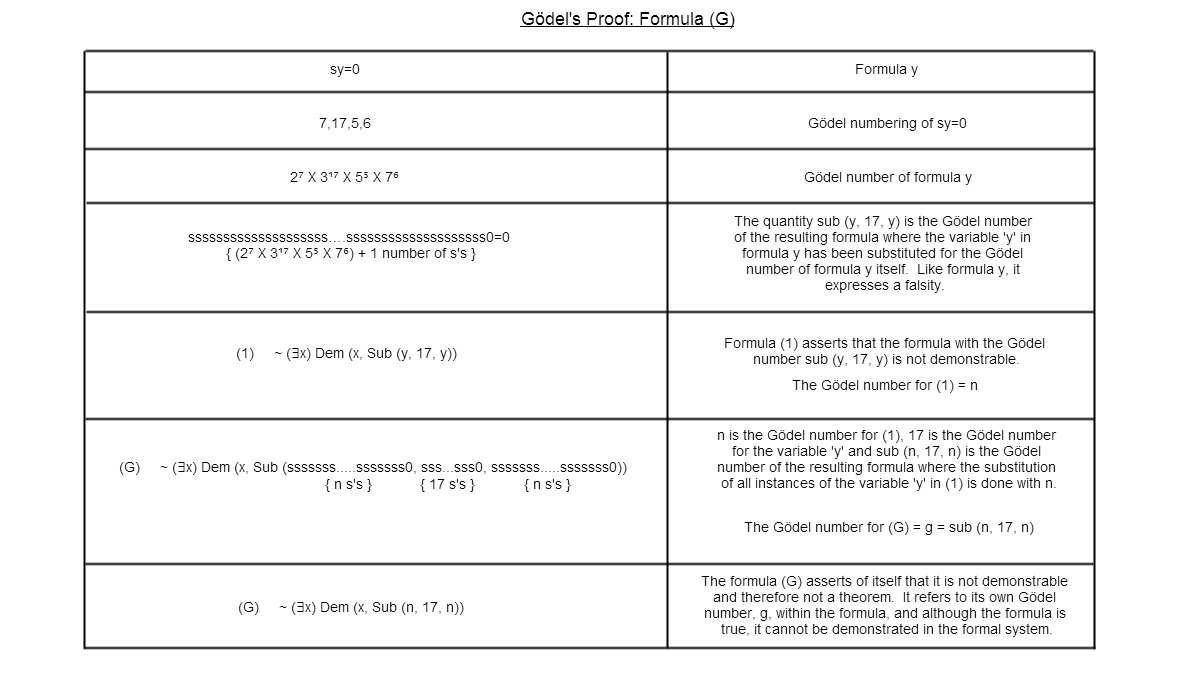
I've drawn up this table to illustrate my interpretation of (G).
