Algebraic geometry predicts a degree 2 branched cover from an elliptic curve to the projective line. What does this map look like topologically?
5 Answers
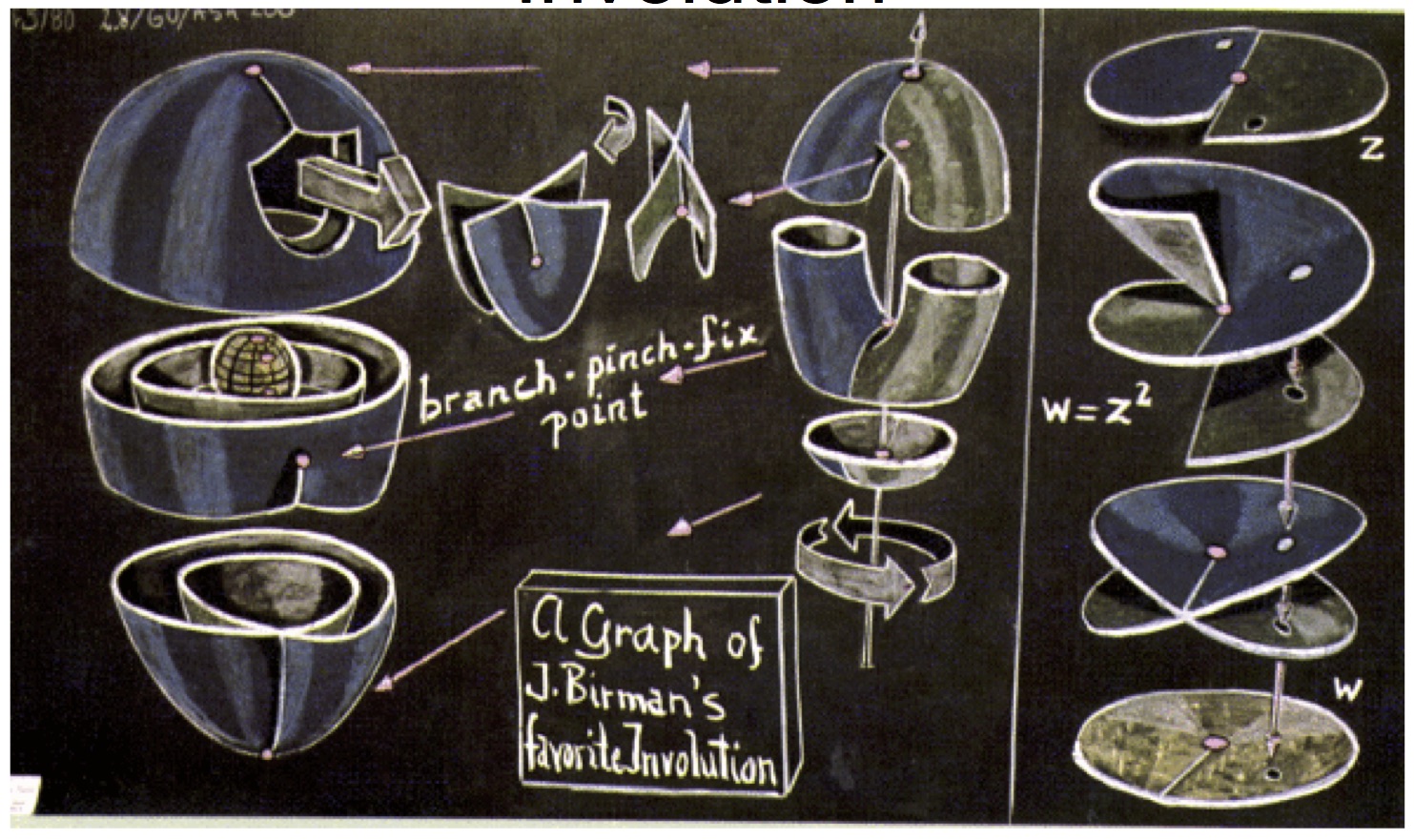
One example: lay your $g$-holed torus $T$ out flat and draw a line the long way through each hole. It hits the torus in $2g + 2$ points. Consider the 180 degree rotation $w$ through that line. Now consider the space $T/w$ formed by identifying two points $P$ and $Q$ if $P = wQ$ (since $w^2 = 1$ we also have $Q = wP$). I claim that it's not too hard to see that $T/w$ is isomorphic to the projective line, and the $2g+2$ points which hit the line are the ramification points.
edit: This is of course more general than you were asking, but the picture is completely general when you're talking about topology.
edit 2: A picture of this (albeit approached from the perspective of starting on the projective line and cutting slits) can be found in section 20e of Fulton's Algebraic Topology book.
If your elliptic curve is $\{(x,y)~|~y^2=x^3 + ax + b\}$ then take the projection $(x,y) \to x$. This has 4 branch points at the 3 roots of $x^3 + ax + b$ and $\infty$. From this perspective, it's easier to see the resulting $\mathbb{CP}^1$, and harder to see that the elliptic curve is a topological torus.
An elliptic curve is an abelian group. The quotient with respect to the equivalence relation $x\sim -x$ is a genus 0 curve. The branched cover is the projection to the quotient and the singular points are the 4 points of order 2 on the curve.
-
-
1$\begingroup$ Anweshi -- applying Riemann-Hurwitz we get the Euler characteristic of the quotient: it is (0-4)/2+4=2 (since the images of all singular points are distinct). $\endgroup$– algoriCommented Feb 3, 2010 at 19:25
-
1$\begingroup$ By the way, the Euler characteristic of the quotient by the hyperelliptic involution described in the stankewicz's answer can also be computed using Riemann-Hurwitz: we get ((2-2g)-(2+2g))/2+2+2g=2. $\endgroup$– algoriCommented Feb 3, 2010 at 19:31
You can do a reverse construction: start with a sphere without 4 points; now add two points over each one in such a way that every time you go around one hole the two points get interchanged.
The same Riemann-Hurwitz calculation guarantees that you get a torus. If you have complex structure on you sphere without 4 points you get one on top as well; a beautiful fact is that you get all complex structures on a torus — in other words, all elliptic curves over $\mathbb C$ — that way.