Surface in 3D that realizes all pairs of principal curvatures

Question

This is a question that Willie Wong raised in comments after he answered my question, Surface analog of clothoid: curvatures covering $\mathbb{R}^3$. Willie's question is more interesting (and challenging) than mine, essentially requiring the curvatures to map onto $\mathbb{R}^2$ rather than only onto $\mathbb{R}$:

Is there a surface $S \subset \mathbb{R}^3$ that realizes all pairs of principal curvatures in the sense that, for every $(\kappa_1,\kappa_2) \in \mathbb{R}^2$, there is a point $p \in S$ such that the principal curvatures at $p$ are $\kappa_1$ and $\kappa_2$?

He also suggested that it might be natural to restrict to $\kappa_1 \ge \kappa_2$.

Answer 1

Here is one view of Manfred's Angel's Curl surface, using $g(u) = e^{u^2}$:
          
Hopefully I've computed this correctly. I've let the parameters $u$ and $v$ range over $\pm 4$.

Answer 2

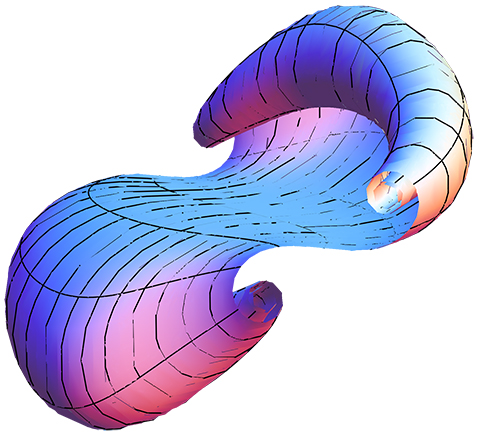
I had yesterday supplied an idea for the construction of such surface as an answer to Surface analog of clothoid: curvatures covering $\mathbb{R}$, however I doubt, whether my construction really generates all pairs of principal curvatures especially the pairs $(\pm\infty,\pm\infty)$, resp. $(\pm\infty,\mp\infty)$, so here is an improvement:

$$ S(u,v)=\left( \begin{array}{c} \int_0^u{sin(s^2)ds} - cos(u^2)*\frac{1}{g(u)}*\int_0^v{cos(t^2)dt}\\\ \int_0^u{cos(s^2)ds} + sin(u^2)*\frac{1}{g(u)}*\int_0^v{cos(t^2)dt}\\\ \frac{1}{g(u)}*\int_0^v{sin(t^2)dt} \end{array}\right)$$ with $g(u) > 0, g''(u) >= 0,$ and $\lim_{u\rightarrow\pm\infty}g(u) = \infty$

Explanation:

the formula without the $\frac{1}{g(u)}$ term resembles my original suggestion of generating $S(u,v)$ by combining two clothoids who's containing planes are mutually perpendicular; the first clothoid (parameterized by $u$), which acts as the master clothoid, is the standard clothoid in the $xy$-plane; the second clothoid (parameterized by $v$) is defined in a local coordinate system with its origin somewhere on the master clothoid, the positive $z$-axis yields the local "$x$-axis" and the curve-normal in that point yields the local "$y$-axis"

The problem with that approach is that, as the second clothoid's origin approaches the limit points of the master clothoid, the limit points of the second clothoid trace out a limit cycle of non-zero radius and thus not both principal curvatures can grow beyond all limits in magnitude.

This shortcoming is addressed by scaling down the second clothoid as it approaches the limit points of the master clothoid; near those limit-points the master clothoid approximately traces out circles, which in turn generates approximately toric surface parts where the second clothoid approaches its limit points. As we have elliptic, cylindric and hyperbolic point on tori, shrinking the second clothoid as it moves along the first one, generates also combinations with arbitrarily large or small principal curvatures.

It remains to check, whether the suggested surface also has points with a pair of arbitrarily small principal curvatures, i.e. pairs of curvatures that are arbitrarily close to $(-\infty,-\infty)$