Assuming the RH and $s \in \mathbb{C}, \rho_n =\frac12 \pm i\gamma_n$, the following (altered) Hadamard product:
$$\displaystyle \displaystyle \prod_{n=1}^\infty \left(1- \frac{s}{\frac12+ (-1)^n i \gamma_n} \right) \left(1- \frac{s}{\frac12+ (-1)^{n+1} i \gamma_n} \right) = \frac{\xi_{rie}(s)}{\xi_{rie}(0)}$$
runs through the alternating non-trivial zeros $\rho_n$ with $\xi_{rie}(s)= \frac12 s(s-1) \pi^{-\frac{s}{2}} \Gamma\left(\frac{s}{2}\right) \zeta(s)$.
Contrary to the factors of the original Hadamard product, these alternating factors do converge.
This question suggest that many similarities exist between infinite (Hadamard/Weierstrass) products using $\gamma_n=n$ and $\gamma_n=\Im(\rho_n)$, and this question shows that a closed form for alternating factors using $\gamma_n=n$ does exist. I therefore like to conjecture that also a closed form exists for the alternating formula above.
Let's call the closed form for each factor $A_-$ and $A_+$ and it is easy to see that:
$$\displaystyle A_-A_+=\frac{\xi_{rie}(s)}{\xi_{rie}(0)}=s(s-1) \pi^{-\frac{s}{2}} \Gamma\left(\frac{s}{2}\right) \zeta(s)$$
is the entire function to be split into two factors.
Splitting this function is easy to do for the Gamma-part: $G_-G_+=s(s-1) \pi^{-\frac{s}{2}} \Gamma\left(\frac{s}{2}\right)$, that for instance (there are more ways) could be factored into:
$$\displaystyle G_-=s\Gamma\left(\frac{s}{4}\right) \pi^{-\frac{s}{4}}2^{\frac{s}{4}-\frac32} \text{ and } G_+=(s-1)\Gamma \left(\frac{s}{4}+\frac12\right) \pi^{-(\frac{s}{4}+\frac12)}2^{\frac{s}{4}+\frac12}$$
But what to do with $\zeta(s)$?
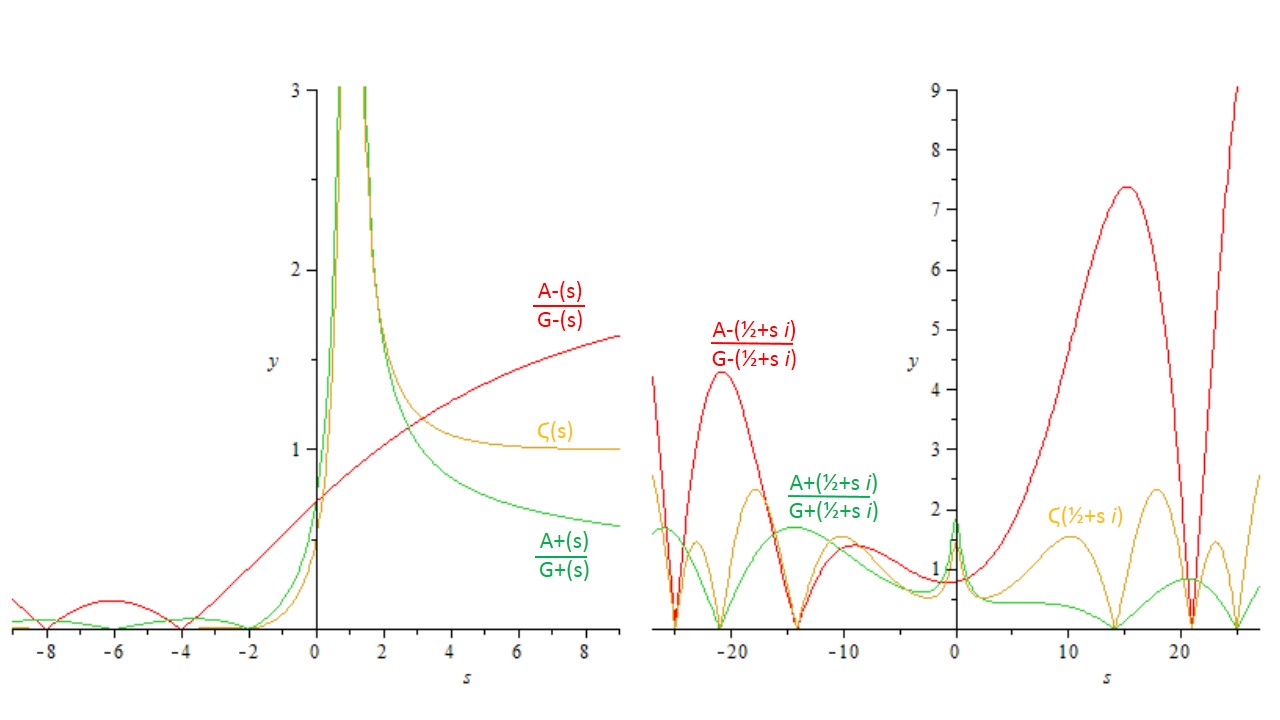
The poles of $G_-$ (-4,-8,...) and $G_+$ (-2,-6,...) might provide some hints, since they need to be annihilated by the zeros of the 'to be found' $\zeta(s)$-factors. It is also clear that a $\zeta(s)$-factor must now induce alternating non-trivial zeros only i.e.: $\frac12+14.134...i,\frac12-21.022...i,\frac12+25.010...i, \dots$ (and its complement). Dividing the infinite product factors $A_-$ en $A_+$ (using n=699) by $G_-$ and $G_+$ respectively, one gets the following graphs of what the (absolute) $\zeta(s)$-factors might look like:
Question (apologies for the long intro):
The graphs of the two potential factors for $\zeta(s)$ above, could both be seen as analytically continued functions across $\mathbb{C}/1$, that have been derived "bottom up" from their alternating zeros. This would imply that in the domain $\Re(s)>1$ the two factors must multiply into:
$$\zeta(s)=\sum_{n=1}^{\infty} \frac{1}{n^s} = \prod _{p \in \mathbb{P}}(1-p^{-s})^{-1}$$
Hence my question: are there any ways to split, the known analytically "discontinued" expressions for $\zeta(s)$ in the domain $\Re(s)>1$, into two factors that each can be analytically continued again?
P.S.:
(1) I have f.i. tried splitting the Euler product into its $(p \mod 4 = 1)$ and $(p \mod 4 = 3)$ factors, however did not see any way to analytically continue these.
(2) Also hoped to find some 'natural' connection with the alternating Zeta: $\eta(s)=\sum_{n=1}^{\infty} \frac{(-1)^{n-1}}{n^s}$ that is valid over the domain $\Re(s)>0$, however so far have been unsuccessful in factoring it further.