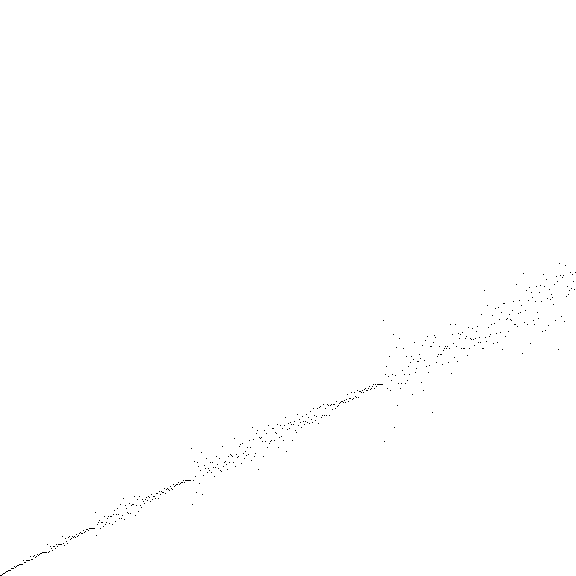
Hofstadter's Q sequence is defined by $Q(1) = Q(2) = 1$ and $Q(n) = Q(n-Q(n-1)) + Q(n-Q(n-2))$ for $n \geq 3$. So far hardly anything on this sequence has been proved -- not even that $Q(n)$ is well-defined for all positive integers $n$.
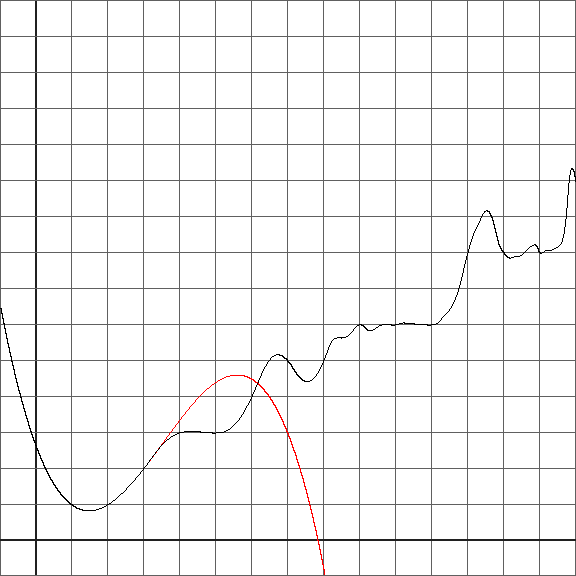
Put $a := \frac{1}{4}(9 - \sqrt{89})$, and let $$ f: \mathbb{R} \rightarrow \mathbb{R}, \ \ x \mapsto \begin{cases} ax^3 + \left(\frac{1}{2} - 6a\right)x^2 + \left(11a-\frac{3}{2}\right)x + 2 - 6a & \text{if} \ x \leq 3, \\\ f(x-f(x-1)) + f(x-f(x-2)) & \text{if} \ x > 3. \\\ \end{cases} $$ Then by choice of the polynomial for $x \leq 3$, the function $f$ is well-defined (note that we have $f(x) > 0$ for any $x \in \mathbb{R}$), continuous and differentiable at all $x \in \mathbb{R}$, and we have $f(n) = Q(n)$ for any positive integer $n$ for which $Q(n)$ is well-defined. Also, by construction for $x \geq 3$ the function~$f$ satisfies the functional equation $f(x) = f(x-f(x-1)) + f(x-f(x-2))$ (but obviously it does not do so for $x < 3$).
Questions:
What can be said about the function $f$ besides these basic facts?
Does the functional equation $f(x) = f(x-f(x-1)) + f(x-f(x-2))$ have continuous and differentiable (or at least continuous) solutions $f: \mathbb{R} \rightarrow \mathbb{R}$ other than the zero function?
Or to be a bit more bold: does it have solutions $f: \mathbb{C} \rightarrow \mathbb{C}$ other than the zero function which are meromorphic on the complex plane?
Remarks: The graph of the function $f$ for $-1 \leq x \leq 15$ looks as follows (the graph of the polynomial used to define $f$ for $x \leq 3$ is shown in red):
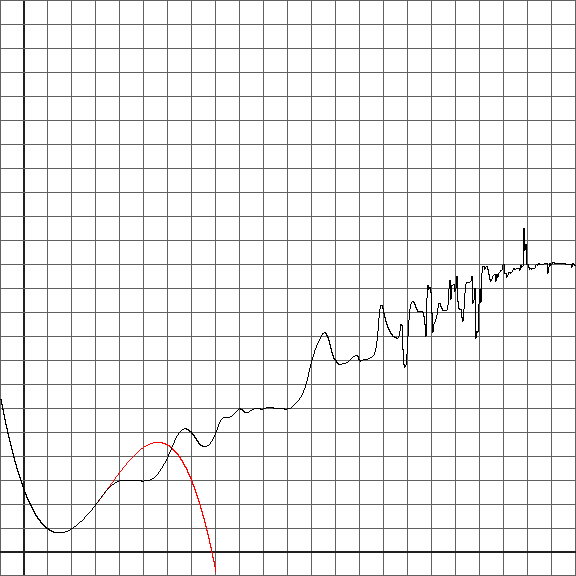
The graph for $-1 \leq x \leq 23$ looks as follows:
A plot of the first 576 terms of Hofstadter's Q sequence looks like this: