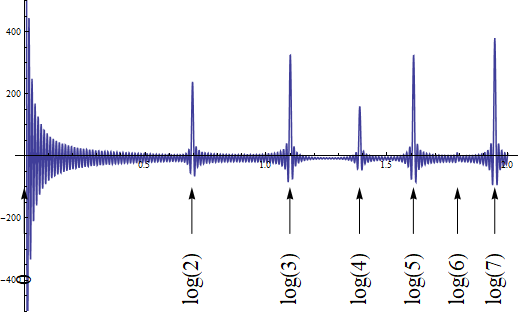
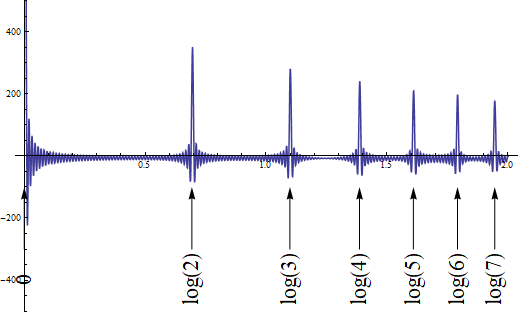
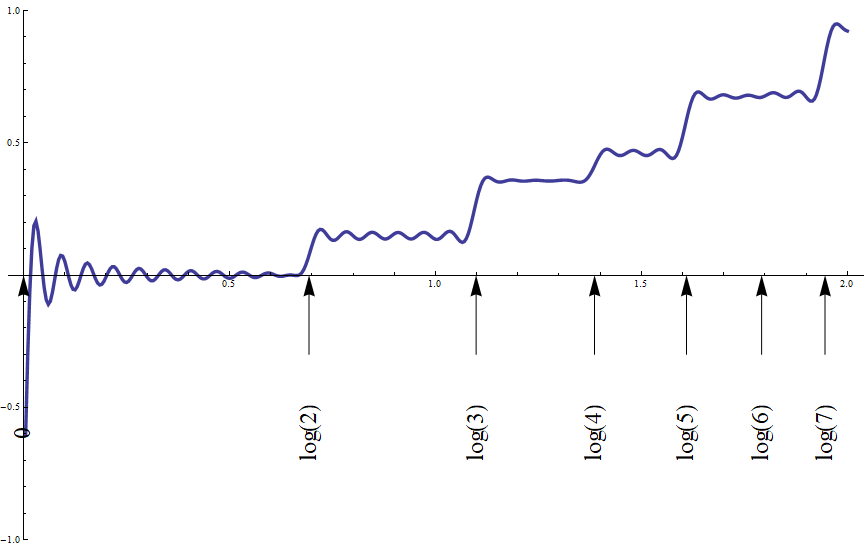
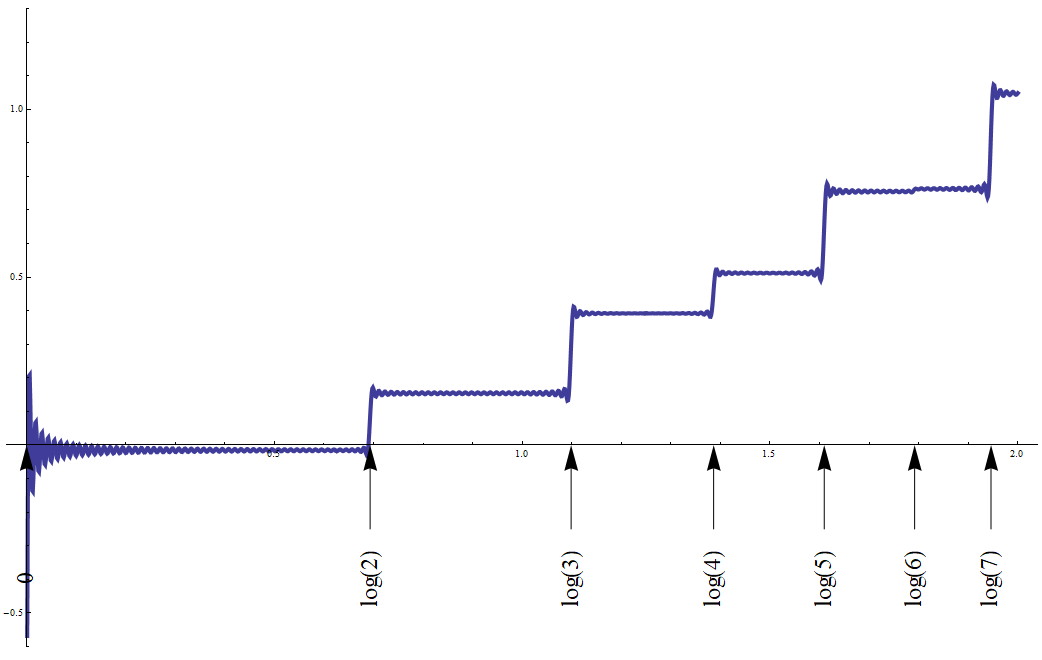
Let $0 < k_1 < k_2 < k_3 < \cdots $ be all the zeros of the Riemann zeta function on the critical line:
$$ \zeta(\frac{1}{2} + i k_j) = 0 $$
Let $f$ be the Fourier transform of the sum of Dirac deltas supported at these points. In other words:
$$ f(x) = \sum_{j = 1}^\infty e^{ik_j x} $$
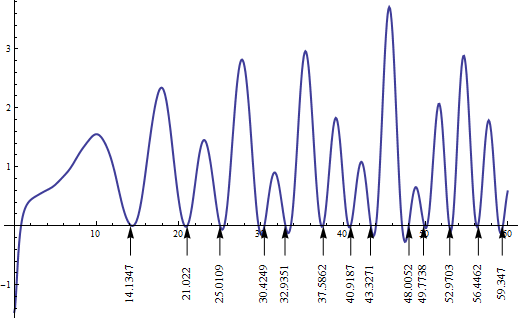
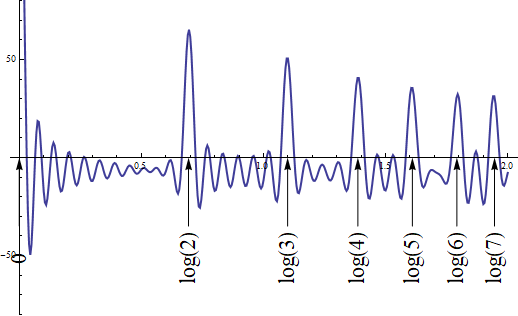
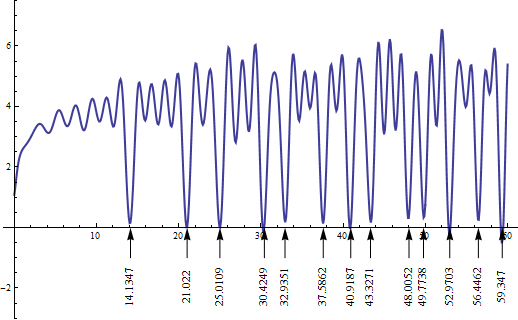
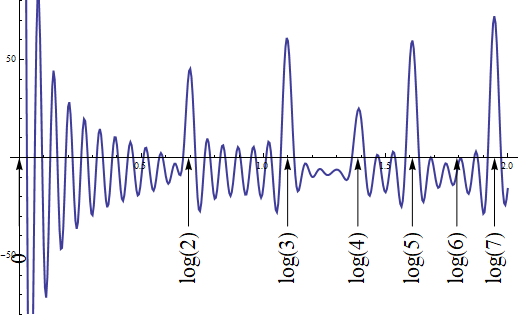
This is not a function, but it's a tempered distribution. Matt McIrvin made a graph of it:
McIrvin seems to get an infinite linear combination of Dirac deltas supported at logarithms of powers of prime numbers. But Freeman Dyson seems to claim that this is only known to be true assuming the Riemann Hypothesis. So, my question is:
1) What do people know about $f$ without assuming the Riemann Hypothesis?
2) What do people know about $f$ assuming the Riemann Hypothesis?
3) Can we prove that $f$ is a linear combination of Dirac deltas supported at prime powers, if we assume the Riemann Hypothesis?
4) Is there some property of $f$, like being a linear combination of Dirac deltas supported at prime powers, that's known to imply the Riemann Hypothesis?
Part of why I'm confused is that J. Main, V. A. Mandelshtam, G. Wunner and H. S. Taylor have a paper containing some equations (equations 8 and 9) that seem to imply
$$ \sum_{j = 1}^\infty \delta(k - k_j) = - \frac{1}{\pi} \sum_{p} \sum_{m = 1}^\infty \frac{\ln p}{p^{m/2}} e^{i k \ln{p^m}} $$
where the sum over $p$ is a sum over primes. This seems to be just what we need to show $f$ is an infinite linear combination of Dirac deltas supported at logarithms of prime powers!
I may be getting some signs and factors of $2 \pi$ wrong, but I don't think that's the main problem: I think the problem is whether a formula resembling the above one is known to be true, or whether it's only known given the Riemann Hypothesis.
Here's what Freeman Dyson said about this in 2009. Unfortunately he omits some details I'm dying to know:
The proof of the Riemann Hypothesis is a worthy goal, and it is not for us to ask whether we can reach it. I will give you some hints describing how it might be achieved. Here I will be giving voice to the mathematician that I was fifty years ago before I became a physicist. I will talk first about the Riemann Hypothesis and then about quasi-crystals.
There were until recently two supreme unsolved problems in the world of pure mathematics, the proof of Fermat's Last Theorem and the proof of the Riemann Hypothesis. Twelve years ago, my Princeton colleague Andrew Wiles polished off Fermat's Last Theorem, and only the Riemann Hypothesis remains. Wiles' proof of the Fermat Theorem was not just a technical stunt. It required the discovery and exploration of a new field of mathematical ideas, far wider and more consequential than the Fermat Theorem itself. It is likely that any proof of the Riemann Hypothesis will likewise lead to a deeper understanding of many diverse areas of mathematics and perhaps of physics too. Riemann's zeta-function, and other zeta-functions similar to it, appear ubiquitously in number theory, in the theory of dynamical systems, in geometry, in function theory, and in physics. The zeta-function stands at a junction where paths lead in many directions. A proof of the hypothesis will illuminate all the connections. Like every serious student of pure mathematics, when I was young I had dreams of proving the Riemann Hypothesis. I had some vague ideas that I thought might lead to a proof. In recent years, after the discovery of quasi-crystals, my ideas became a little less vague. I offer them here for the consideration of any young mathematician who has ambitions to win a Fields Medal.
Quasi-crystals can exist in spaces of one, two, or three dimensions. From the point of view of physics, the three-dimensional quasi-crystals are the most interesting, since they inhabit our three-dimensional world and can be studied experimentally. From the point of view of a mathematician, one-dimensional quasi-crystals are much more interesting than two-dimensional or three-dimensional quasi-crystals because they exist in far greater variety. The mathematical definition of a quasi-crystal is as follows. A quasi-crystal is a distribution of discrete point masses whose Fourier transform is a distribution of discrete point frequencies. Or to say it more briefly, a quasi-crystal is a pure point distribution that has a pure point spectrum. This definition includes as a special case the ordinary crystals, which are periodic distributions with periodic spectra.
Excluding the ordinary crystals, quasi-crystals in three dimensions come in very limited variety, all of them associated with the icosahedral group. The two-dimensional quasicrystals are more numerous, roughly one distinct type associated with each regular polygon in a plane. The two-dimensional quasi-crystal with pentagonal symmetry is the famous Penrose tiling of the plane. Finally, the one-dimensional quasi-crystals have a far richer structure since they are not tied to any rotational symmetries. So far as I know, no complete enumeration of one-dimensional quasi-crystals exists. It is known that a unique quasi-crystal exists corresponding to every Pisot–Vijayaraghavan number or PV number. A PV number is a real algebraic integer, a root of a polynomial equation with integer coefficients, such that all the other roots have absolute value less than one${}^1$. The set of all PV numbers is infinite and has a remarkable topological structure. The set of all one-dimensional quasi-crystals has a structure at least as rich as the set of all PV numbers and probably much richer. We do not know for sure, but it is likely that a huge universe of one-dimensional quasi-crystals not associated with PV numbers is waiting to be discovered.
Here comes the connection of the one-dimensional quasi-crystals with the Riemann hypothesis. If the Riemann hypothesis is true, then the zeros of the zeta-function form a one-dimensional quasi-crystal according to the definition. They constitute a distribution of point masses on a straight line, and their Fourier transform is likewise a distribution of point masses, one at each of the logarithms of ordinary prime numbers and prime-power numbers. My friend Andrew Odlyzko has published a beautiful computer calculation of the Fourier transform of the zeta-function zeros${}^2$. The calculation shows precisely the expected structure of the Fourier transform, with a sharp discontinuity at every logarithm of a prime or prime-power number and nowhere else.
My suggestion is the following. Let us pretend that we do not know that the Riemann Hypothesis is true. Let us tackle the problem from the other end. Let us try to obtain a complete enumeration and classification of one-dimensional quasicrystals. That is to say, we enumerate and classify all point distributions that have a discrete point spectrum...We shall then find the well-known quasi-crystals associated with PV numbers, and also a whole universe of other quasicrystals, known and unknown. Among the multitude of other quasi-crystals we search for one corresponding to the Riemann zeta-function and one corresponding to each of the other zeta-functions that resemble the Riemann zeta-function. Suppose that we find one of the quasi-crystals in our enumeration with properties that identify it with the zeros of the Riemann zeta-function. Then we have proved the Riemann Hypothesis and we can wait for the telephone call announcing the award of the Fields Medal.
These are of course idle dreams. The problem of classifying one-dimensional quasi-crystals is horrendously difficult, probably at least as difficult as the problems that Andrew Wiles took seven years to explore. But if we take a Baconian point of view, the history of mathematics is a history of horrendously difficult problems being solved by young people too ignorant to know that they were impossible. The classification of quasi-crystals is a worthy goal, and might even turn out to be achievable.
[1] M.J. Bertin et al., Pisot and Salem Numbers, Birkhäuser, Boston, 1992.
[2] A.M. Odlyzko, Primes, quantum chaos and computers, in Number Theory: Proceedings of a Symposium, 4 May 1989, Washington, DC, USA (National Research Council, 1990), pp. 35–46.