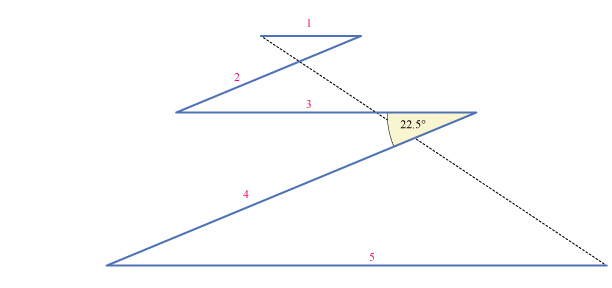
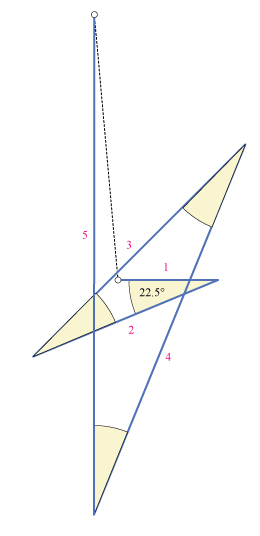
I have posted this question here without answer. Maybe I can get some light here.
Suppose we are given $n$ segments $l_1,...,l_n$ in $\mathbb{R}^2$ such that $|l_i|=i,\ \forall\ i=1,...,n$, where $|l_i|$ is the length of $l_i$. Let $\alpha_1,...,\alpha_{n-1}$ be $n-1$ angles such that $\alpha_i>0$ and $\sum_{i=1}^{n-1}\alpha_i\leq\frac{\pi}{2}$.
Consider the following operation: (All angles are assumed to be "clockwise").
1) Place $l_{1}$ anywhere in $\mathbb{R}^2$,
2) Place $l_{2}$ at either of the end points of $l_{1}$, making an angle of $\alpha_{1}$,
3) Place $l_{3}$ at the end point of $l_{2}$ not occupied by $l_{1}$, making an angle of $\alpha_{2}$,
...
n-1) Place $l_{n-1}$ at the end point of $l_{n-2}$ not occupied by $l_{n-3}$, making an angle of $\alpha_{n-2}$,
n) Place $l_{n}$ at the end point of $l_{n-1}$ not occupied by $l_{n-2}$, making an angle of $\alpha_{n-1}$.
Now consider arbitrary permutations both of the angles $\alpha_i$ and of the lengths $l_i$. Which ordering of the angles and lengths maximizes the distance between the two endpoints of the chain of segments?