48
$\begingroup$

This is a vague question, and I will no doubt be (properly!) chastised for posing it. I would like to generate a set $S$ of points in $\mathbb{R}^3$—$|S|$ finite or infinite—which has the property that, viewing $S$ under orthogonal projection along a random direction $\vec{u}$ results in a more-or-less generic, undistinguished cloud of points. But, there is some specific projection direction $\vec{u^*}$, where suddenly (if one were 3D-rotating the points under mouse control) the cloud resolves itself, through unlikely point alignments, to paint a recognizable image, e.g.,
           
Is this an impossible :-) hope?

Update. Following Michael Murray's recipe, with $10,000$ points within a cube in $\mathbb{R}^3$, three different viewpoints:
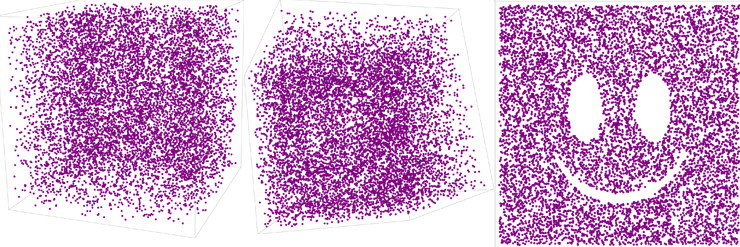
     Smileys: 3 views
(Somehow my analytical smiley has a Halloween evil glint!)

PS(31 Oct 2012). Happy Halloween!
           PumpkinSmiley

Another addition (23Jun2018):

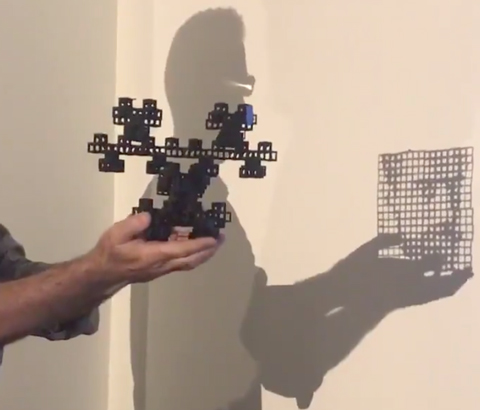
          ShadowFaces
          (Image from John Urschel / MoMath video.)

Improve this question
$\endgroup$
11
  • 3
    $\begingroup$ A questions to see if I understand what you're asking. Suppose you take $S$ to be $\mathbb{Q}^3$ minus the points that are at a distance $\epsilon$ from the $x$-axis. Does this count as a set that is a random cloud in every direction except along the $x$-axis? $\endgroup$
    – Timothy Chow
    Commented Oct 27, 2012 at 1:04
  • 18
    $\begingroup$ You may like these sculptures :-) friki.net/fotos/86210-el-hombre-que-le-gano-al-photoshop.html $\endgroup$
    – Mariano Suárez-Álvarez
    Commented Oct 27, 2012 at 3:32
  • 5
    $\begingroup$ This may be a situation where the artists beat the mathematicians to it. There are several painters and sculptors who create such effects. A particularly striking example is the work of Vesna Kovacic. She constructs three dimensional many-coloured obects with irregular surfaces which form chaotic patterns except when viewed from one particular angle, when they resolve themselves into a simple geometric form . A striking example is her column at the Krankenhaus Weisshorn. Examples of her work can be found at her home page www.vesnakovacic.de or by googling her name under google images. $\endgroup$
    – jbc
    Commented Oct 27, 2012 at 10:29
  • 2
    $\begingroup$ (The middle image is scarier that the third one!) $\endgroup$
    – Mariano Suárez-Álvarez
    Commented Oct 27, 2012 at 23:51
  • 7
    $\begingroup$ @Mariano: That's a Rorschach test, and you just inadvertently revealed ... something about yourself?! :-) $\endgroup$
    – Joseph O'Rourke
    Commented Oct 28, 2012 at 0:46

8 Answers 8

Reset to default
36
$\begingroup$

Surely you can draw the 2-D image in the XY plane so it consists of points of the form (x, y, 0) and then give each point in it a random non-zero Z co-ordinate. So it should look like a mess except viewed looking in along the Z-axis.

Improve this answer
$\endgroup$
2
  • 5
    $\begingroup$ @Michael: My concern is that the halo/shadow of the hidden shape would be discernable from other directions. I realize this need quantification... $\endgroup$
    – Joseph O'Rourke
    Commented Oct 27, 2012 at 1:40
  • 4
    $\begingroup$ It depends on the probability distribution of the random coordinate and whether you can observe the density of points or merely the location. If you observe the density, then the shape twisted by a small angle will be a blur, via convolution with the probability density function, of the original shape. If you observe the location then, as long as the original shape has thickness, it just depends on whether the distribution is bounded or unbounded. $\endgroup$
    – Will Sawin
    Commented Oct 27, 2012 at 4:27
40
$\begingroup$

Glad that MO is up and running again.

Following the suggestion by Michael Murray one can also produce more than just one sudden smiley:

alt text

I guess, that a higher number of images is also possible. But probably some structure may be visible from other directions as well in this case. By the way: The problem seems to be a bit related to tomography...

Improve this answer
$\endgroup$
1
  • $\begingroup$ Ha!!! $\mbox{}$ $\endgroup$
    – Joseph O'Rourke
    Commented Nov 1, 2012 at 11:19
25
$\begingroup$

Perhaps the digital sundial of Falconer is what you need: http://www.researchgate.net/publication/225574085_Digital_sundials_paradoxical_sets_and_vitushkins_conjecture

Here is a photograph of a working model: http://apod.nasa.gov/apod/ap120626.html
     SolsticeDial
     (Image added by O'Rourke)

Improve this answer
$\endgroup$
8
  • 1
    $\begingroup$ I would think Falconer's construction is far too complicated to implement in any realistic setting (even with lots of computing power). Lots of splitting into many pieces and recursively modifying each piece. $\endgroup$
    – Mike Hall
    Commented Oct 27, 2012 at 5:28
  • 4
    $\begingroup$ I also thought so, until I learned about working models, like the one shown in the photo in my answer:-) $\endgroup$
    – Alexandre Eremenko
    Commented Oct 27, 2012 at 14:37
  • $\begingroup$ It seems the second link is broken :( $\endgroup$
    – Alexander Chervov
    Commented Oct 27, 2012 at 17:58
  • 1
    $\begingroup$ The model in the picture is unrelated to Falconer's construction except in spirit. It's a back-solution to getting a desired projection on one day, and a solid shadow on others, which is a much simpler problem (and I don't think would satisfy Joseph O'Rourke, but I could be wrong). $\endgroup$
    – Mike Hall
    Commented Oct 28, 2012 at 0:04
  • 2
    $\begingroup$ I wonder what future archaeologists will make of it. $\endgroup$
    – Douglas Zare
    Commented Oct 28, 2012 at 14:23
16
$\begingroup$

A Painting Made From Pieces of Glass enter image description here

Improve this answer
$\endgroup$
9
$\begingroup$

If you have no desire for compactness, you can choose the set of points to be all of the points in $\mathbb R^3$ which lie in the inverse image of :-) under the orthogonal projection. Then the image under a different orthogonal projection will always be a union of intervals times $\mathbb R$, giving no indication of the shape of the smiley.

On the other hand, if you demand that the points lie in a ball of radius $R$, then if you shift the angle of the projection by $\epsilon$, then you shift the image of each point by $O(\epsilon R)$, so the new image will be very similar: each point of the new image is within $O(\epsilon R)$ of a point of :-), and each point of :-) has a point of the new image within $O(\epsilon R)$ of it. In particular for $\epsilon R$ small enough this will be visually indistinguishable.

Improve this answer
$\endgroup$
2
  • 2
    $\begingroup$ Wouldn't the intervals times $\mathbb{R}$ give a lot of information? If you did this with the complement, you would get the whole plane from any other projection, though. $\endgroup$
    – Douglas Zare
    Commented Oct 27, 2012 at 16:41
  • $\begingroup$ Not really. For instance, if the smiley is surrounded by a circle, you just get the height of that circle in whatever projection. Basically you get all projections of your 2-d figure onto 1-d lines, which conceals a lot about it. $\endgroup$
    – Will Sawin
    Commented Nov 1, 2012 at 19:24
6
$\begingroup$

I would like to see such a subset of $\mathbb{R}^3$ having the property that several of its shadows are recognizable. For example, I would like to see sets of points whose shadows realize the actions of two or more generators in a Cayley graph.
See this question for more details.

Improve this answer
$\endgroup$
3
  • $\begingroup$ Nice question, David! The challenge reminds me of the cover of Winkler's book Mathematical Mind Benders" books.google.com/books/about/… $\endgroup$
    – Joseph O'Rourke
    Commented Oct 28, 2012 at 13:42
  • $\begingroup$ If you just take the intersection of the preimages of projections of several large sets, you get several nice projections. It is more difficult to do this when you want several particular small projections. The smiley is small, but its complement is large, so there should be no problem making a cloud of points which projects to the complement of a smiley in several directions. $\endgroup$
    – Douglas Zare
    Commented Oct 28, 2012 at 14:26
  • 2
    $\begingroup$ And also the cover of Hofstadter's "Gödel, Escher, Bach" (en.wikipedia.org/wiki/Gödel,_Escher,_Bach) $\endgroup$
    – Cristi Stoica
    Commented Nov 2, 2012 at 9:15
5
$\begingroup$

Or the other way, a Sudden 2013 :)

Improve this answer
$\endgroup$
3
  • $\begingroup$ Ha! What a clever idea! $\endgroup$
    – Joseph O'Rourke
    Commented Aug 9, 2013 at 11:39
  • 1
    $\begingroup$ Is this for real? $\endgroup$
    – JRN
    Commented Dec 21, 2013 at 13:51
  • 2
    $\begingroup$ I have serious doubts. But it triggered me a sudden smiley :) $\endgroup$
    – Cristi Stoica
    Commented Dec 21, 2013 at 18:35

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .