Packing and isoperimetrics

Question

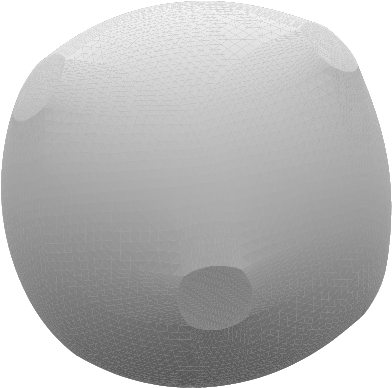
Suppose a manufacturer bottles small units of liquid and ships them via very large trucks.

If the transportation cost nothing, spherical bottles would minimize the packaging cost (isoperimetric inequality); if packaging cost nothing, cubic bottles (say) would minimize the transportation cost, because they would pack on the trucks with no wasted space.

How would the ideal container vary with the relative cost of packaging (measured as the surface area of one bottle) and transportation (measured by the packing density on an infinitely capacious truck)?

[My "applied" formulation notwithstanding, I mean this as a pure mathematics question, so please idealize and ignore any distracting side issues.]

A reasonable conjecture might seem that one gets the correct family of shapes by starting with a tight lattice packing of spheres and then over-inflating and rescaling the spheres until asymptotically they assume the form of Voronoi cells of a lattice packing. So one would get constant mean curvature surfaces up to the circular disks at the interfaces between adjacent spheres. Optimal or not, this would give lower bounds. But how to compute them?

Answer 1

This is the problem of wet foams, soap bubble clusters separated by water. As the liquid fraction goes to zero, you get the dry foam or partitioning problem, optimally the hexagonal honeycomb in 2D (Hales) and conjecturally the Weaire-Phelan structure in 3D or the Kelvin structure if all cells must be congruent. As the liquid fraction increases you pass through the optimal sphere packing (proved by Thue in 2D and Hales in 3D) to spherical bubbles floating in a sea of liquid. The transition in 2D from the hexagonal honeycomb to the circle packing is easy to conjecture but would be very hard to prove. In 3D a similar smooth transition beginning with the Kelvin partition would yield the inefficient BCC lattice sphere packing instead of the optimal FCC lattice sphere packing. A smooth transition beginning with the less efficient rhombic dodecahedra would yield the optimal FCC lattice sphere packing. So the optimal wet foam probably jumps discontinuously from one branch to the other at some point.

Answer 2

You probably already know this, but the problem of dividing 3-dimensional space into equal volumes with minimal interfacial area is called Kelvin's problem. It may be one limit of the problem you're considering.

You can read about the currently best-known solution in 3D on Wikipedia here and there are links to pages with 3D models and data. The Weaire-Phelan structure uses two types of cells though, which I guess would be rather inconvenient from your applied perspective. Kelvin's original conjectured solution uses only one type of cell.

In 2D the minimal perimeter solution is the honeycomb, as bees know and Thomas C. Hales proved.

While googling I found some talk slides by Hales on some recent work on the 3D problem which I found interesting as well.

Answer 3

As an aside, there is a Swedish food packaging company, Tetra Pak, that was built on the idea of packaging milk in tetrahedral containers:
        TetraPak http://upload.wikimedia.org/wikipedia/commons/thumb/b/b1/Tetra_pak_2008.jpg/305px-Tetra_pak_2008.jpg
Practically speaking, there is another criterion that is important: the surface should unfold flat to a polygon that tiles the plane, so that they can be cut out easily. Of course the regular tetrahedron unfolds to an equilateral triangle, but also to a $2 \times 1$ parallelogram; I wouldn't be surprised if Tetra Pak used the latter.

Answer 4

László Fejes Tóth considered the planar version in his book Regular Figures. On page 175, he gives the following theorem, which verifies your guess (discs here does not necessarily mean circular discs):

If P denotes the total perimeter of n convex discs, each of area a, lying in a convex hexagon of area H without mutual overlapping, then $$\frac{P}{H}\ge \sqrt{\frac{n}{H}} p(na/H)\text.$$

The function $p(a')$ is defined as follows: it is equal to the perimeter of a circle of area $a'$ for $a'\le\pi/\sqrt{12}$ and equal to the perimeter of a rounded hexagon of area $a'$ for $\pi/\sqrt{12}<a'\le 1$. A rounded hexagon being a regular hexagon of unit area, whose corners have been rounded to arcs of circles.

If there was no restriction to convex discs, this would easily imply the honeycomb conjecture. Fejes Tóth writes "It may be assumed that this proposition remains valid without the restriction to convex faces. In the case of isoperimetric faces this conjecture turns out to be true, but for faces of equal area its proof seems to involve considerable difficulties". These difficulties were eventually overcome by Thomas Hales, as pointed out by jc.

Regarding your last question "how to compute them", you can use Surface Evolver to simulate a bubble confined to the interior of a rhombic dodecahedron. Here, for example, is the result of a simulation of a bubble confined to a cube:

If you're interested, I can provide the input file I used to do the simulation.