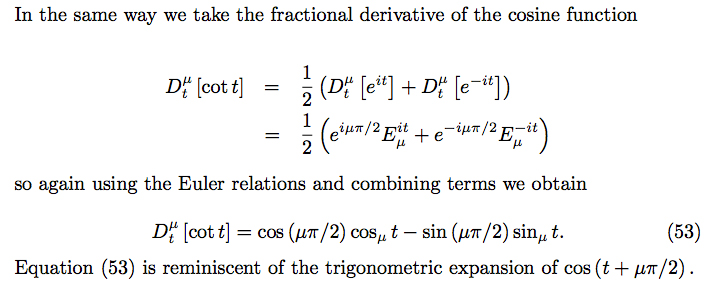
I wonder if there any established formula for fractional derivative of a function $\pi \cot (\pi x)$.
I derived the following expression:
$(\pi \cot (\pi q))^{(p)}=-\frac{\zeta'(p+1,q)+(\psi(-p)+\gamma ) \zeta (p+1,q)}{\Gamma (-p)}-\Gamma (p+1) \zeta (p+1,1-q)$
where $\psi$ is digamma, $\zeta$ is Hurwitz zeta, $\zeta'$ is the derivative by first argument
and I want to compare it with the other expressions, and, possibly, equate them to derive further results.
My derivation is as follows.
First of all there is a known formula that is only valid for integer $n$:
$\psi_n(z)=(-1)^{(n+1)}n!\zeta(n+1,z)$ (can be seen here: http://mathworld.wolfram.com/PolygammaFunction.html, formula 12).
Espinoza and Moll mention in their article on the definition of balanced polygamma that they were unable to find a generalization for this formula despite any attempts.
If to try to directly expand the formula to non-integer values, the resulting function will be non-real. If to replace the $(-1)^n$ with a cosine, the resulting polygamma generalization will be undefined at negative integer values. Red line on this graph:

This is highly undesirable because many formulas for integrals and discrete integrals use negapolygamma at negative integer order. If we take a traditional or balanced polygamma, multiplying it by cosine or another simple fiunction will not give the zeta function. Even more the resulting function will not be discrete-analytic. So this approach is also undesirable.
Following this a thought came to my mind that the alternating sign naturally arises if we differentiate a function of negative argument.
Since $\psi(1-x)=\psi(x) + \pi\cot\pi x$ we can rewrite the previously cited formula in the following form:
$(\psi(x) + \pi\cot\pi x)^{(n)}=-n! \zeta(n+1,1-x)$
By taking different definitions of fractional derivative of cotangent one can arrive at different generalizations of polygamma.
The formula also works in the opposite way: by taking a given generalization of polygamma one can arrive at different expressions for fractional derivative of cotangent.
Indeed,
$(\pi\cot\pi x)^{(n)}=-n! \zeta(n+1,1-x)-\psi^{(n)}(x)$
If we assume the polygamma being the balanced generalization, the formula
$(\pi \cot (\pi q))^{(p)}=-\frac{\zeta'(p+1,q)+(\psi(-p)+\gamma ) \zeta (p+1,q)}{\Gamma (-p)}-\Gamma (p+1) \zeta (p+1,1-q)$
arises.
This gives the formula $(\cot (q))^{(p)}=-\frac{\zeta'(p+1,\frac q\pi)+(\psi(-p)+\gamma ) \zeta (p+1,\frac q\pi)}{\pi^{p+1}\Gamma (-p)}-\frac 1{\pi^{p+1}}\Gamma (p+1) \zeta (p+1,1-\frac q\pi)$ for fractional derivative of cotangent.
Below is the graphic of function $2\cot x \csc x^2$ which is the second derivative of cotangent and the 1.9999th derivative of cotangent following the above formula. The graphs seem to coincide.
I wonder whether there are known other generalizations of fractional derivative of cotangent and which corresponding generalizations of polygamma will arise if to insert those in the previous formula.