Possible locations for non trivial zeroes lying off the critical line

Question

It has been proven that:

1) if $s$ is a non trivial zero $\rho$ of $\zeta(s)$ then so is $1−s$.

2) $\zeta(s) = 2^s \pi^{s-1} \sin(\frac{\pi s}{2}) \Gamma(1-s) \zeta(1-s)$

3) $ 0 < \Re(\rho) <1$

From this it follows that when $s \to \rho$:

$\displaystyle \lim_{s \to \rho} |\dfrac{\zeta(s)}{\zeta(1-s)}| = |2^s \pi^{s-1} \sin(\frac{\pi s}{2}) \Gamma(1-s)|=1$

It is easy to see that the outcome will be $1$ for all $y$ in $s=\frac12 + y i$.

But if a $\rho$ would lie off this critical line, it also must reside in 'spots' where $\displaystyle \lim_{s \to \rho} |\dfrac{\zeta(s)}{\zeta(1-s)}|=1$.

On which points off the critical line could this occur? I found a surprisingly small domain (no proof).

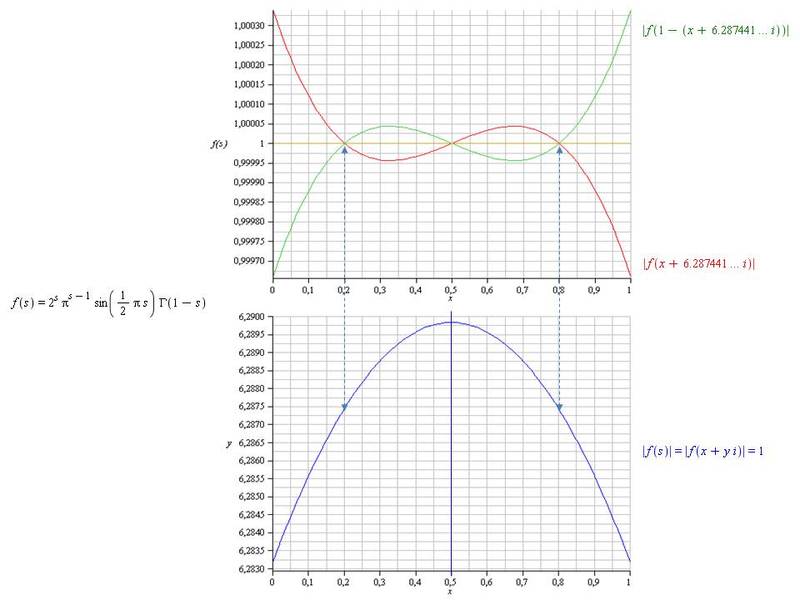
The blue line shows the only values where:

$\displaystyle |2^s \pi^{s-1} \sin(\frac{\pi s}{2}) \Gamma(1-s)|=1$, $s=x + y i$, $ 0 \le x \le 1$.

Note that $y \to 2\pi$ for both $x=0$ and $x=1$. The $y$ rises only a little in the middle.

This doesn't say anything about whether or not off-line $\rho$'s are actually hiding on this curve. There still is an infinite number to check. However, I wondered if anything more is known about this curve?

^{(source: Wayback Machine)}

Answer 1

I believe you're mistaken that $$ \lim_{s\to\rho}\left|\frac{\zeta(s)}{\zeta(1-s)}\right|=1. $$ Write $\zeta(1-s)=\zeta(s)f(s)$ with $f(s)$ as implied by your equation (2). The series expansion for $\zeta(s)$ at $s=\rho$ is $$ \zeta(s)=\zeta^\prime(\rho)(s-\rho)+O(s-\rho)^2. $$ The series expansion for $\zeta(1-s)$ at $s=\rho$ is $$ \zeta(1-s)=\zeta(s)f(s)=\zeta^\prime(\rho)f(\rho)(s-\rho)+O(s-\rho)^2. $$ By standard manipulation of series, $$ \frac{\zeta(s)}{\zeta(1-s)}=\frac{1}{f(\rho)}+O(s-\rho), $$ so the limit should equal $1/f(\rho)$.

Answer 2

There is some mild confusion here. Yes, for $s$ with real part $1/2$ the function $f(s)=2^{s}\pi^{s-1} \sin(\pi s/2)\Gamma(1-s)$ has magnitude $1$, which is an easy consequence of $|\Gamma(1/2+it)|=\sqrt{\frac{\pi}{\cosh {\pi t}}}$, but $|f(s)| \neq 1$ in general since a meromorphic function whose magnitude is constant on any open set is necessarily a constant. The limit $\lim_{s \to \rho} \frac{\zeta(s)}{\zeta(1-s)}$ is $f(\rho)$, but this doesn't put any constraint on $\rho$...

Answer 3

Let $\chi(s)=2^s \pi^{s-1}\sin(\pi s/2) \Gamma(1-s)$ so that $\zeta(s)=\chi(s)\zeta(1-s)$. You are asking about the curve $|\chi(s)|=1$.

As you have observed, $|\chi(1/2+it)|=1$ for real $t$. There is a partial converse to this statement, namely that there is a positive absolute constant $C_0$ such that if $|\chi(\sigma+it)| = 1$ with $0 \le \sigma \le 1$ and $|t| \ge C_0$, then $\sigma=1/2$.

A simple proof can be found in Lemma 6.1 of S. M. Gonek "Finite Euler products and the Riemann hypothesis" Trans. Amer. Math. Soc. 364 (2012), 2157-2191. This paper is also on the arXiv. Gonek states that $C_0<6.3$ so it seems that phenomena in your pictures stops shortly after the ranges you plotted.