Angle between Coordinate Vector and Normal Vector of Facet in a Convex Polytope, Asking for a Counterexample

Question

Definitions

Let $\mathcal{C}$ be a convex polytope in $\mathbb{R}^{D}$ with $K$-facets $F_{1},\ldots,F_{K}$. I denote the normal vector of the $k^\mathrm{th}$ facet as $\mathbf{w}\_k=(w_{k1},\ldots,w_{kD})$.

In the sequel, I will use $k$ as the index of $K$ facets and $d$ as the index of $D$ dimensions. Namely, $d\in \{1,\ldots,D\}$ and $k\in \{1,\ldots,K\}$.

Let $\mathbf{p}=(p_{1},\ldots,p_{D})$ be a point in $\mathbb{R}^{D}$. Define

$L_{d}=\{\mathbf{p}+\theta\mathbf{u}_{d}|\theta\in \mathbb{R}\},$

where $\mathbf{u}_{d}$ is the vector of the form $(0,\ldots,0,1,0,\ldots,0)$ with a $1$ only at the $d^{\mathrm{th}}$ dimension.

For $k=1,\ldots, K$, define

$G_{k}=\{d|L_{d}\cap F_{k}\neq \emptyset\}.$

Define $f:\mathbb{R}^{D}\times\mathbb{R}^{D}\rightarrow [0,1]$ as

$f(\mathbf{x},\mathbf{y})=\frac{|\mathbf{x}^\mathrm{T}\mathbf{y}|}{\left\|\mathbf{x}\right\|\left\|\mathbf{y}\right\|}.$

My conjecture

For any $\mathbf{p}\in \mathrm{int}\mathcal{C}$, there exist $d$ and $k$ such that $d\in G_{k}$ and $f(\mathbf{u}\_{d},\mathbf{w}\_{k})=\max \{f(\mathbf{u}\_{1},\mathbf{w}\_{k}),\ldots,f(\mathbf{u}\_{D},\mathbf{w}\_{k})\}$.

Can anyone provide a counterexample?

An illustrative example in $\mathbb{R}^2$

In particular, if we restrict ourself in $\mathbb{R}^2$, the above conjecture can be restated as follows:

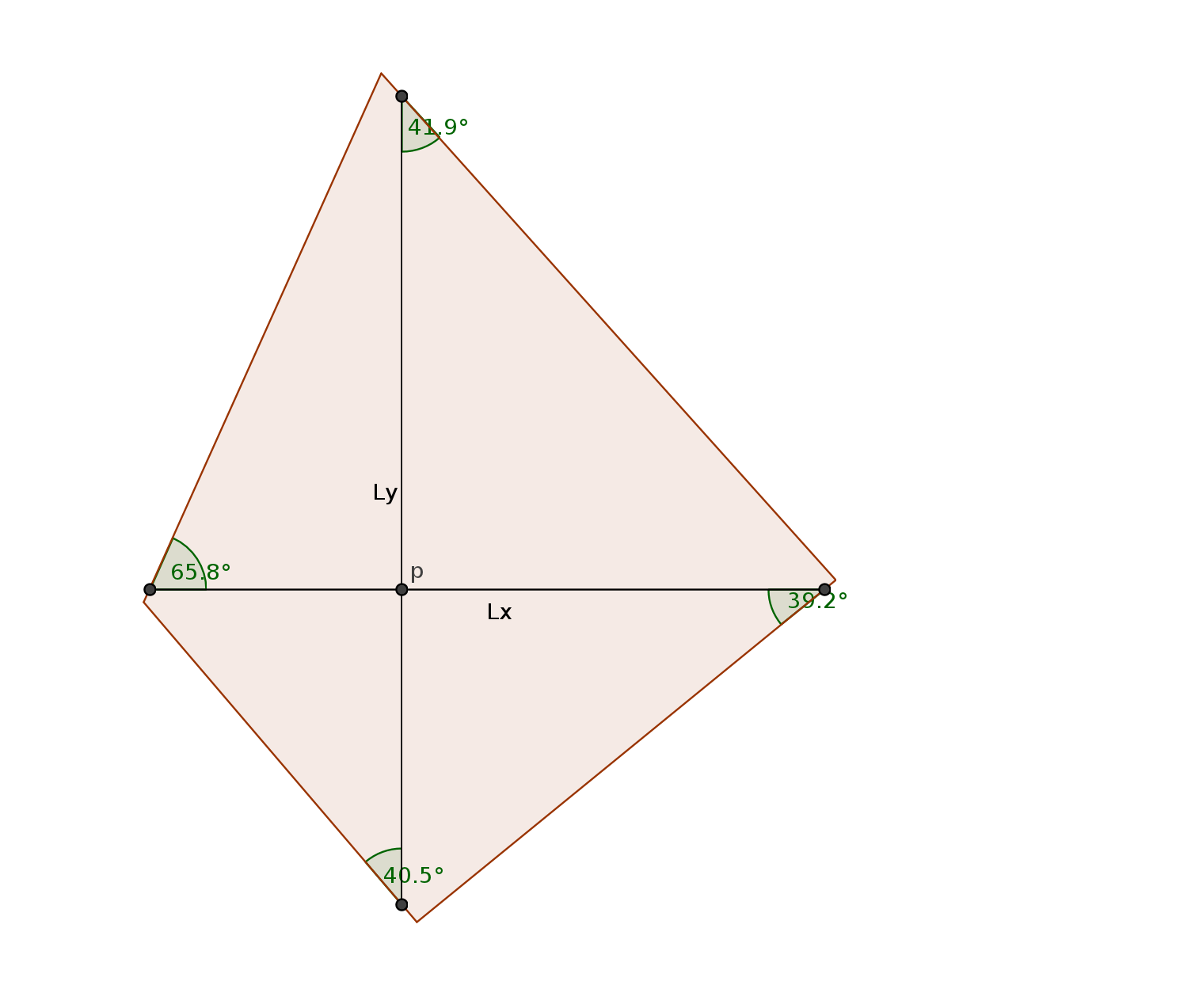
Let $p$ be a point in the interior of a convex polygon $\mathcal{C}$. Let $L_x$ and $L_y$ be two lines through $p$, which are parallel to $x$-axis and $y$-axis respectively. Consider all acute angles at intersections of $L_x$ $L_y$ and $\partial \mathcal{C}$, there is at least one angle $\geq$45°.

The figure below gives an example.

I haven't found any counterexample in $\mathbb{R}^2$, and that's why I'm considering to generalise this conjecture into high dimensional space.

Finally, any problem reformulation is also welcome.

Answer 1

$\def\u{{\bf u}}\def\p{{\bf p}}\def\q{{\bf q}}$ Consider all the points of intersection of the lines $L_d$ with the hyperplanes $H_k$ defining the facets $F_k$. Let $\q$ be the one closest to $\p$; suppose $\q=L_d\cap H_k$. Then $(d,k)$ is a desired pair.

Firstly, $\q$ should belong to $F_k$, otherwise the segment $[\p,\q]$ would intersect the boundary of a polytope at a point on another facet; thus $d\in G_k$. Next, let $\q_1,\dots,\q_D$ be the intersection points of the hyperplane $H_k$ with the lines $L_1,\dots,L_D$ (some of these points may be ideal). Then $\|\p-\q\|=\min_i\|\p-\q_i\|$ which is equivalent to your relation.

EDIT: Surely, the convexity condition IS necessary.