The group cohomology can be useful to describe the many-body quantum Condensed Matter systems with emergent underlying intrinsic Topological Orders. These classes of systems at low energy and long distance behave like certain TQFT theories. In particular, one can write explicit exact solvable Lattice quantum Hamiltonian operator $\hat{H}$ on the space discretized lattice, and its ground states give rise to discrete gauge theory TQFT with finite group $G$ (quantum double models in 2+1d or its 3+1d, etc analogous, $D(G)$, with Drinfield and Hopf algebra), twisted discrete gauge theories (twisted quantum double models $D^\omega(G)$).
See:
Twisted Quantum Double Model of Topological Phases in Two-Dimension arxiv:1211.3695 PhysRevB.87.125114
Twisted Gauge Theory Model of Topological Phases in Three Dimensions arxiv:1409.3216
PhysRevB.92.045101
Non-Abelian String and Particle Braiding in Topological Order: Modular SL(3,Z) Representation and 3+1D Twisted Gauge Theory arxiv:1404.7854 PhysRevB.91.035134
The low energy and long distance physics of the theories are the same as the Dijkgraaf-Witten topological gauge theories, Kitaev toric code and quantum double models, and some of Levin-Wen string-net models, and many of twisted discrete gauge theory.
This is how the quantum Hamiltonian operator (acting on the Hilbert space of quantum states) looks like:
$$
\hat{H}=-\sum_v A_v-\sum_f B_f,
$$
where $B_f$ is the face operator defined at each triangular face $f$, and $A_v$ is the vertex operator defined on each vertex $v$. As in the TQD model in $(2+1)$-d, each operator $A_v$ behaves as a gauge transformation on the group elements respectively on the edges meeting at $v$, and a $B_f$ detects whether the flux through face $f$ is zero. This kind of Hamiltonians generically feature ground states that are gauge invariant and bear zero flux everywhere.
A normalized
$$\omega_d\in H^d(G,U(1)),$$
as a function
$\omega:G^4\rightarrow U(1)$, satisfies the $d$-cocycle
condition. The cocycle $\omega$ will fill into the spacetime lattice $d$-simplex ($d+1$-cell). Let us take $D=4$-dim spacetime as an example below.
The operator $A_v$ is a summation
$$ A_v=\frac{1}{|G|}\sum_{[vv']=g\in G}A_v^g.
$$
The value $|G|$ is the order of the group $G$. The operator $A_v^g$ acts on
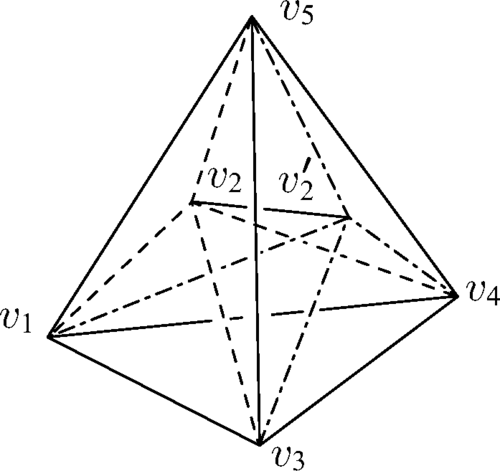
a vertex $v$ with a group element $g\in G$ by replacing $v$ by a new enumeration $v'$ that is ``slightly" less than $v$ but greater than all the enumerations that are less than $v$ in the original set of enumerations before the action of the operator, such that $v'v=g$. In a dynamical picture of Hamiltonian evolution, $v'$ is understood as on the next \textquotedblleft time" slice, and there is an edge $v'v\in G$ in the $(3+1)$ dimensional \textquotedblleft spacetime" picture. That is, the new vertex $v'$ and the original vertices before the action of $A_v$ delineate a $4$-dimensional picture. Let us consider as follows the simplest subgraph---namely a single tetrahedron---of some large $\Gamma$ to illustrate how an $A_v$ acts:
 where $v'_4v_4=g$.
where $v'_4v_4=g$.
The action of $B_f$ on a basis vector is
 The discrete
delta function $\delta_{v_1v_2\cdot v_2v_3\cdot v_3v_1}$ is unity if ${v_1v_2\cdot v_2v_3\cdot v_3v_1=1 }$, where $1$ is the identity element in $G$, and 0 otherwise. Note again that here, the ordering of $v_1,v_2$, and $v_3$ does not matter because of the identities
$\delta_{v_1v_2\cdot v_2v_3\cdot v_3v_1}
=\delta_{v_3v_1\cdot v_1v_2\cdot v_2v_3}$ and
$\delta_{v_1v_2\cdot v_2v_3\cdot v_3v_1}
=\delta_{\overline{v_1v_2\cdot v_2v_3\cdot v_3v_1}}
=\delta_{\overline{v_3v_1}\cdot \overline{v_2v_3}\cdot \overline{v_1v_2}}
=\delta_{v_1v_3\cdot v_3v_2\cdot v_2v_1}$.
In other words,
in any state on which $B_f=1$ on a triangular face $f$, the three group degrees of freedom
around $v$ is related by a chain
rule:
$$
v_1v_3=v_1v_2\cdot v_2v_3
$$
for any enumeration
$v_1,v_2,v_3$ of the three vertices of the face $f$.
The discrete
delta function $\delta_{v_1v_2\cdot v_2v_3\cdot v_3v_1}$ is unity if ${v_1v_2\cdot v_2v_3\cdot v_3v_1=1 }$, where $1$ is the identity element in $G$, and 0 otherwise. Note again that here, the ordering of $v_1,v_2$, and $v_3$ does not matter because of the identities
$\delta_{v_1v_2\cdot v_2v_3\cdot v_3v_1}
=\delta_{v_3v_1\cdot v_1v_2\cdot v_2v_3}$ and
$\delta_{v_1v_2\cdot v_2v_3\cdot v_3v_1}
=\delta_{\overline{v_1v_2\cdot v_2v_3\cdot v_3v_1}}
=\delta_{\overline{v_3v_1}\cdot \overline{v_2v_3}\cdot \overline{v_1v_2}}
=\delta_{v_1v_3\cdot v_3v_2\cdot v_2v_1}$.
In other words,
in any state on which $B_f=1$ on a triangular face $f$, the three group degrees of freedom
around $v$ is related by a chain
rule:
$$
v_1v_3=v_1v_2\cdot v_2v_3
$$
for any enumeration
$v_1,v_2,v_3$ of the three vertices of the face $f$.
Here are how the space lattice and the triangulation of spacetime lattices looks like:
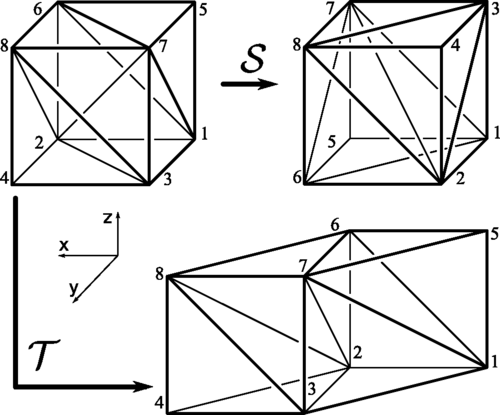
Here are how the $SL(N,\mathbb{Z})$'s modular $S$ and $T$-transformations look like: