Point on a line nearest a point in Banach space

Question

I have a Banach space geometry question (a curiosity-driven spin-off from a research topic). Given a point $x$ on the unit sphere of a Banach space and a vector $y\ne 0$, there is a multiple $t_0y$ of $y$ for which $\|t_0y-x\|$ is minimized (this will be unique if the norm is strictly convex).

My question is this:

For which Banach spaces $X$ is it guaranteed that $\|t_0y\|\le \|x\|$?

My "Euclidean intuition" suggested that this should be the case for all Banach spaces, but a little experimentation showed that this is not the case. You quickly see this is really a question about two dimensions. In fact it seems to fail for every $\ell^p$, $p\ne 2$ (see the attached figure in $p=1.2$).

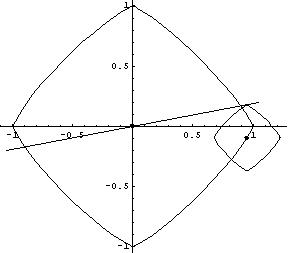
Could it be true that this property characterizes Hilbert space? (I looked at the obvious sources: (MO 11192 and papers mentioned in there and didn't find anything of the sort).

Answer 1

The answer is no in dimension 2 and yes in dimension 3 and higher. The property that the nearest-point projection to a line does not increase the norm is equivalent to the symmetry of orthogonality relation defined as follows: $x$ is orthogonal to $y$ iff $\|x+ty\|\ge\|x\|$ for all $t\in\mathbb R$.

It is well-known that symmetry of this orthogonality relation in dimension $\ge 3$ implies that the norm is Euclidean, see e.g. Thompson's "Minkowski geometry", Theorem 3.4.10.

This is not the case in dimension 2. There are many counter-examples (I believe they are called Radon planes). Basically you only need to ensure that every unit vector with its unit orthogonal one span a constant parallelogram area, this is easy to satisfy and is equivalent to the symmetry of orthogonality. For a simple explicit example (although non-smooth), consider a norm on the plane whose unit ball is a regular hexagon.

Answer 2

I do not know the answer, but I would suggest having a look at the book "Characterizations of inner product spaces" by Dan Amir, which features, who would have guessed, many many characterizations of inner product spaces. There is also a book called "Inner product structures" by Istratescu which I have found useful.