52
$\begingroup$

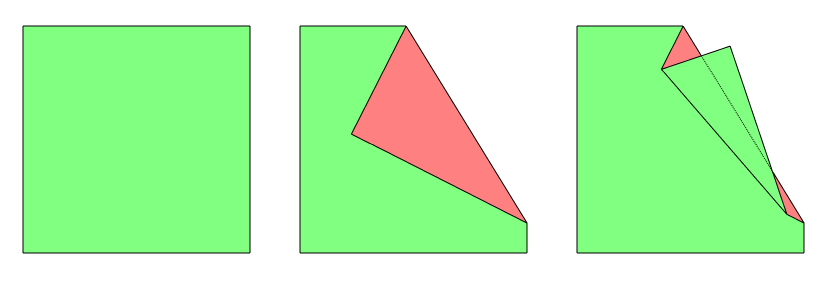
The falsity of the following conjecture would be a nice counter-intuitive fact.

Given a square sheet of perimeter $P$, when folding it along origami moves, you end up with some polygonal flat figure with perimeter $P'$.
Napkin conjecture: You always have $P' \leq P$.

In other words, you cannot increase the perimeter using any finite sequence of origami folds.

Question 1: Intuition tells us it is true (how in hell can it increase?). Yet, I think I read somewhere that there was some weird folding (perhaps called "mountain urchin"?) which strictly increases the perimeter. Is this true?
Note 1: I am not even sure that the initial sheet's squareness is required.

I cannot find any reference on the internet. Maybe the name has changed; I heard about this 20 years ago.

The second question is about generalizing the conjecture.

Question 2: With the idea of generalizing the conjecture to continuous folds or bends (using some average shadow as a perimeter), I stumble on how you can mathematically define bending a sheet. Alternatively, how do you say "a sheet is untearable" in mathematical terms?
Note 2: It might also be a matter of physics about how much we idealize bending mathematically.

Improve this question
$\endgroup$
4
  • 3
    $\begingroup$ en.wikipedia.org/wiki/Napkin_folding_problem Has some relevant information, you were probably thinking of the "sea urchin" solution, however, it does require some stretching of the paper. $\endgroup$
    – trutheality
    Commented Nov 21, 2010 at 22:24
  • 3
    $\begingroup$ There are several references to this problem in Lang, Robert J., Origami design secrets, Mathematical methods for an ancient art. A K Peters, Ltd., Natick, MA, 2003. ISBN: 1-56881-194-2, MR2013930 (2004g:52001). Unfortunately, the relevant pages aren't available on Google. $\endgroup$
    – Gerry Myerson
    Commented Nov 21, 2010 at 22:39
  • 3
    $\begingroup$ from the MO : if you google "napkin conjecture" you do not get the wikipedia entry.So I had done my homework.. $\endgroup$
    – Jérôme JEAN-CHARLES
    Commented Nov 22, 2010 at 1:07
  • 1
    $\begingroup$ "math.lsu.edu/~verrill/origami/perimeter" also claims to give an example where the perimeter increases. $\endgroup$
    – Zsbán Ambrus
    Commented Apr 4, 2011 at 12:54

3 Answers 3

Reset to default
47
$\begingroup$

There is a general version of this question which is known as "the rumpled dollar problem". It was posed by V.I. Arnold at his seminar in 1956. It appears as the very first problem in "Arnold's Problems":

Is it possible to increase the perimeter of a rectangle by a sequence of foldings and unfoldings?

According to the same source (p. 182),

Alexei Tarasov has shown that a rectangle admits a realizable folding with arbitrarily large perimeter. A realizable folding means that it could be realized in such a way as if the rectangle were made of infinitely thin but absolutely nontensile paper. Thus, a folding is a map $f:B\to\mathbb R^2$ which is isometric on every polygon of some subdivision of the rectangle $B$. Moreover, the folding $f$ is realizable as a piecewise isometric homotopy which, in turn, can be approximated by some isotopy of space (which corresponds to the impossibility of self-intersection of a paper sheet during the folding process).

Have a look at

  • A. Tarasov, Solution of Arnold’s “folded rouble” problem. (in Russian) Chebyshevskii Sb. 5 (2004), 174–187.

  • I. Yashenko, Make your dollar bigger now!!! Math. Intelligencer 20 (1998), no. 2, 38–40.

A history of the problem is also briefly discussed in Tabachnikov's review of "Arnold's Problems":

It is interesting that the problem was solved by origami practitioners way before it was posed (at least, in 1797, in the Japanese origami book “Senbazuru Orikata”).

Improve this answer
$\endgroup$
7
  • 3
    $\begingroup$ A reference is A S Tarasov, Solution of Arnolʹd's "folded ruble'' problem. (Russian. Russian summary) Chebyshevskiĭ Sb. 5 (2004), no. 1(9), 174–187. MR2098977 (2005e:52005). $\endgroup$
    – Gerry Myerson
    Commented Nov 21, 2010 at 22:39
  • $\begingroup$ @Jérôme JEAN-CHARLES: You are welcome. $\endgroup$
    – Andrey Rekalo
    Commented Nov 22, 2010 at 12:57
  • 4
    $\begingroup$ @Andrey Rekalo : may be you knew it already but the general audience (as well as me) would appreciate it for the sake of poetry. “Senbazuru Orikata” stands for "Folding a thousand cranes" (Sen =1000 ,zuru = crane , orikata =folding). Why a thousand folds is not totally clear : something to do with the fact that the result was used for garlands (long ones I guess) in ceremonies. $\endgroup$
    – Jérôme JEAN-CHARLES
    Commented Nov 24, 2010 at 2:23
  • 3
    $\begingroup$ The complete title is hi den sen ba zuru ori kata (秘傳千羽鶴折形). The "Hiden" means secret, and the "ba" (literally "feather") is a marker that makes it clear that the thousand is counting birds. There is an incomplete scan at: origami.gr.jp/SenbazuruOrikata $\endgroup$
    – S. Carnahan ♦
    Commented Apr 9, 2011 at 5:10
  • 1
    $\begingroup$ A video showing A S Tarasov solution can be found at the source file of Anton Petrunin and Allan Yashinski lecture notes on piecewise distance preserving maps. $\endgroup$
    – Tadashi
    Commented Jan 6, 2017 at 22:10
27
$\begingroup$

Permit me to supplement Andrey's definitive answer.

First, as Gerry Myerson says, this problem is discussed in Robert Lang's Origami Design Secrets: pp.315-318, under the title "The Margulis Napkin Problem." He credits the problem to Gregori Margulis.

Second, the problem is discussed in Igok Pak's book Lectures on Discrete and Polyhedral Geometry, p.354, which is available online. You can pretty much guess the proof from the following instructive figure of Igor's:


   Napkin

Third, there is another surprising result that is intellectually analogous to increasing the perimeter by folding: The volume enclosed by any convex polyhedron can be increased by bending the surface (retaining intrinsic isometry) to render it nonconvex! See Chapter 39 of Igor's book, p.339ff.

Improve this answer
$\endgroup$
3
  • $\begingroup$ Thank you: a very very nice reference ,I downloaded the book, such beautiful drawings. Yet I must confess the above is not enough for me to see the folds ( though I looked in the book only at the part you mentioned). Is there (roughly) a date for resolution, the book says "unsolved for decades" (but not from when)? $\endgroup$
    – Jérôme JEAN-CHARLES
    Commented Nov 23, 2010 at 16:40
  • 3
    $\begingroup$ @JJ-J: It depends on what you mean by "resolution." Here is Robert Lang: "several origami artists had created models [that solved the problem] years before it had even arisen in mathematical circles." Among those artists is Lang himself, I gather. So this may be a case where the solution was known before the problem was posed! $\endgroup$
    – Joseph O'Rourke
    Commented Nov 23, 2010 at 21:36
  • 3
    $\begingroup$ OK I get it and I think this is a very interesting epistemological fact. Definitely a hard riddle that of asking a mathematician :" Give me a problem whose solution was known much before it was answered?" $\endgroup$
    – Jérôme JEAN-CHARLES
    Commented Nov 23, 2010 at 23:35
26
$\begingroup$

In addition to the answers above, here are some remarks from my paper in Russian; part of it used in the last lecture here. (Sorry for self-advertisement.)

1. An other solution. It is based on idea of Yashenko [Math. Intelligencer 20 (1998)]. This way you can increase the perimeter just a bit, but it is done by repeating one fold (which is very simple but not "simple" in the sense below).

alt text

2. It is still not known if you can increase the perimeter by a sequence of natural folds; i.e., folds like this: alt text

I just learned that this problem also appears in Pak's book, Problem 40.16b; it is marked by [$*$] which means that the problem is open.

Improve this answer
$\endgroup$
3
  • 1
    $\begingroup$ I was a bit surprised by fact 2, after some reflection OK. Moreover I suddenly realize that something is dreadfully missing from the question: the figure at start and at the end should be flat ( a closed polygonal line made of finitely many segments). In you first lines of 3 drawings can you confirm that the first two are in volumes and the last one is flat? $\endgroup$
    – Jérôme JEAN-CHARLES
    Commented Apr 11, 2011 at 0:33
  • $\begingroup$ Sorry I don't read Russian but the drawings are still worth looking at thank you. $\endgroup$
    – Jérôme JEAN-CHARLES
    Commented Apr 11, 2011 at 0:36
  • 1
    $\begingroup$ @Jérôme: in (1), figure at start is net of folds for middle picture. it is iteration number 5 of "simple" folds. The picture on the right is the limit after infinite number of iteration, but it is enough to make finite number of iterations to increase the perimeter. $\endgroup$
    – Anton Petrunin
    Commented Apr 11, 2011 at 4:47

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .