1
$\begingroup$

I am going through this paper by Tanaka. In the proof of Proposition 3.2(1) given below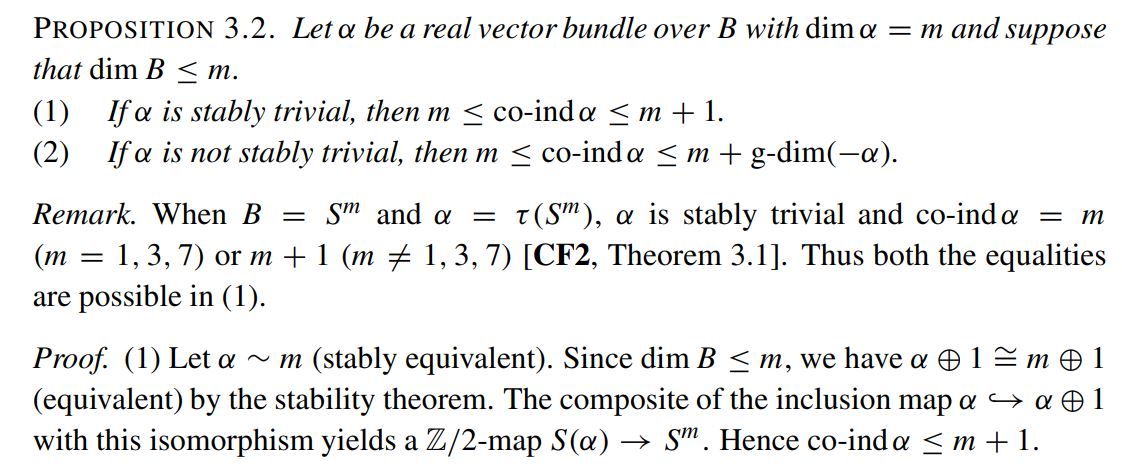enter image description here

The author says that by the stability theorem as $\dim (B)\le m$ we have $\alpha\oplus1\cong m\oplus1$. But I can't figure that out, Since we are given that $\alpha $ and $m$ are stably equivalent we have that $\alpha\oplus k\cong m\oplus n$ for some trivial bundles $k,n$. Unfortunately, I can't figure that out.

I am using the stability theorem in Dale Husemoller's Fiber bundles book chapter 9.

kindly help. regards

Improve this question
$\endgroup$
3
  • 2
    $\begingroup$ It seems to me that `stably equivalent' here is meant to not forget about the rank (that is, we ask $\alpha \oplus k = m \oplus k$ for some $k$). But what you seem to be confused about is the statement of the stability theorem, which says (something like) that if $\dim B = m$ then for any $n > m$ stably isomorphic vector bundles of rank $n$ over $B$ are in fact isomorphic. $\endgroup$
    – mme
    Commented Feb 6 at 14:18
  • $\begingroup$ @mme How can we make that assumption that rank is preserved and even if the rank is preserved why that k=1 is true.? can you explain a bit? $\endgroup$
    – Devendra Singh Rana
    Commented Feb 6 at 17:14
  • 1
    $\begingroup$ The bundles $\alpha$ and $m$ have the same rank (namely $m$), so $\alpha\oplus k \cong m\oplus n$ is only possible if $k = n$. As for why $\alpha\oplus 1 \cong m\oplus 1$, this follows from obstruction theory, see the third paragraph of this answer for example. $\endgroup$
    – Michael Albanese
    Commented Feb 9 at 0:17

0

Reset to default

You must log in to answer this question.