7
$\begingroup$

The subsequent statements are extracted from the article titled 'Generating r-regular graphs' (https://doi.org/10.1016/S0166-218X(02)00593-0).

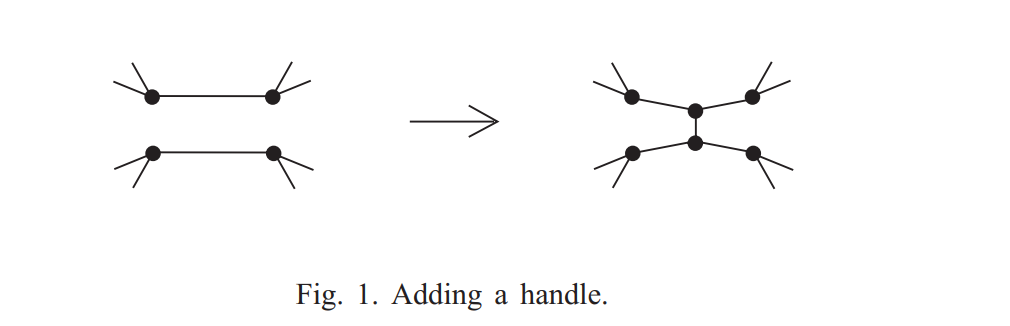
A well-known classical theorem of Steinitz and Rademacher [22] states that the class $\mathcal{G}$ of 3-connected 3-regular planar simple graphs can be generated from the Tetrahedron by adding handles, a graph operation illustrated in Fig. 1 below.

enter image description here

[22] E. Steinitz, H. Rademacher, Vorlesungen über die Theorie de Polyeder, Berlin, 1934.

In my manuscript, I similarly express this statement and cite the reference [22]; however, the reviewer mentioned that: [22] is a whole book. Write exactly to which part you refer. As the original book is in German, I am uncertain about the particular chapter or section that discusses this theorem. Is there someone familiar with German who can help check which specific chapter in the book this theorem is in? Thank you in advance.

Additionally, I would like to inquire whether there is an English version of this book.

Improve this question
$\endgroup$
3
  • 1
    $\begingroup$ The book can be read here with a free account. I didn't notice anything obvious in a quick scan but my German barely exists. $\endgroup$
    – Brendan McKay
    Commented Dec 1, 2023 at 6:41
  • $\begingroup$ @Brendan McKay Unfortunately, do you know that there is an English version of this theorem. Perhaps I would consider citing an additional reference. $\endgroup$
    – Licheng Zhang
    Commented Dec 1, 2023 at 8:12
  • 1
    $\begingroup$ Almost certainly, but I don't know an example offhand. In searching don't forget to search for the dual version which is generation of 3-connected triangulations. $\endgroup$
    – Brendan McKay
    Commented Dec 1, 2023 at 10:36

1 Answer 1

Reset to default
6
$\begingroup$

According to Frank Lutz's article it's in paragraph 46 of the Steinitz-Rademacher book: "every triangulated 2-sphere can be reduced to the boundary of the tetrahedron by a sequence of edge contractions".

Improve this answer
$\endgroup$
1
  • 2
    $\begingroup$ Yes, section 46 on page 192. "Jedes K-Polyeder läßt sich aus dem gewöhnlichen Tetraeder durch reguläre Spaltungen ableiten." It seems to me like the operation OP drew rather than the dual operation on triangulations. Equivalent of course. $\endgroup$
    – Brendan McKay
    Commented Dec 1, 2023 at 13:44

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .