There are different versions of the Suslinian property for continua. One is called finitely Suslinian, a term I think I learned about from the OP - if I recall correctly, it asks that, for any eps>0, there is no infinite collection of pairwise disjoint subcontinua all having diameter at least eps.
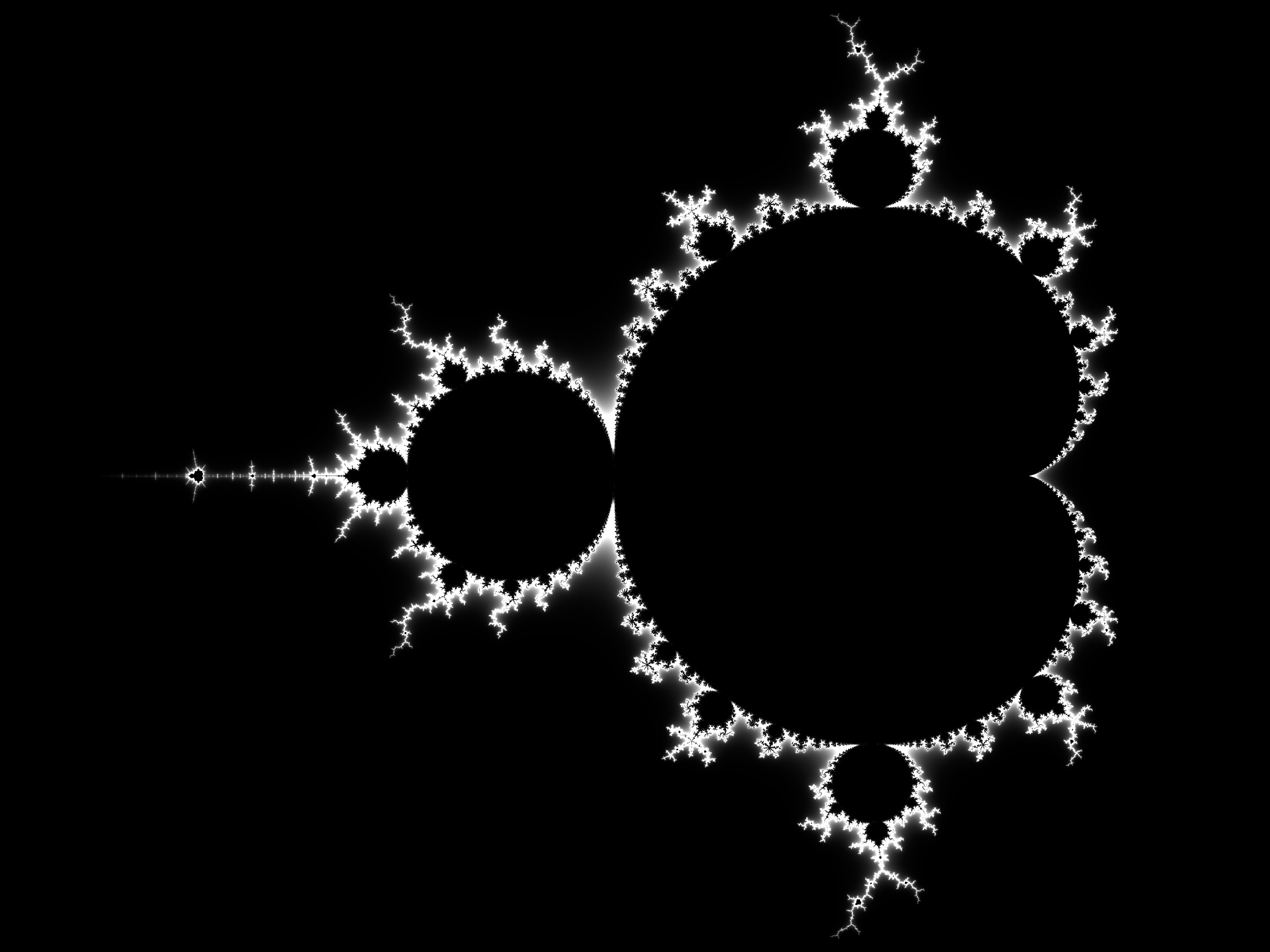
For boundaries of planar simply-connected domains, being finitely Suslinian is equivalent to local connectivity. The boundary of the Mandelbrot is such a set (the complement of the Mandelbrot set in the sphere is a simply-connected domain). So local connectivity certainly implies Suslinian. In particular, IF the Mandelbrot set is locally connected (as is widely believed), it is Suslinian.
The opposite implication is false for general boundaries of simply-connected domains; consider for example the Warsaw circle. On the other hand, the Knaster buckethandle is an example of a non-Suslinian continuum that is also the boundary of a simply-connected domain.
It is plausible that, if the Mandelbrot set turned out to be not locally connected, it would also not be Suslinian. (Not only because I think it is very likely that the Mandelbrot set is locally connected.) Indeed, if local connectivity fails, then we know this would have to be at some "infinitely renormalisable" parameters; i.e., there would be some nested sequence of little Mandelbrot copies that did not shrink to a point. But then one would surely expect to have several choices of little Mandelbrot copies at each stage, resulting in uncountably many different choices of such continua.
Of course, this is a heuristic argument, not a proof. It is not clear to me whether it would be possible to give a proof without also having sufficient control to settle the question of local connectivity of the Mandelbrot set. :) But perhaps someone else can comment.
I remark that in all cases of NOT locally connected quadratic Julia sets that we understand sufficiently to answer the question, the Julia set is NOT Suslinian.