6
$\begingroup$

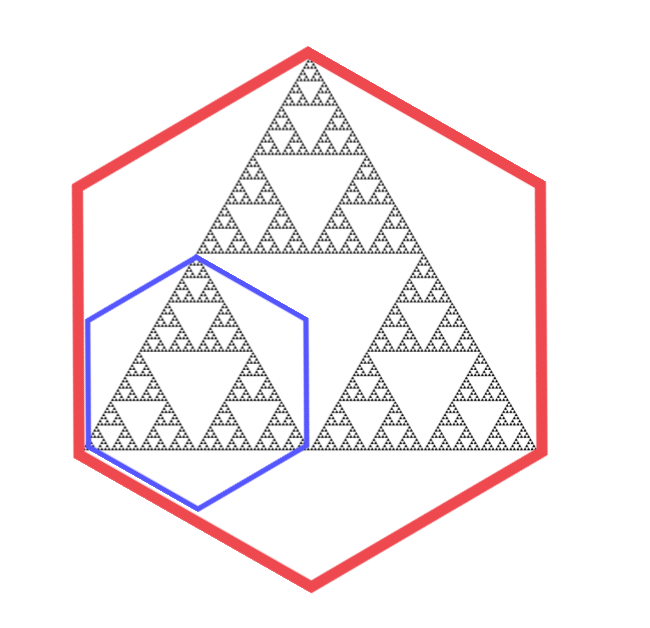
The Open Set Condition is fulfilled by an Iterated Function System in the plane $\{\mathbb{R}^2, \phi_1, \phi_2, \dots, \phi_m \}$ if there exists a nonempty open set $V$ such that $\bigcup \phi_{i}(V) \subseteq V$ and $\phi_{1}(V), \phi_{2}(V), \dots, \phi_{m}(V)$ are all disjoint.

I think it would be very natural then to choose, in the case of the Sierpinski Triangle, the equilateral open triangle "with same vertices" as the first iteration of Sierpinski triangle. However, in pdf by Bandt and Wikipedia it is chosen this hexagon... I wonder why would they be complicating things?

enter image description here

Improve this question
$\endgroup$
2
  • $\begingroup$ Where did this question come from? I want to learn more about stuff like this $\endgroup$
    – Sidharth Ghoshal
    Commented Apr 30, 2023 at 20:38
  • $\begingroup$ From my study on Fractal Geometry. I'm using mostly the books Fractal Geometry by Falconer and Fractals in Prob. And Analysis by Bishop-Peres. $\endgroup$
    – anchova
    Commented Apr 30, 2023 at 22:33

1 Answer 1

Reset to default
9
$\begingroup$

I guess that illustration relates to the paper

Bandt, Christoph; Nguyen Viet Hung; Rao, Hui, On the open set condition for self-similar fractals, Proc. Am. Math. Soc. 134, No. 5, 1369-1374 (2006). ZBL1098.28004.

They want to start with the iterated function system and systematically construct an open set that works. Unlike the Sierpinski gasket, in most IFSs the open set cannot be chosen to be connected, so this requires some work.

Improve this answer
$\endgroup$

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .