Mutation Wiki My related question 1 My related question 2
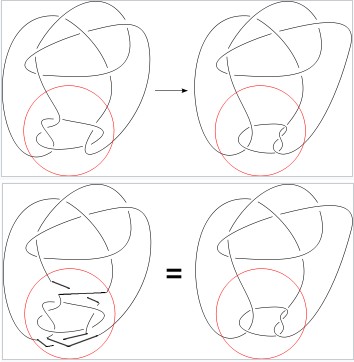
Top: How wiki describes mutation. Doesn't generalize well.
Bottom: How I think of it.
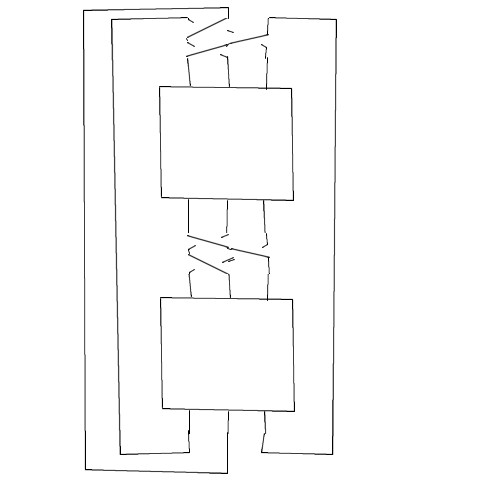
Now replace "four" in the Wiki text by "six". Is anything known about such higher mutants? This could look e.g. like this:
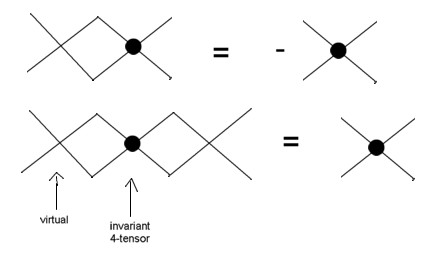
Endnote: This is IMHO still not the optimal framework to think about mutation. You need virtual crossings, and since they don't mix well with overcrossings - yikes, forbidden move - to work with the antisymmetric rank 4 tensor of the aforementioned Lie algebra.
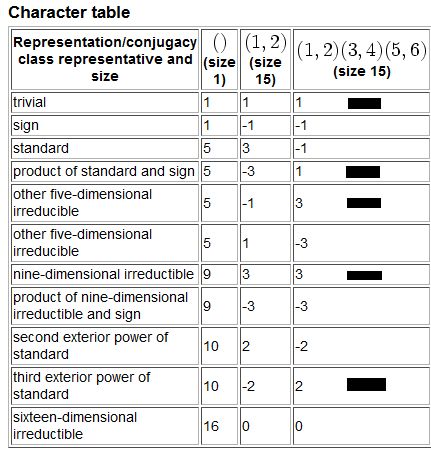
Then you can classify any tangle by symmetry under the symmetric group $S_4$ and find that mutation equality kills the modules of the two $d=3$ irreps. My dabblings with higher tangles suggest that, likewise, for the symmetric group $S_6$ only the five irreps marked in the pic below survive for a "decent" knot polynomial, and it would be interesting which "higher mutations" produce this result. But I ask that as a new question eventually, this was very shorthand...