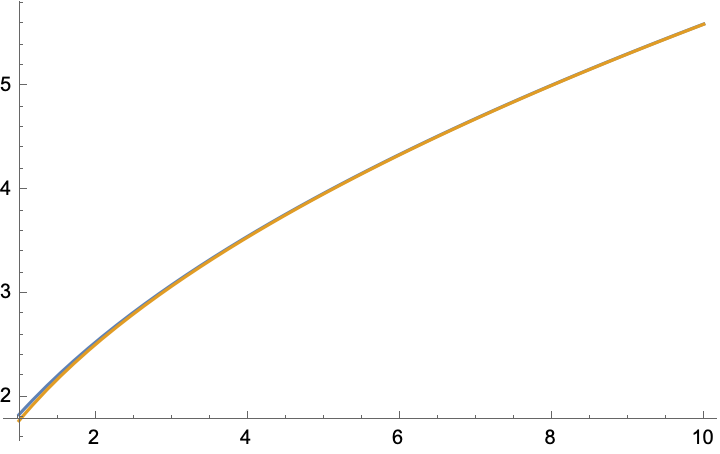
$\newcommand\Ga\Gamma$Let $f(x)$ denote your hypergeometric expression. Then
$$f(x)\sim\sqrt{\pi x}$$
(as $x\to\infty$).
Indeed,
$$f(x)=\sum_{k\ge0}\frac{(1/2)_k}{k!}r_k(x)^2,$$
where $(a)_k:=a(a+1)\cdots(a+k-1)=\Ga(a+k)/\Ga(a)$ and
$$r_k(x):=\frac{(x)_k}{(x+1/2)_k}=\frac{\Ga(x+k)}{\Ga(x)}\Big/\frac{\Ga(x+1/2+k)}{\Ga(x+1/2)}
\sim\sqrt{\frac x{x+k}}$$
uniformly in $k\ge0$ (as $x\to\infty$).
So,
\begin{equation*}
f(x)\sim x\,\sum_{k\ge0}\frac{(1/2)_k}{k!} \frac1{x+k}
=\frac{\sqrt{\pi }\, \Ga(x+1)}{\Ga(x+1/2)}
\sim\sqrt{\pi x} \tag{1}\label{1}
\end{equation*}
(as $x\to\infty$).
Details on the equality in \eqref{1}:
\begin{equation*}
\begin{aligned}
\sum_{k\ge0}\frac{(1/2)_k}{k!} \frac1{x+k}
& =\sum_{k\ge0}\frac{(1/2)_k}{k!} \int_0^1 dt\,t^{x+k-1} \\
& =\int_0^1 dt\,t^{x-1}\sum_{k\ge0}\frac{(1/2)_k}{k!} t^k \\
& =\int_0^1 dt\,t^{x-1}(1-t)^{-1/2},
\end{aligned}
\end{equation*}
in view of the Maclaurin series for $(1-t)^{-1/2}$.
Therefore,
\begin{equation*}
\begin{aligned}
x\,\sum_{k\ge0}\frac{(1/2)_k}{k!} \frac1{x+k}
=x\,B(x,1/2)= x\,\frac{\Ga(1/2)\, \Ga(x)}{\Ga(x+1/2)}
=\frac{\sqrt{\pi }\, \Ga(x+1)}{\Ga(x+1/2)},
\end{aligned}
\end{equation*}
as claimed.