Let us define a pyramid as a convex polyhedron with one quadrilateral face and four triangular faces.
Question: How many pyramids (or families of pyramids) are known that can fill 3D space without gaps? The packing need not be face to face or edge to edge (not sure if these relaxations matter at all).
Remark: the same question on tetrahedrons is not fully resolved as explained in https://www.jstor.org/stable/2689983
About the tetrahedra problem, I have found this reference/proof that they cannot be used to fill 3D space (since the proven upper bound is smaller than $100 \cdot (1-2.7 \cdot 10^{-25})\%$: https://en.wikipedia.org/wiki/Tetrahedron_packing ), and here is another interesting resume on the same topic (dated 2010): https://www.nytimes.com/2010/01/05/science/05tetr.html\ About a solid lower bound, we have Chen's valuable paper entitled "A Picturebook of Tetrahedral Packings": https://www.semanticscholar.org/paper/A-Picturebook-of-Tetrahedral-Packings.-Chen/d20f70381ee445a60222e402ee39a0c32c07f804
Now, let us answer to the square pyramid packing problem: I have to say that my first thought has been that we couldn't use square pyramids to fill the gap... and it seems that I was right from the beginning, since here is a very interesting result by A. Bezdek $\&$ W. Kuperberg (see p. $10$ of DENSE PACKING OF SPACE WITH VARIOUS CONVEX SOLID, whereas a lower bound is shown in Figure 6, ibid.): https://arxiv.org/pdf/1008.2398.pdf
Finally, let me show a trivial composition that I have just made in a rush (many thanks to GeoGebra) to show how square pyramids and tetrahedra would fit very well if combibed 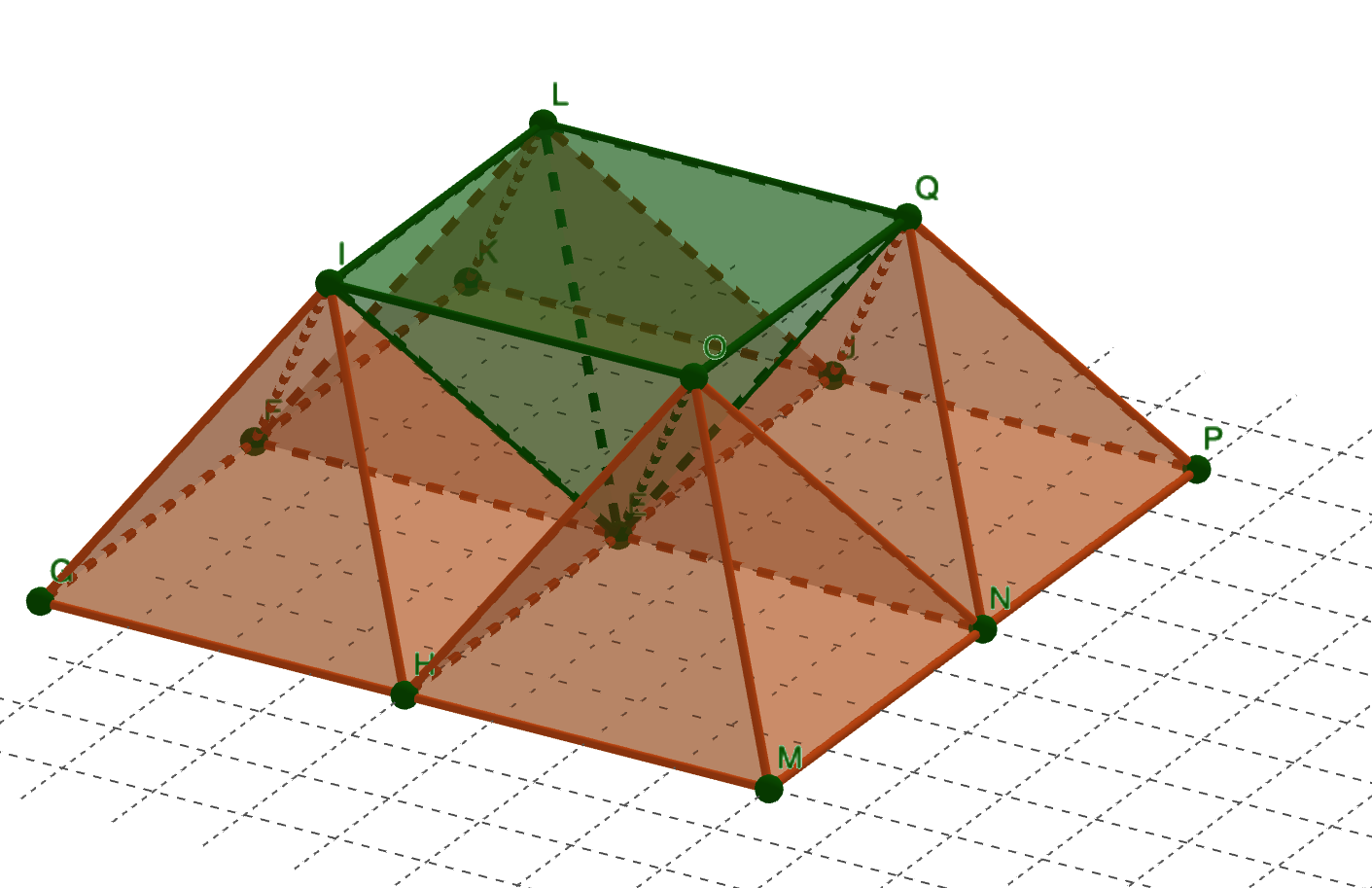togheter: 
$\&$ Tetrahedra togheter" />
