8
$\begingroup$

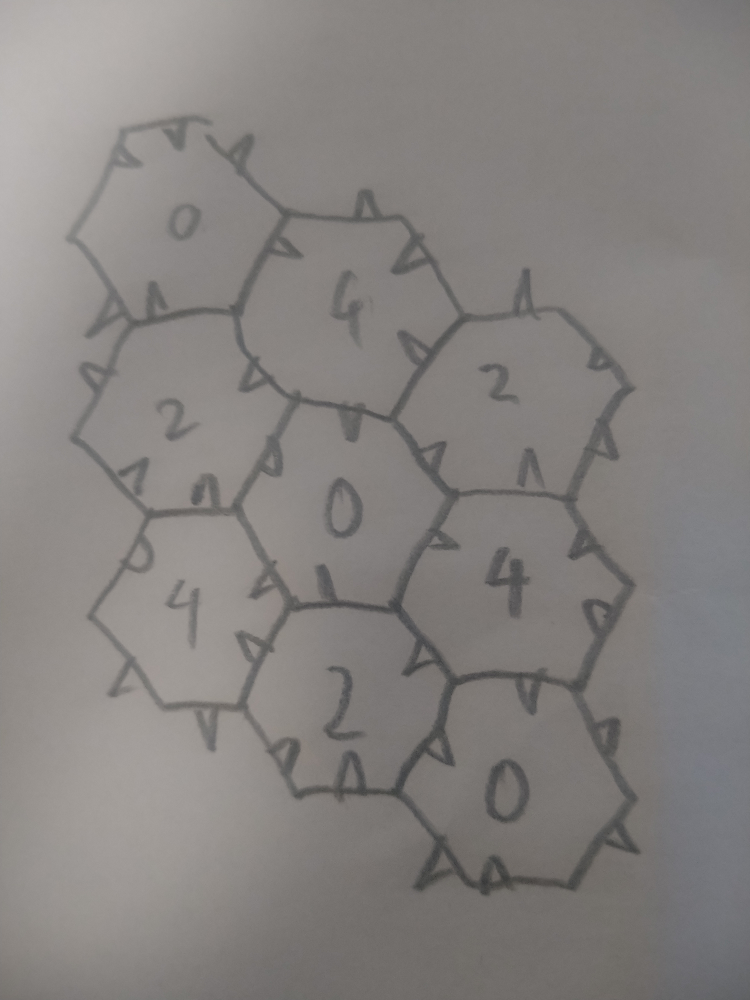
This hexagon-with-dents is a tile which, I think, tiles the plane in a necessarily aperiodic way:

     ________  __ 
    /        \/  \__
   _\              /
  /                \
 /                  \
/                    \
\                    /
 \                  _\
  \                /
  /_              /
    \_/\_________/

This is essentially the Socolar–Taylor tile. I'm rather surprised that this tile does not appear in the paper of Socolar and Taylor, and that it also doesn't appear in Wikipedia's list of aperiodic sets of tiles.

Did I miss something?
Am I maybe wrong to claim that this tile tiles the plane in a necessarily aperiodic way?

Question: Does the above tile tile the plane in a necessarily aperiodic way?

Improve this question
$\endgroup$
9
  • $\begingroup$ Strictly speaking, this is not a hexagonal tile. The dents and extrusions count as additional sides, so the tile would be called 24-gonal. $\endgroup$
    – Oscar Lanzi
    Commented Sep 16, 2022 at 10:52
  • $\begingroup$ Well, you don't give us a proof of aperiodicity, or say how it is related to the Socolar–Taylor tile. Are the dents supposed to enforce the matching rules prescribed by the decorations in Figure 1? $\endgroup$
    – Algernon
    Commented Sep 16, 2022 at 12:17
  • $\begingroup$ @Algernon. Yes. The black lines in Figure 1 of the Socolar-Taylor paper can be consistently oriented (so that all black triangles of varying sizes run conterclockwise). The dents in my tile mark to location where the black lines of Socolar-Taylor meet the boundary of the hexagon. [Oscar Lanzi: you're absolutely correct] $\endgroup$
    – André Henriques
    Commented Sep 16, 2022 at 12:24
  • 1
    $\begingroup$ @AndréHenriques: I was just pointing out the loopholes in your (non-existent) argument. Wasn't that the reason you posted your question? $\endgroup$
    – Algernon
    Commented Sep 16, 2022 at 13:40
  • 3
    $\begingroup$ Cool use of ASCII art! $\endgroup$
    – Dan Romik
    Commented Sep 16, 2022 at 14:52

1 Answer 1

Reset to default
19
$\begingroup$

This seems to admit periodic tilings, so there is probably some problem. periodic. Numbers are rotation amounts.

Improve this answer
$\endgroup$
2
  • 2
    $\begingroup$ There's no problem. You have found a periodic tiling. $\endgroup$
    – André Henriques
    Commented Sep 16, 2022 at 13:37
  • 10
    $\begingroup$ I just meant, problem with the Socolar-Taylor connection. $\endgroup$
    – Ville Salo
    Commented Sep 16, 2022 at 13:44

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .