Configurations of points and circles

Question

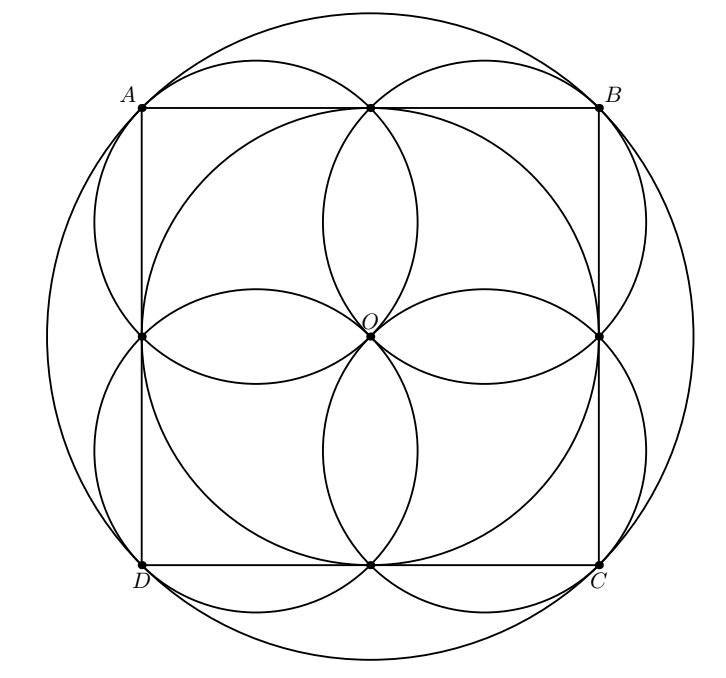
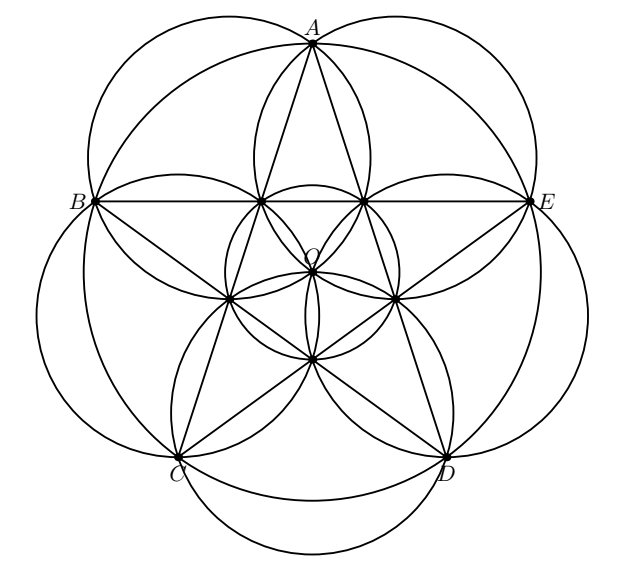
Problem. Several circles are drawn on the plane and all points of their intersection or touching are marked. For which $n$ it is possible that each circle contains exactly $n$ marked points and each point belongs to exactly $n$ circles?

Examples for $n=2,3$ are trivial. For $n=4$ and $5$ there only two known examples on the figures below (we apply an inversion with a center not lying on these lines to obtain the required configurations). Are there examples for $n>5$ and other examples for $n=4,5$?

UPD $n=5$ example arises from stereographic projection of $12$ vertices of an icosahedron and $12$ circles passing through any $5$ vertices incident to the same vertex. Ilya Bogdanov noticed that $n=4$ example arises from stereographic projection of the following configuration of $10$ point and $10$ circles on the sphere. Consider polyhedron which is a convex hull of the following $10$ points: a vertex of some octahedron, $4$ midpoints of edges incident with this vertex, $4$ centers of faces incident with this vertex and the center of the octahedron; and $10$ circles: $8$ circumscribed circles of all faces of the polyhedron, the circle through midpoints and the circle through face centers.

Answer 1

This is just a partial answer.

Notice that in all your examples so far, for any given pair of points in your drawing, there are at most two circles containing both of them. I claim that there is no example with $n \geq 6$ that has this property.

Supposing there were such an example, draw it out (with all vertices drawn -- no points at infinity). You have a non-simple planar graph $G$, non-simple because some of your vertices have two different edges connecting them. We may suppose $G$ is connected, because every connected component of a valid configuration is also a valid configuration. For every pair of doubly connected vertices in $G$, delete one of the two edges connecting them, so that the result is a simple connected planar graph $H$.

If you have $v$ vertices, then you also have $v$ circles in your configuration. Each circle gives you exactly $n$ edges in $G$, for a total of $vn$ edges in $G$. This means there are $\geq\! vn/2$ edges in $H$. But it is well known that, in a planar graph, the number of edges must be $\leq\! 3v-6$. (This formula can be found, for example, here on Wikipedia.) If $n \geq 6$, then the number of edges in $H$ is $\geq\! vn/2 \geq 3v$, so $H$ violates this formula.

Answer 2

You can use a "hypercube". Take n vectors with length 1. Now, make every combination as the sum of the vectors. In general, you now have 2ⁿ different points, which we can represent with "binary". The circles are every circle with radius 1 centred around one of the points. Every point has in general n points with distance 1, which are obtained by changing a single digit of the binary string. This means that every circle contains n points, in general. Analogously, every point lies on n different circles with radius 1, which are the circles centred around the neighbours.

I first came up with a more complicated way, extending the contructions of Clifford's circle theorems (there is a wikipage), also using 2ⁿ points and circles, but then I came up with the easy solution.