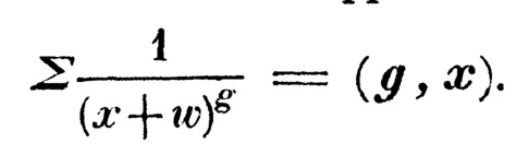What is the history of Eisenstein series? Did the mathematician Eisenstein actually encounter them?
There are, as far as I know, two major perspectives on what Eisenstein series are. The first is in the study of modular forms. By summing over lattice points, one obtains a holomorphic modular form
$$E_{2k}(z) = \sum\limits_{(m,n) \neq (0,0)} \frac{1}{(m+nz)^{2k}}$$
for $\operatorname{SL}_2(\mathbb Z)$ which together with the cusp forms exhausts the space of modular forms of a given weight.
The other perspective is in considering a unitary representation $(\pi, V)$ of a Levi subgroup $M$ of a semisimple real Lie group $G$. The representation considered should be left $\Gamma \cap M$- invariant for a lattice $\Gamma$ of $G$. If $P$ is a parabolic subgroup of $G$ containing $M$ as a Levi subgroup, one forms the induced representation $\mathcal V = \operatorname{Ind}_P^G \pi$ of $G$ and, wishing to embed $\mathcal V$ into $L^2(\Gamma \backslash G)$, one associates to each $\varphi_{\pi} \in \mathcal V$ the Eisenstein series
$$E(\varphi_{\pi},g) = \sum\limits_{\gamma \in \Gamma \cap P \backslash \Gamma \cap G} \varphi_{\pi}(\gamma g). \tag{1}$$
There is a bit more to it, as one needs to take continuous sums (integrals) of such Eisenstein series $E(\varphi_{\pi_s},g)$ for various unramified twists $\pi_s$ of $\pi$, and analytically continue $E(\varphi_{\pi_s},g)$ so that the formula (1) no longer makes sense.
These perspectives can be connected by associating modular forms with automorphic forms on $\operatorname{SL}_2(\mathbb Z) \backslash \operatorname{SL}_2(\mathbb R)$.
Did these two points of view on Eisenstein series develop independently? Who were the first people to connect them?