Riemann, fluid dynamics, and critical lines

Question

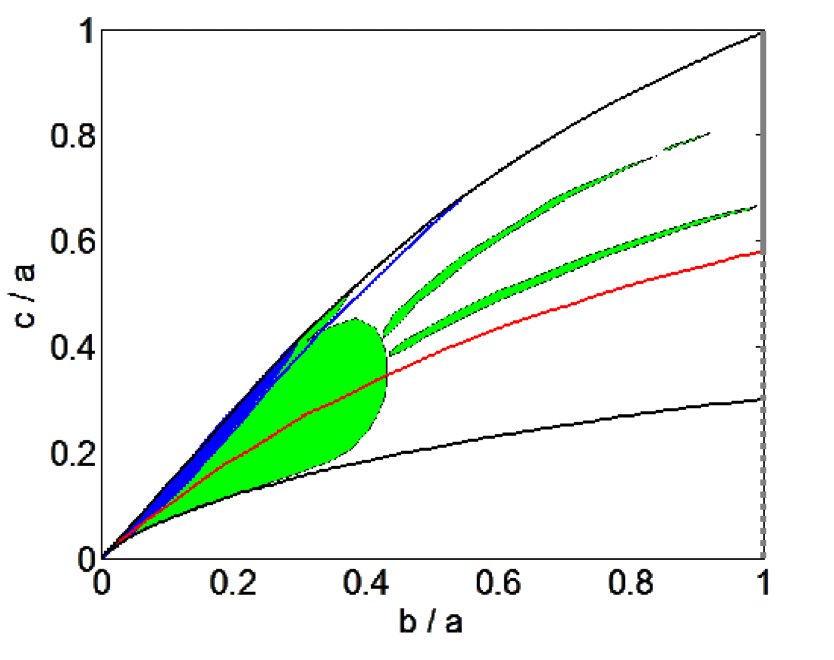
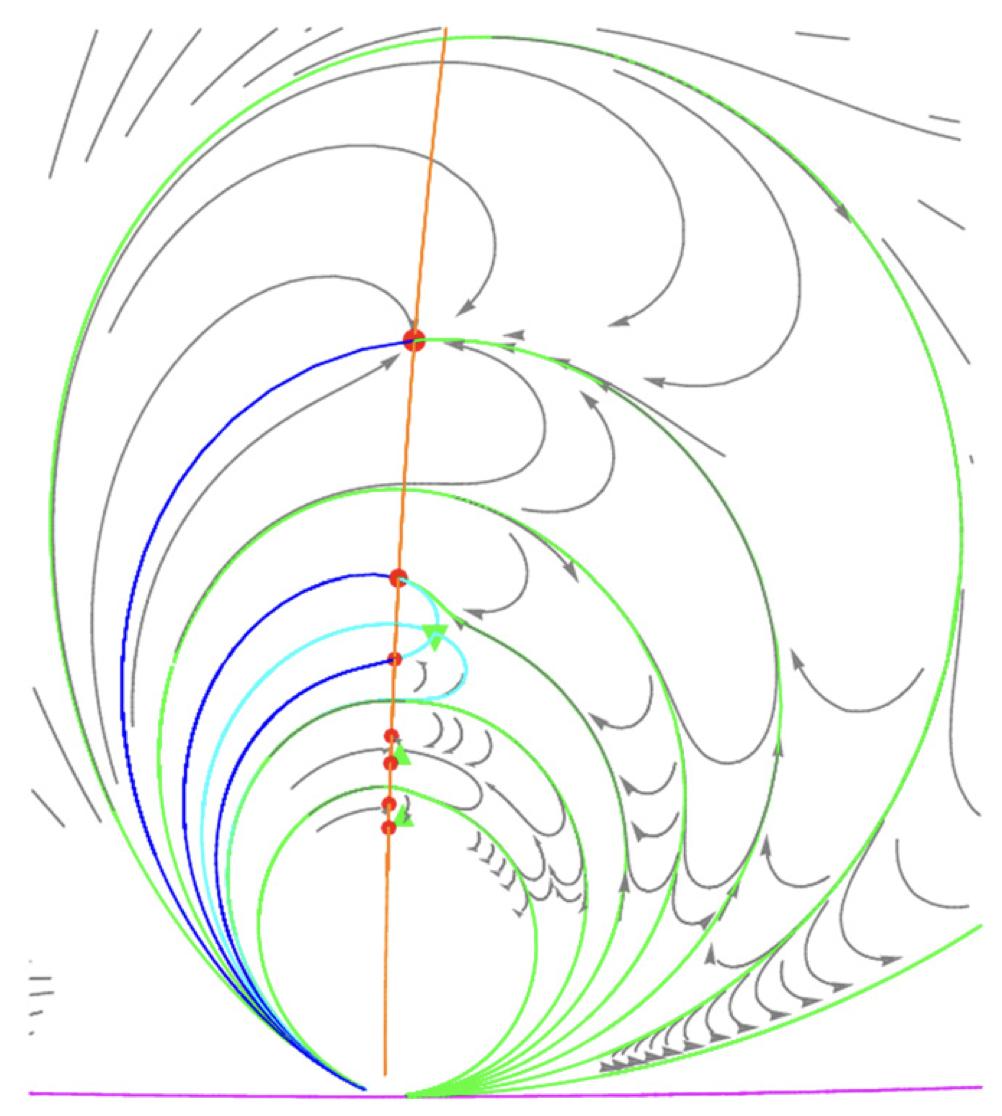
Marcus du Sautoy, in the section Riemann's Final Twist (pp. 278-80) in his book The Music of the Primes, discusses a discovery of Jon Keating of a connection in Riemann's Nachlass between Riemann's simultaneous investigations on the hydrodynamics of a ball/ellipsoid of fluid and the non-trivial zeros of the Riemann zeta function--both involve critical lines on which important imaginary numbers are distributed.

Does anyone know of a reference which discusses more thoroughly the critical line appearing in the hydrodynamics problem? (I suppose it has something to do with Hermite functions.)

Excerpts from The Music of the Primes:

The physicists believe that the reason Riemann's zeros will be in a straight line is that they will turn out to be frequencies of some mathematical drum. A zero off the line would correspond to an imaginary frequency which was prohibited by the theory. It was not the first time that such an argument had been used to answer a problem. Keating, Berry and other physicists all learnt as students about a classical problem in hydrodynamics whose solution depends on similar reasoning. The problem concerns a spinning ball of fluid held together by the mutual gravitational interactions of the particles inside it. For example, a star is a ball of spinning gas kept together by its own gravity. The question is, what happens to the spinning ball of fluid if you give it a small kick? Will the fluid wobble briefly and remain intact, or will the small kick destroy the ball completely? The answer depends on showing why certain imaginary numbers lie in a straight line. If they do, the spinning ball of fluid will remain intact. The reason why these imaginary numbers do indeed line up is related very closely to the quantum physicists' ideas about proving the Riemann Hypothesis. Who discovered this solution? Who used the mathematics of vibrations to force these imaginary numbers onto a straight line? None other than Bernhard Riemann.

Before Keating set off for Gottingen, one of his colleagues in the mathematics department, Philip Drazin, recommended looking at the part of the Nachlass in which Riemann tackles the classical problem of hydrodynamics.

At the library in Gottingen, Keating ordered the two different parts of the Nachlass that he wanted to consult: one on Riemann's ideas about the zeros in his zeta landscape, and the second on his work on hydrodynamics. When only one pile of papers appeared from the vaults, Keating mentioned that he had asked to see two parts. Both 'parts' were on the same sheets of paper, the librarian told him. As Keating explored the pages, he found to his amazement that Riemann had been concocting his proof about rotating balls of fluid at the very same time that he'd been thinking about the points at sea level in his zeta landscape. The very method by which modern-day physicists were proposing to force Riemann's zeros to line up had been used by Riemann to answer the hydrodynamics problem.

There, in front of Keating on the same pieces of paper, were Riemann's thoughts on both problems.

Yet again, the Nachlass had revealed how far Riemann was ahead of his time. He could not have failed to recognise the significance of his solution of the problem in fluid dynamics. His method had shown why certain imaginary numbers that emerged from his analysis of the ball of fluid were all in a straight line. Yet at the same time, and on the same paper, he was trying to prove why the zeros in his zeta landscape all lay on a straight line. In the year following his discoveries about primes and hydrodynamics, he was recording his new ideas in the little black book which, infuriatingly, disappeared from the archives. With it have disappeared Riemann's thoughts on uniting these two themes from number theory and physics.

Answer 1

Q: Does anyone know of a reference which discusses more thoroughly the critical line appearing in Riemann's hydrodynamics problem?

A: A recent reference is Elliptical instability in hot Jupiter systems by Cébron et al. (2013). The stability analysis of Riemann was not quite correct, it turns out.

_{Stability diagram of ellipsoids with a (resp. b) the longest (resp. shortest) equatorial axis and c the polar axis. For perturbations that are linear in the spatial coordinates, Riemann obtained unstable ellipsoids between the solid blue line and the black solid uppermost line, but Chandrasekhar showed in 1965 that the correct unstable zone is the blue one. The green zone corresponds to unstable ellipsoids for quadratic perturbations.}

---

For a hydrodynamic approach to the Riemann zeta function (unrelated to the above), see The Riemann hypothesis illuminated by the Newton flow of $\zeta$ by Neuberger, Feilers, Maier, and Schleich.

We analyze the Newton flow of the Riemann zeta function $\zeta$ and rederive in an elementary way the Riemann-von Mangoldt estimate of the number of non-trivial zeros below a given imaginary part. The representation of the flow on the Riemann sphere highlights the importance of the North pole as the starting and turning point of the separatrices, that is of the continental divides of the Newton flow. We argue that the resulting patterns may lead to deeper insight into the Riemann hypothesis. For this purpose we also compare and contrast the Newton flow of $\zeta$ with that of a function which in many ways is similar to $\zeta$, but violates the Riemann hypothesis.

---

Update, 9 June 2022: professor Keating's answer to my email inquiry

It is now many years since I saw the papers in the Riemann Nachlass and since I had this conversation, so I cannot be certain what I said, but essentially Riemann considered the stability of a rotating gas of particles held together by gravity. He computed the linear stability in the usual way, by considering small perturbations and computing the frequencies. Complex frequencies correspond to unstable modes (as you know) and real frequencies to stable vibrations. He showed that all of the frequencies are real by demonstrating that they are the eigenvalues of a self-adjoint operator (eg as one shows that the zeros of Bessel functions are real). So the critical line in this case is the real line (of frequency).

Experts tell me that Riemann made some mistakes in the calculations, but I have not looked into this carefully. Chandrasekhar wrote a book about the problem.

Juan Marin at Harvard has been going through the Nachlass carefully. He wrote to me that

"It also includes a version of Riemann's letter to Betti on how to find the attraction due to any homogeneous right ellipsoidal cylinder, including why "for the roots of the given equation F = 0 ... the singularities of the integrand are all real."

Indeed in a letter to Prym, Betti writes that Riemann was working on number theory on his last days. I found evidence in another notebook suggesting he worked specifically on the twin prime conjecture while finishing his article on the "hydraulics" of sound vibrations and the anatomy of the ear."

So it seems that Riemann worked on vibrations and number theory simultaneously on several occasions.

Jon Keating