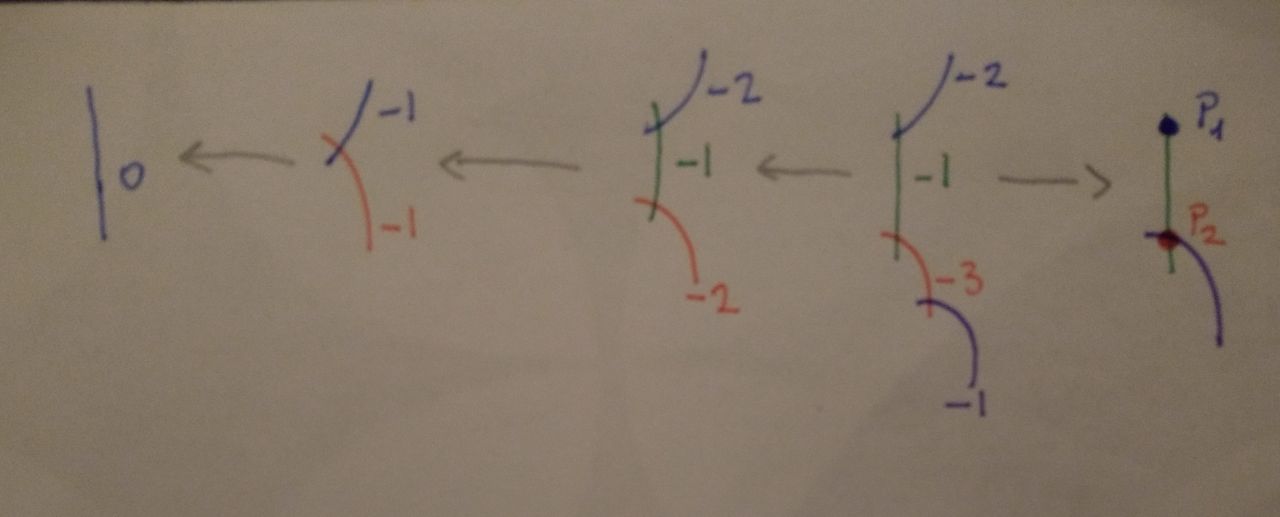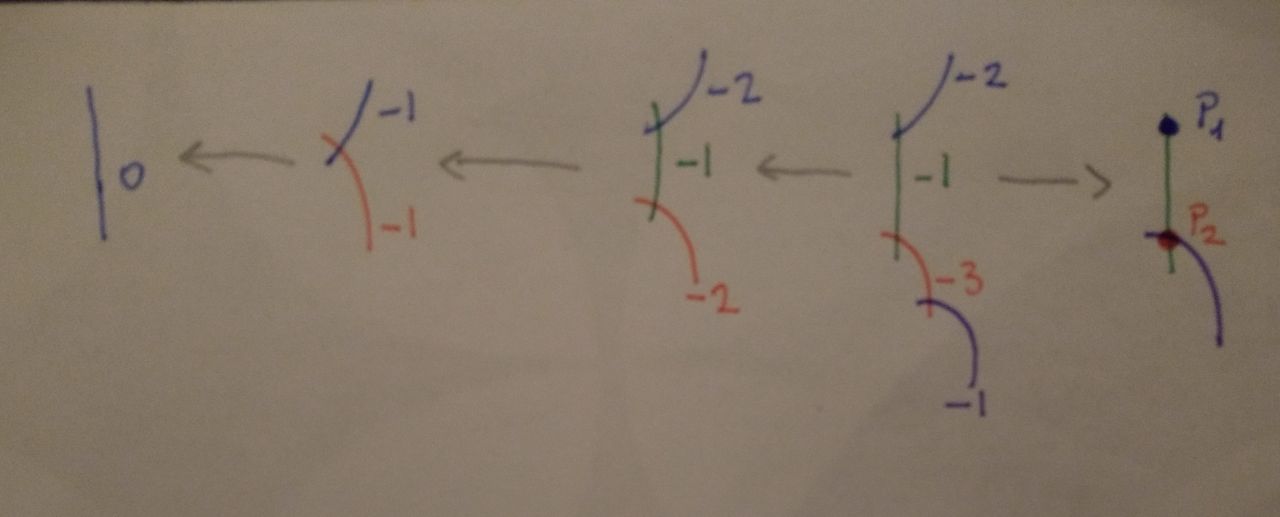Let $S$ be a projective surface with a morphism $S\rightarrow\mathbb{P}^1$ whose fibers are either smooth $\mathbb{P}^1$'s or the union of two smooth $\mathbb{P}^1$'s intersecting in a point.
Question: Is it true that $S$ is either smooth or if it is singular then its singularities are rational double points?
The answer is no, as shown by the following example.
Start from a Hirzebruch surface $\mathbb{F}_n$ and blow-up the same fibre three times as in the picture.
Then, by Artin's contractibility criterion, you can contract the $(-2)$-curve and the $(-3)$ curve to a singular projective surface $X$, endowed with a morphism $X \to \mathbb{P}^1$ whose general fibre is $\mathbb{P}^1$ and having a unique singular fibre, which is the union of two smooth $\mathbb{P}^1$ intersecting at one point.
The singularities of $X$ (which lie on this fibre) are an ordinary node and a $1/3(1, \, 1)$-singularity. The latter, denoted by $P_2$ in the picture and coming from the contraction of the $(-3)$-curve, is not a rational double point.
