2
$\begingroup$

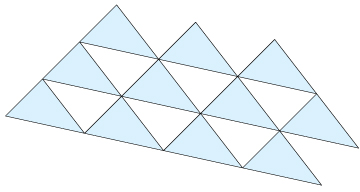
This post continues Thinnest rigid packings of the plane

A packing of the plane with copies of any shape is called rigid (or "stable") if every unit is fixed by its neighbors, i.e., no unit can be translated without disturbing others in the packing. Let us consider 'constrained rigid packs' - a rigid pack of identical units with the additional constraint that any 2 given units can touch each other at most at one point (we allow both corner to corner or corner to edge contacts).

  • Given a general triangle $T$ of unit area, how does one find a constrained rigid packing with copies of $T$ such that packing density is maximized (minimized) - ie. the highest(lowest) fraction of the plane is covered? Specifically, for any $T$, can one approach a perfect packing arbitrarily closely with such a constrained rigid pack?

  • Which specific triangle gives maximum(minimum) density for constrained rigid pack?

One can ask same questions in higher dimensions (in 3D, contact between units can be at a point or along a line segment or both) and in 2D with triangle replaced by other convex polygonal shapes.

Further question: What if rigidity is defined as "a unit cannot be translated or rotated without disturbing other units"?

Improve this question
$\endgroup$
3
  • $\begingroup$ Are your conditions equivalent to: A tiling where each $T$ touches others at its three corners, and only at those three corners? $\endgroup$
    – Joseph O'Rourke
    Commented Apr 29, 2022 at 11:04
  • $\begingroup$ Both corner to corner and corner to edge touches are allowed between units. Edited qn.. $\endgroup$
    – Nandakumar R
    Commented Apr 29, 2022 at 12:22
  • $\begingroup$ Ah, I see. My misreading. Thanks for clarifying. $\endgroup$
    – Joseph O'Rourke
    Commented Apr 29, 2022 at 13:09

1 Answer 1

Reset to default
1
$\begingroup$

An observation. If you are aiming for a minimal tiling, you could always achieve $\frac{1}{2}$ this way:

TilingHoles

Improve this answer
$\endgroup$
6
  • $\begingroup$ Yes. But I am not sure if that is the lowest density constrained pack. Thanks. $\endgroup$
    – Nandakumar R
    Commented Apr 29, 2022 at 17:07
  • $\begingroup$ Yes. At least it is a target to beat. $\endgroup$
    – Joseph O'Rourke
    Commented Apr 29, 2022 at 17:39
  • $\begingroup$ Considering the above pictured arrangement with equilateral triangle units, it appears: with the centroid of one unit as center, we can rotate that unit a little bit without disturbing other units - and that unit comes loose from the arrangement. So, it seems this way cannot achieve a rigid pack with 1/2 as packing fraction - at least with equilateral triangles. $\endgroup$
    – Nandakumar R
    Commented Apr 30, 2022 at 6:39
  • $\begingroup$ Note you specified translation: "no unit can be translated without disturbing others." It is a different problem if the arrangement must also be rigid w.r.t. rotations. $\endgroup$
    – Joseph O'Rourke
    Commented Apr 30, 2022 at 10:30
  • $\begingroup$ Thanks for pointing this out. Added that bit to the question. $\endgroup$
    – Nandakumar R
    Commented Apr 30, 2022 at 12:53

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .