7
$\begingroup$

Two students in my class asked and answered what might be a novel question. It is well known that the cube has exactly $11$ edge-unfoldings (or "nets"), as shown below:

   

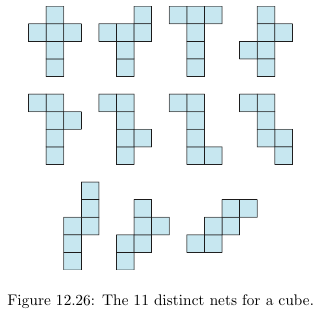
    (Image from this MO posting.)

It is also well known that each of the eleven can tile the plane, i.e., form a monohedral tiling. The students, Elsa Bieger and Heather Robertson, asked: Can the $11$ unfoldings be edge-to-edge glued together, each used exactly once, to form a supertile that can then tile the plane monohedrally? They found four supertiles:

   Supertiles

and here's how each supertile fits together with copies of itself:

   Monohedral

So in the spirt of the earlier MO question, Which unfoldings of the hypercube tile 3-space, which led to Moritz Firsching showing that all hypercube unfoldings can (individually) tile space, it is natural to ask:

Q. Can the $261$ unfoldings of the hypercube be face-to-face glued together, each used exactly once, to form a supertile that can then tile $3$-space monohedrally?

Improve this question
$\endgroup$
1
  • 1
    $\begingroup$ Note that all four supertiles satisfy the Conway criterion for tiling the plane, which prompts the obvious question as to whether there's a version of the criterion that applies in three dimensions; I feel like answering that question is a good (if hardly necessary) step towards answering this one. $\endgroup$
    – Steven Stadnicki
    Commented Apr 12, 2022 at 0:14

0

Reset to default

You must log in to answer this question.