Rate of decrease of the Fourier transform of standard mollifiers

Question

What is the the rate of decrease of $|\widehat{f_p}(t)|$ (as $t\to\infty$), where $p\in(0,\infty)$, $$\widehat{f_p}(t):=\int_{\mathbb R} e^{itx}f_p(x)\,dx,$$ and $$f_p(x):=e^{-1/(1-x^2/p)^p}1(|x|<\sqrt p),$$ so that $f_p\in C^\infty$, $f_p'(0)=0$, and $f_p''(0)=-2/e$ for all $p$.

Here are the graphs $\{(x,f_p(x))\colon|x|<\sqrt p\}$ for $p=1$ (red), $p=2$ (green), and $p=3$ (blue):

The decrease seems to be very fast, almost to $0$ in a finite time $t>0$. Below are parts of the graphs of the functions $\ln\ln\dfrac1{|\widehat{f_p}|}$ for $p=1$ (red), $p=2$ (green), and $p=3$ (blue):

This seems to almost contradict Hardy's uncertainty principle -- which implies, as noted in Dmitry Krachun's comment, that $\widehat{f_p}(t)$ cannot decay faster than $e^{-ct^2}$ for any $c>0$.

Answer 1

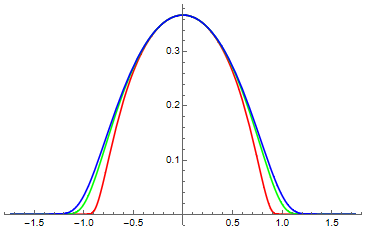
So, morally, the only real way to bound the decay of $\hat{f}$ is to obtain some bounds on the derivatives of $f$. Let me do a little dilation and consider $f(x) = \exp(-1/(1-x)^p - 1/(1+x)^p)$, it does a well-known thing on the Fourier side and doesn't affect general decay properties. And fortunately for us, bounds for the derivatives of this function were written out by Arie Israel in this beautiful paper (I think it's a third time I've given this reference to someone, see also this MO question for related matters). Specifically, Lemma 2 of his paper says that

$$|f^{(k)}(x)| \le (16p)^k k^{(1+1/p)k}.$$ (he considers the case $p\in \mathbb{N}$ but his methods should work for any $p > 0$).

We have $$|\hat{f}(\xi)| \le 2\frac{||f^{(k)}||_{L^\infty}}{|\xi|^k}.$$ Factor of $2$ here is because $\text{supp} f = [-1, 1]$ has length $2$ and there also might be some powers of $2\pi$ thrown here and there depending on the normalization of the Fourier transform. Neither of those will affect our argument of course.

Combining this with the above estimate we get

$$|\hat{f}(\xi)| \le 2 \left(\frac{16p k^{(1+1/p)}}{|\xi|}\right)^k.$$

We want to choose $k\in \mathbb{N}$ so that the number in the brackets is some constant, say it's at most $\frac{1}{2}$ and at least $\frac{1}{4}$ (this can always be done for $|\xi|$ big enough). This gives us $$|\hat{f}(\xi)| \le 2^{1-k}.$$ On the other hand we have $$k \ge \left(\frac{|\xi|}{64p}\right)^{p/(p+1)},$$ which gives us the desired decay in terms of $|\xi|$ of the form $$|\hat{f}(\xi)|\le A\exp(-B|\xi|^{p/(p+1)})$$ for some $A, B>0$.

Note that $\hat{f}$ can't have exponential decay because otherwise $f$ would be analytic in a strip and thus not compactly supported; one can actuallly deduce even more from the general results, see Denjoy-Carleman Theorem and Beurling-Malliavin Theorem in my answer to the above MO question. But here I think it is probable that the above decay is the correct one up to the values of $A$ and $B$: indeed, if $\hat{f}$ decays very fast then we can obtain better pointwise bounds on the derivatives of $f$ and the Israel's bounds are probably sharp, although I'm not sure how to prove it.