After checking the details, here is a statement that seems to answer your question:
Fact: For every free action of $F_n$ on a real tree $T$ and for all non-commensurated elements $g,h \in F_n$, the inequality $$\|h^kg^k\|_T > \frac{1}{2} \|hg\|_T$$ holds for every $k \geq 2$.
This is an immediate consequence of the following observation:
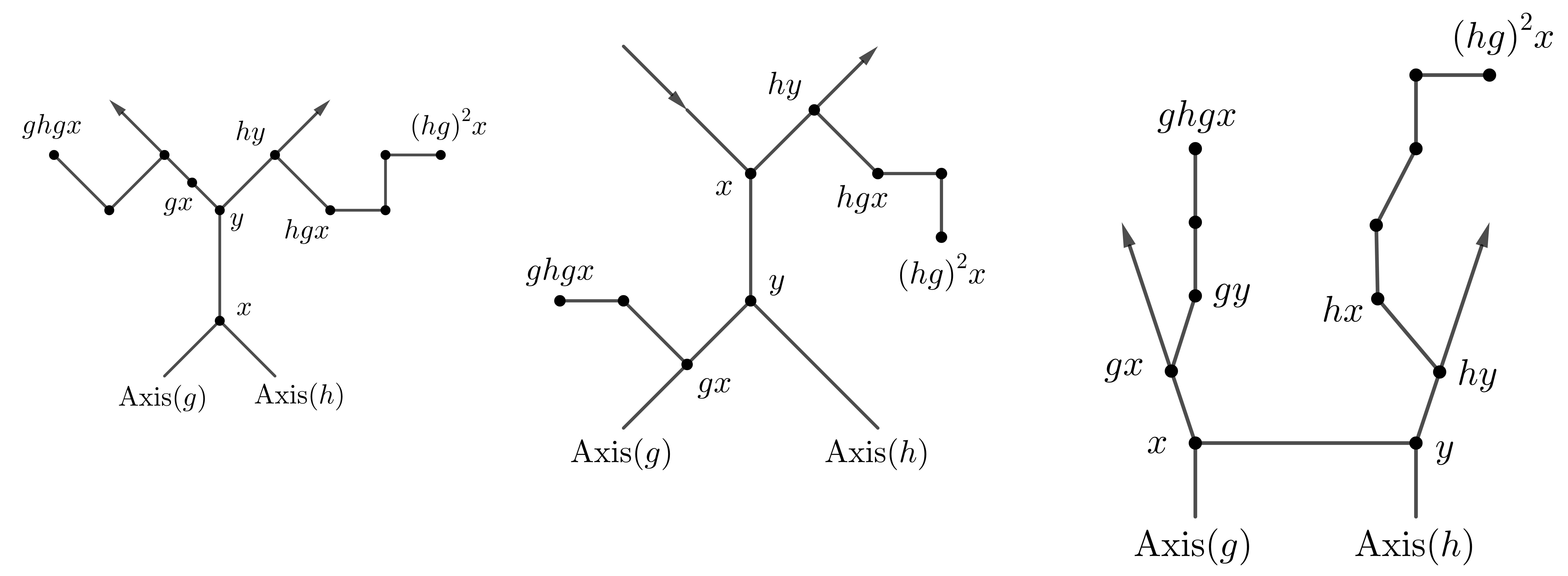
Lemma: Let $T$ be a real tree and $g,h \in \mathrm{Isom}(T)$ two independent loxodromic isometries. Assume that $$\mathrm{diam}(\mathrm{Axis}(g) \cap \mathrm{Axis}(h)) < \|g\|,\|h\|.$$ Then $hg$ is also loxodromic and its translation length is
- $\|h\|+ \|g\|$ if $\mathrm{diam}(\mathrm{Axis}(g) \cap \mathrm{Axis}(h))>0$ and if the translation directions of $g,h$ agree on the intersection $\mathrm{Axis}(g) \cap \mathrm{Axis}(h)$;
- $\|h\|+\|g\| - 2 \cdot \mathrm{diam}(\mathrm{Axis}(g) \cap \mathrm{Axis}(h))$ if $\mathrm{diam}(\mathrm{Axis}(g) \cap \mathrm{Axis}(h))>0$ and if the translation directions of $g,h$ disagree on the intersection $\mathrm{Axis}(g) \cap \mathrm{Axis}(h)$;
- $\|hg\|= \|h\|+\|g\| + 2 \cdot d(\mathrm{Axis}(h), \mathrm{Axis}(g))$ if $\mathrm{diam}(\mathrm{Axis}(g) \cap \mathrm{Axis}(h))=0$.
The proof is straightforward: it suffices to find a point $x \in T$ such that $hgx$ lies on $[x,(hg)^2x]$ and to compute the distance between $x$ and $hgx$. The different cases are illustrated by the following figures.