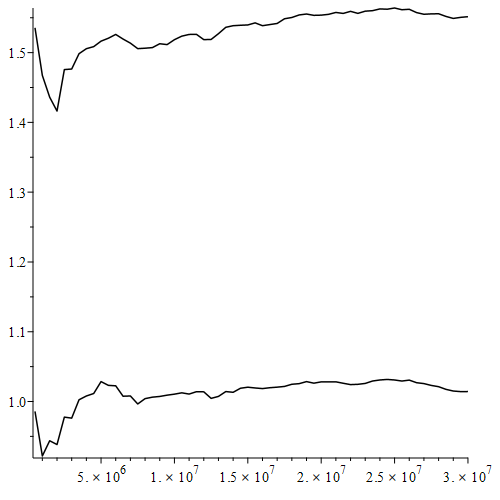
This question generalizes Symmetry in Hardy-Littlewood k-tuple conjecture. Say two prime constellations are equivalent up to permutation if they consist of the same multiset of prime gaps. One can order such constellations lexicographically according to the considered prime gaps, a smaller prime gap playing a role analogous to a letter occurring earlier in the alphabet.
That way, one can uniquely define a permutation $\sigma$ such that the action of $\sigma$ on the multiset of prime gaps contained in the constellation $C$ gives rise to the constellation $C_{\sigma}$.
My question is: do any two prime constellations equivalent up to permutation have the same distribution? Can a link with Chebotarev's theorem be established, viewing the set of prime constellations equivalent to $C$ up to permutation as an analogue of a conjugacy class?
I'm interested in both conditional and unconditional results or heuristics.