Solution of parabolic partial differential equation using singular perturbation method

Question

Consider the following parabolic partial differential equation (PDE)

\begin{align} \label{eq:42} \left(\cos\psi \frac{\partial}{\partial r} + \frac{\gamma}{r} \sin\psi \frac{\partial}{\partial \psi} + \epsilon \frac{\partial^{2}}{\partial \psi^{2}} \right)u(r, \psi) = -1, \end{align}

where $u(r, \psi): [0,1]\times[0,2\pi] \to \mathbb{R}^+$ and $\epsilon,\gamma \in \mathbb{R}^+$ are constant parameters. The boundary conditions are Dirichlet $u(r, \psi)|_{r=1} = 0$ and periodic $u(r, \psi+2\pi) = u(r, \psi)$.

When $\epsilon\to 0$, the above second order PDE reduces to first order PDE, the boundary conditions generally cannot be fulfilled anymore, entailing singular perturbation method to handle the equation.

How to solve the above the equation with $\epsilon\to 0$ using singular perturbation method?

I mainly refer to the book written by J. Kevorkian and J.D. Cole titled ‘‘Multiple Scale and Singular Perturbation Methods’’. Singular perturbation methods for elliptic and parabolic PDEs are introduced there. However, I still have no clue of how to handle the above parabolic PDE. In case it helps, the (projected) characteristic curve of the reduced PDE when $\epsilon=0$ is

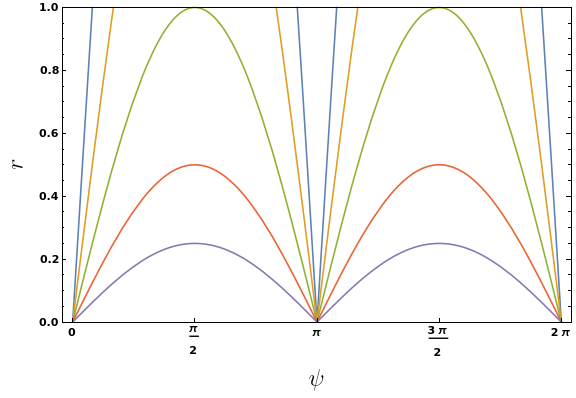
\begin{align} \label{eq:62} r = |\sin\psi|^{\frac{1}{\gamma}} C, \end{align}

where $C$ is constant, which can be visualized in the following plot ($\gamma=1$).

My background is theoretical physics. Please let me know if there is something mathematically inaccurate in the above problem formulation. Any suggestion or recommendation of references would be greatly appreciated. Thanks.

Answer 1

This is not an attempt at an answer, just some comments to get you started. But it is going to be too long for a comment. The first order of business should be to solve the reduced problem for $\epsilon=0$. There is a difference between the region above the green curves and the region below. For the region above, you have a characteristic connecting to your initial condition at r=1, and you can use that to get a solution. Below the green curve, you do not connect to r=1, and instead the condition you impose should be some condition of regularity at r=0. Patching this together will probably leave you with some singularity along the green curve, leading to a transition layer which you have to resolve with an appropriate rescaling. Following through with all this should give you a formal approximation to a solution. Depending on your philosophical outlook, you will either celebrate victory at this point or feel challenged to launch an effort to derive a dozen or (probably) more pages of estimates.

Answer 2

I hope this (partial) answer is still relevant. In the first place, I would not classify this as a parabolic problem, but as an elliptic problem. You can see this intuitively, as your problem is prescribed by bc's, not by an initial condition that you let evolve (as you would expect for a parabolic problem). Now you could expect there to be a boundary layer somewhere, probably at a part of the edge of your circular domain, a numerical investigation could help you there. In other words, the $\varepsilon=0$ approximation is only a good approximation away from the boundary layer. There should be plenty of modern books that explain boundary layers in elliptic pde's, but one of the original sources is $\S$7.2 in Eckhaus' book Asymptotic analysis of singular perturbations.