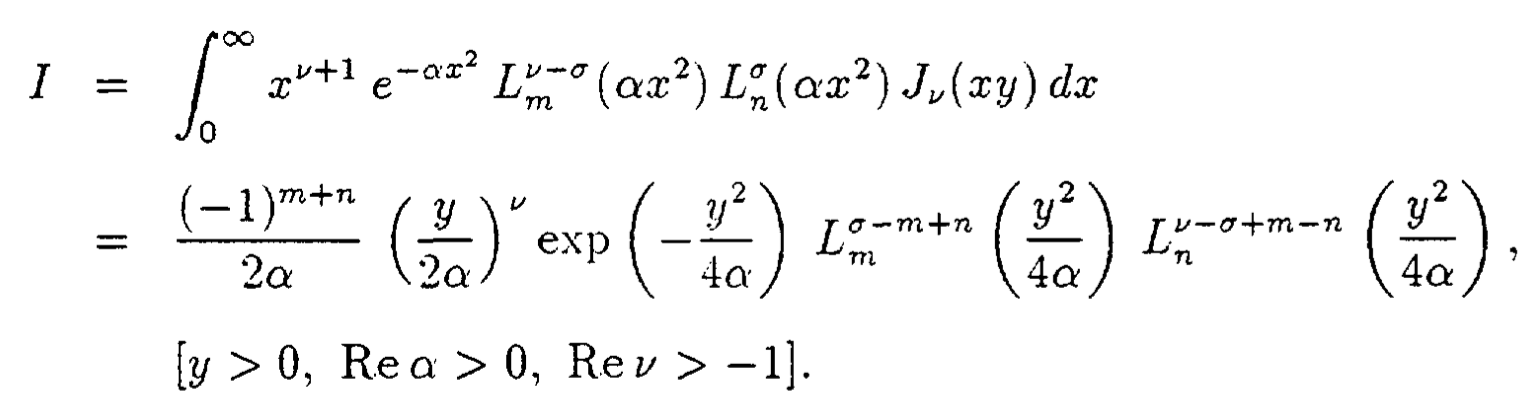1
$\begingroup$

When we think about the Fourier transform in two dimensional polar coordinates, the Hankel transform is the transformation with respect to the polar diameter. Now I have a question, why is the following expression invariant for the Hankel transform? And How to prove this equation?

$\exp \left(-\frac{1}{2} x\right) x^{m / 2} L_{p}^{m}(x)=\frac{1}{2}(-1)^{p} \int_{0}^{+\infty} \mathrm{d} y \exp \left(-\frac{1}{2} y\right) J_{m}(\sqrt{x y}) y^{m / 2} L_{p}^{m}(y)$

$L_{p}^{m}(y)$ is Laguerre function. m, p is order;

$J_{m}(\sqrt{x y})$ is Bessel function with order of m.

L. Yu et al., The Laguerre-Gaussian series representation of two-dimensional fractional Fourier transform. Journal of Physics A: Mathematical and General 31, 9353-9357 (1998).

Improve this question
$\endgroup$
1
  • $\begingroup$ The first part is trivial (why is). The Hankel transform is its own inverse. Just apply the Hankel transform and you are done. I agree you should ask on math.se $\endgroup$
    – username
    Commented Jun 23, 2021 at 12:33

1 Answer 1

Reset to default
0
$\begingroup$

Q: How to prove this equation?

A: An explicit proof, of a more general identity, is in On a Hankel Transform Integral containing an Exponential Function and Two Laguerre Polynomials. See Equation (5), and take $\sigma=0$, $m=n$.

enter image description here

Another special case ($m=n$, $\nu=2\sigma$) was discussed at MSE, with a reference to a 1936 paper by Watson, An Integral Equation for the Square of a Laguerre Polynomial

Improve this answer
$\endgroup$

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .